Unraveling the Formula for Calculating Variance
August 14, 2023 by JoyAnswer.org, Category : Statistics
What is the computational formula for variance? Understand the computational formula used to calculate variance in statistics. Explore the mathematical steps involved in determining variance and its significance in analyzing data.
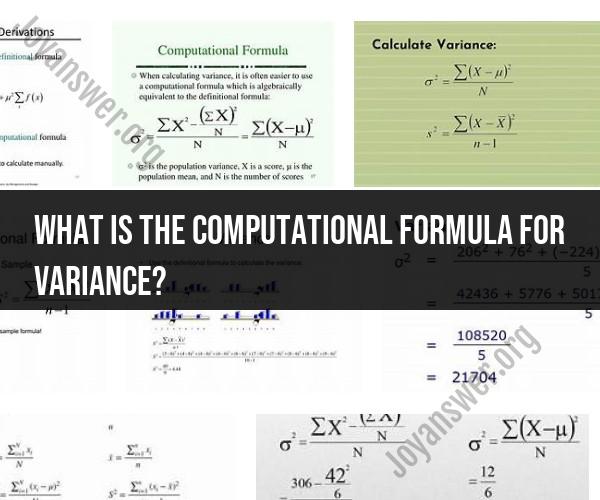
What is the computational formula for variance?
Variance is a fundamental concept in statistics that measures the spread or dispersion of a set of data points around the mean. It provides insight into the variability within a dataset, helping analysts and researchers better understand the distribution of values. Let's dive into the formula for calculating variance:
The Formula:
The formula for calculating the variance of a dataset involves several key steps:
- Calculate the mean (average) of the data points.
- For each data point, subtract the mean from the value and square the result.
- Sum up the squared differences from step 2.
- Divide the sum from step 3 by the total number of data points (sample size).
The formula can be expressed mathematically as:
Variance = Σ(xi - μ)^2 / n
Where:
- Σ represents the sum symbol.
- xi represents each individual data point.
- μ represents the mean of the data points.
- n represents the total number of data points.
Interpretation:
The variance measures how much each data point deviates from the mean. A larger variance indicates greater variability, while a smaller variance suggests less dispersion. Variance is always a non-negative value, as it involves squaring the differences.
Application:
Variance is used in various fields, including finance, economics, science, and engineering. It is a crucial component in calculating standard deviation, another measure of data dispersion. In data analysis, understanding variance helps identify trends, patterns, and outliers in datasets.
Conclusion:
The formula for calculating variance provides valuable insights into the distribution of data points and their deviations from the mean. Whether you're conducting research, making financial decisions, or analyzing scientific data, a clear understanding of variance enhances your ability to interpret and draw meaningful conclusions from datasets.
