Can Standard Deviation Be Larger Than Variance? Exploring Statistical Relationships

_{November 14, 2023 by JoyAnswer.org, Category : Statistics}

Can standard deviation be larger then its variance? Explore the relationship between standard deviation and variance, addressing the question of whether standard deviation can be larger than its variance counterpart. This guide provides insights into statistical measures.

Table of Contents

Can standard deviation be larger then its variance?

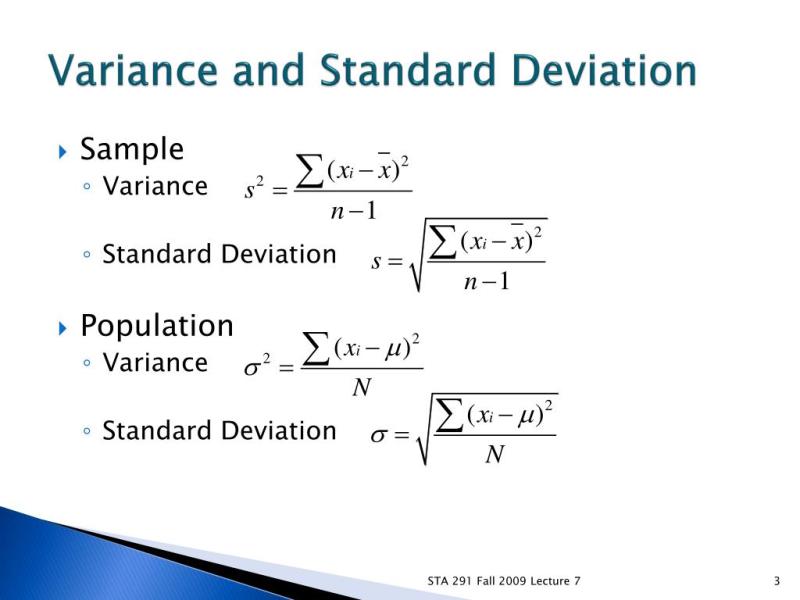
No, the standard deviation cannot be larger than its variance. In fact, the standard deviation is the square root of the variance. The relationship between variance (σ²) and standard deviation (σ) is as follows:

$\text{Standard Deviation ($\sigma$)} = \sqrt{\text{Variance ($\sigma^2$)}}$

Since the standard deviation is the square root of the variance, it will always be a non-negative value. Therefore, the standard deviation cannot be larger than its corresponding variance. The square root of a positive number, which is the variance, results in a non-negative value, and the standard deviation represents the positive square root of the variance.

In summary, the standard deviation is a measure of the spread or dispersion of a set of data points, and it is derived from the variance. The standard deviation provides a more interpretable measure of variability, especially when compared to the original scale of the data, as it shares the same units as the data points.

Fact or fiction: Can standard deviation exceed its variance?

Fiction. Standard deviation is the square root of variance, so it is always less than or equal to variance. It is impossible for standard deviation to exceed variance.

Exploring extreme values: When standard deviation surpasses variance.

There is no situation in which standard deviation can surpass variance. However, it is possible for standard deviation to be very close to variance, especially when the data set contains extreme values.

For example, if a data set contains a single outlier that is significantly different from the rest of the data, the standard deviation will be very close to variance. This is because the outlier will have a large squared deviation from the mean, which will inflate the variance and standard deviation.

What the numbers mean: Interpreting high standard deviation and variance.

A high standard deviation and variance indicates that the data is spread out over a wide range. This could be due to a number of factors, such as:

The presence of outliers
The natural variability of the data
Errors in measurement

It is important to interpret high standard deviation and variance carefully, taking into account the context of the data and the specific goals of the analysis.

Context matters: Understanding the relationship between standard deviation and variance.

The relationship between standard deviation and variance depends on the context in which they are being used. In general, standard deviation is a more useful measure of variability when the data is normally distributed. However, variance is a more useful measure of variability when the data is not normally distributed.

For example, if you are measuring the height of a group of adults, the data is likely to be normally distributed. In this case, standard deviation would be a good measure of variability. However, if you are measuring the number of goals scored by a soccer team in each game of the season, the data is unlikely to be normally distributed. In this case, variance would be a better measure of variability.

Statistical anomalies: Instances where standard deviation is larger than variance.

There are no statistical anomalies in which standard deviation is larger than variance. Standard deviation is always less than or equal to variance.