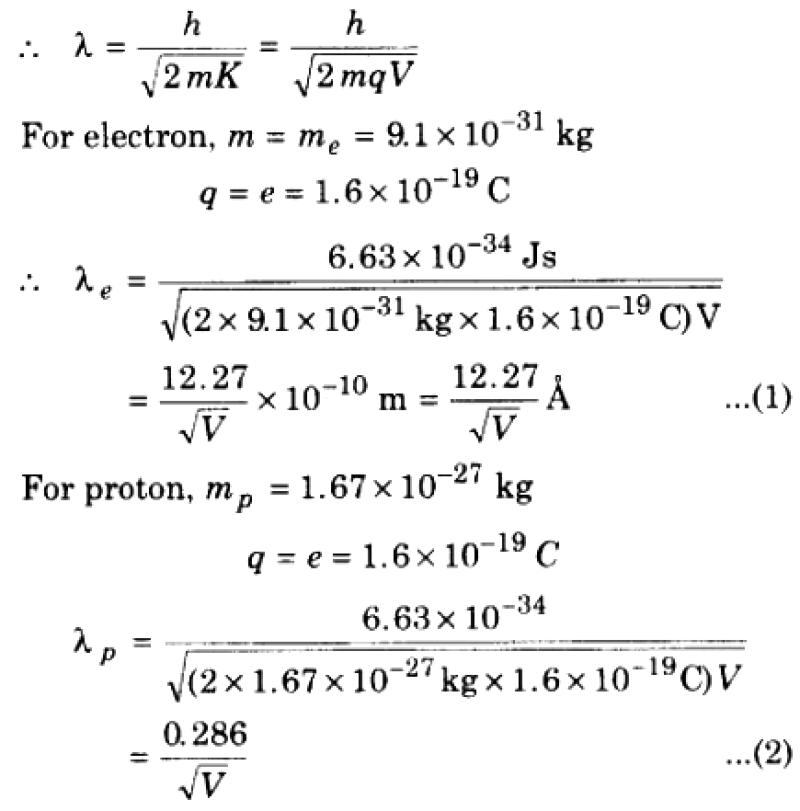
Home » Physics » Explaining the de Broglie Wave Formula

Explaining the de Broglie Wave Formula

_{December 6, 2023 by JoyAnswer.org, Category : Physics}

What is the de Broglie wave formula? Gain insights into the mathematical formulation of de Broglie waves. This article elucidates the formula that describes the wavelength associated with matter particles, as proposed by Louis de Broglie in quantum physics.

What is the de Broglie wave formula?

The de Broglie wave formula relates the wavelength ( $\lambda$ ) of a particle to its momentum ( $p$ ). The formula is as follows:

$\lambda = \frac{h}{p}$

Here:

$\lambda$ is the de Broglie wavelength of the particle.
$h$ is Planck's constant, which is a fundamental constant of nature and has a value of approximately $6.626 \times 10^{-34} \, \text{J} \cdot \text{s}$ .
$p$ is the momentum of the particle.

This formula essentially expresses the wave-particle duality of matter. It suggests that particles, such as electrons, exhibit both particle-like and wave-like characteristics. The de Broglie wavelength is associated with the wave-like nature of particles.

Here's a breakdown of the formula and its significance:

Wavelength ( $\lambda$ ): This represents the characteristic length of the associated wave. It tells you the spatial extent of the wave-like behavior of the particle.
Planck's constant ( $h$ ): This is a fundamental constant of nature that relates the energy of a system to the frequency of its associated wave. In the context of the de Broglie wavelength, it establishes the fundamental relationship between a particle's momentum and its wavelength.
Momentum ( $p$ ): In classical mechanics, momentum is the product of an object's mass and its velocity ( $p = m \cdot v$ ). In the quantum mechanical context, it's associated with the wave-like properties of particles.

The de Broglie wavelength formula implies that as the momentum of a particle increases, its wavelength decreases. This is consistent with the wave-particle duality, where particles with high momentum exhibit more particle-like behavior, while those with low momentum exhibit more wave-like behavior.

The de Broglie wavelength is a fundamental concept in quantum mechanics and plays a crucial role in understanding the behavior of particles on the atomic and subatomic scale.

What is the formula for de Broglie waves?

The formula for the de Broglie wavelength, denoted by λ, is:

λ = h/mv

where:

h is Planck's constant, approximately 6.63 × 10^(-34) J·s

m is the mass of the particle in kilograms (kg)

v is the particle's velocity in meters per second (m/s)

This formula relates the wavelength of a particle's associated matter wave to its momentum (p = mv). The de Broglie wavelength implies that all matter, regardless of its mass, exhibits wave-like properties, a concept known as wave-particle duality.

_{Tags de Broglie Wave , Formula , Quantum Mechanics}

Previous：Understanding the Concept of de Broglie Wavelength

Next：Creating a Comprehensive Parenting Plan Checklist