Finding Angular Speed: Formulas and Methods

_{October 14, 2023 by JoyAnswer.org, Category : Physics}

How can you find angular speed? Discover various methods and formulas for calculating angular speed, a fundamental concept in physics. This article provides step-by-step guidance for determining angular speed.

Table of Contents

How can you find angular speed?

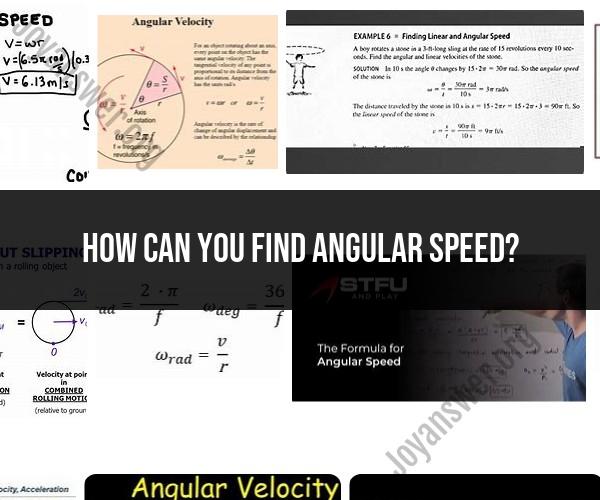
Angular speed, also known as angular velocity, is a measure of how quickly an object rotates around a fixed point or axis. It is typically measured in radians per second (rad/s) or degrees per second (°/s). To find angular speed, you can use the following formulas and methods:

Using Basic Formula:
- Angular Speed (ω) = Change in Angle (Δθ) / Change in Time (Δt)
- The change in angle is typically measured in radians (for rad/s) or degrees (for °/s).
Converting from Linear Speed:
- If you know the linear speed (v) of an object that is rotating in a circle of radius (r), you can find the angular speed using this formula:
  - Angular Speed (ω) = Linear Speed (v) / Radius (r)
- Make sure the linear speed and radius have consistent units (e.g., meters per second and meters) for the calculation.
Using Period (T):
- If you know the time it takes for one complete revolution (period, T) of the rotating object, you can find the angular speed using:
  - Angular Speed (ω) = 2π / T (in rad/s)
- If T is in seconds, the angular speed will be in rad/s.
Using Linear Velocity (v) and Circumference (C):
- If you know the linear velocity (v) and the circumference (C) of the circular path, you can find the angular speed:
  - Angular Speed (ω) = v / C
- The linear velocity is typically measured in meters per second, and the circumference is the distance around the circle.
Using Revolution Frequency (f):
- If you know the frequency (f) at which an object completes revolutions, you can find the angular speed using:
  - Angular Speed (ω) = 2πf (in rad/s)
- The frequency is measured in hertz (Hz).
Using Degrees:
- If you want to find the angular speed in degrees per second, you can use the formula:
  - Angular Speed (ω) = Change in Angle (Δθ) / Change in Time (Δt) in degrees per second
- Ensure that the change in angle is measured in degrees and the time in seconds.

It's important to use consistent units when applying these formulas. Additionally, angular speed can be positive for counterclockwise rotation and negative for clockwise rotation, depending on the chosen coordinate system.

To find angular speed, you typically need information about the change in angle, time, radius, linear speed, or linear velocity, depending on which formula is most appropriate for your specific situation.

Calculating Angular Speed in Circular Motion

Angular speed is the rate of change of angular position in circular motion. It is measured in radians per second (rad/s).

To calculate angular speed, you can use the following formula:

ω = θ/t

where:

ω is the angular speed in rad/s
θ is the angular displacement in radians
t is the time in seconds

For example, if an object rotates 360 degrees in 10 seconds, then its angular speed is:

ω = θ/t = 2π rad / 10 s = 0.2π rad/s

Differentiating Angular Speed from Angular Velocity

Angular speed is a scalar quantity, meaning that it has only a magnitude. Angular velocity, on the other hand, is a vector quantity, meaning that it has both a magnitude and a direction.

The direction of angular velocity is perpendicular to the plane of rotation. For example, the angular velocity of a spinning top would be pointing straight up.

Angular Speed Units and Conversions

The SI unit of angular speed is rad/s. However, there are other units of angular speed that are commonly used, such as degrees per second (deg/s) and revolutions per minute (rpm).

To convert between different units of angular speed, you can use the following conversion factors:

1 rad = 180/π deg
1 rpm = 2π/60 rad/s

For example, to convert 0.2π rad/s to rpm, you would use the following conversion factor:

0.2π rad/s * 60/(2π) = 6 rpm

Applications and Use Cases of Angular Speed

Angular speed is used in a variety of applications, including:

Engineering: Angular speed is used to design and analyze rotating machines, such as engines, turbines, and generators.
Physics: Angular speed is used to study the motion of objects in circular motion, such as planets and stars.
Sports: Angular speed is used to analyze the performance of athletes in sports such as baseball, golf, and tennis.

Angular Speed in Everyday Life and Physics

Angular speed is all around us in everyday life. For example, the Earth rotates on its axis at a rate of 0.00417 rad/s. This means that it takes the Earth 24 hours to complete one revolution.

Another example of angular speed is the fan in your computer. The fan rotates at a high speed to cool the components of your computer.

In physics, angular speed is used to study the motion of objects in circular motion. For example, the angular speed of a planet orbiting the Sun is determined by its distance from the Sun and its mass.

Angular speed is an important concept in physics and engineering. It is used to design and analyze rotating machines, to study the motion of objects in circular motion, and to explain a variety of phenomena in the natural world.