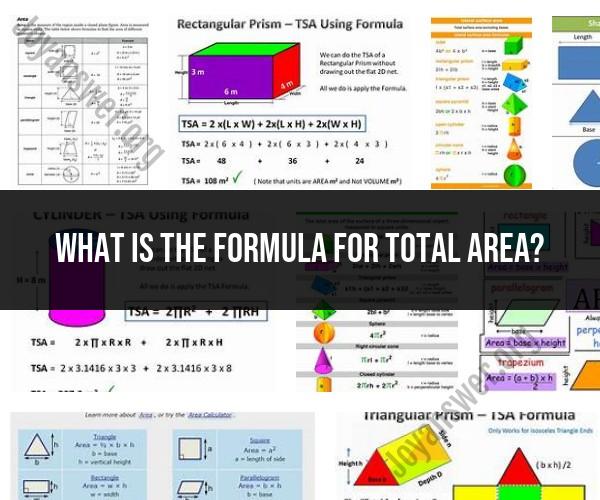
What is the formula for total area?
The formula for calculating the total area of a shape or object depends on the specific shape you are dealing with. Here are some common formulas for calculating the total area of various geometric shapes:
Rectangle:
- Total Area = Length × Width
Square:
- Total Area = Side Length × Side Length (or Area = Side^2)
Triangle:
- Total Area = (Base × Height) / 2
Circle:
- Total Area = π × (Radius^2), where π (pi) is approximately 3.14159
Trapezoid:
- Total Area = (1/2) × (Sum of the lengths of the parallel sides) × Height
Parallelogram:
- Total Area = Base × Height
Ellipse:
- Total Area = π × (Semi-Major Axis) × (Semi-Minor Axis)
Regular Polygon (e.g., Regular Hexagon, Regular Octagon):
- Total Area = (Perimeter × Apothem) / 2
Irregular Shapes:
- For irregular shapes, you may need to break them down into simpler shapes (e.g., triangles, rectangles) and calculate the areas of those individual parts separately. Then, add up the areas of the parts to find the total area.
Remember to use the appropriate formula for the specific shape you are dealing with. These formulas are applicable in two-dimensional space (2D) for flat shapes. If you're dealing with three-dimensional objects (3D), you would use volume formulas to find the total volume instead of total area.
Total Area Calculation: The Formula and Process for Comprehensive Measurement
The total area of a shape is the sum of the areas of all of its faces. To calculate the total area, you can use the following formula:
Total area = sum of the areas of all faces
For example, to calculate the total area of a cube, you would add up the areas of all six of its faces.
Total area of a cube = 6 * (side length)^2
To calculate the total area of a more complex shape, you may need to break it down into simpler shapes. For example, to calculate the total area of a cylinder, you would need to calculate the area of the top and bottom circles, as well as the area of the rectangular side.
Total area of a cylinder = 2 * (area of the base circle) + (area of the rectangular side)
Going Beyond Surface Area: How to Find the Total Area of Complex Shapes
To find the total area of complex shapes, you may need to use a variety of formulas. Here are some examples:
- Sphere: Total area = 4 * π * radius^2
- Cone: Total area = π * radius * (radius + slant height)
- Pyramid: Total area = lateral area + base area
- Prism: Total area = 2 * base area + lateral area
The lateral area of a prism or pyramid is the sum of the areas of all of its faces except for the base.
Total Area Formulas: Tools for Accurate Measurement in Various Contexts
Total area formulas can be used in a variety of contexts, such as:
- Construction: Architects and engineers use total area formulas to calculate the amount of materials needed to build structures.
- Manufacturing: Manufacturers use total area formulas to calculate the amount of material needed to produce products.
- Painting: Painters use total area formulas to estimate the amount of paint needed to paint a room or object.
- Gardening: Gardeners use total area formulas to calculate the amount of soil or mulch needed to cover a garden bed.
By understanding how to calculate total area, you can solve a variety of problems in different fields.
Here are some additional tips for calculating the total area of complex shapes:
- Break the shape down into simpler shapes. This will make it easier to calculate the area of each face.
- Use the appropriate formula for each face. Be sure to identify the type of face and then use the appropriate formula to calculate its area.
- Add up the areas of all the faces. Once you have calculated the area of each face, add them up to get the total area of the shape.
If you are unsure how to calculate the total area of a complex shape, you can consult a reference book or website.