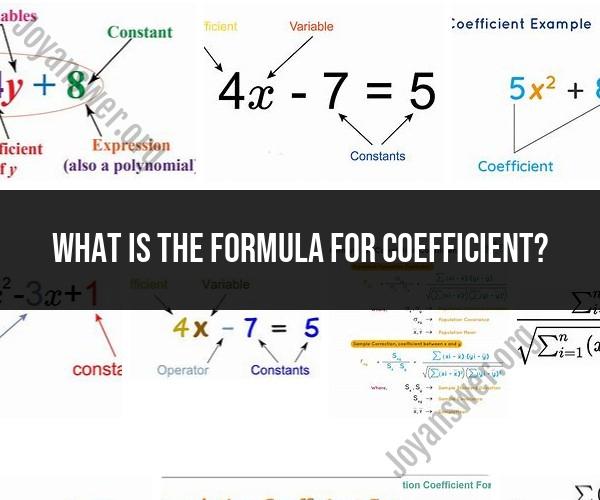
Coefficient Formula: Understanding the Mathematical Concept

_{October 20, 2023 by JoyAnswer.org, Category : Mathematics}

What is the formula for coefficient? Learn about the concept of a coefficient and its formula, highlighting its significance in various mathematical and scientific contexts.

Table of Contents

What is the formula for coefficient?

In mathematics, physics, and various other fields, the term "coefficient" is used in different contexts and has different formulas associated with it. The specific formula for a coefficient depends on the context in which it is used. Here are a few common contexts and their associated coefficient formulas:

Linear Equation Coefficient:
In a linear equation in the form "y = mx + b," where "m" is the slope of the line, "m" is the coefficient of the variable "x." The formula for the coefficient in this context is simply the value of "m."
Quadratic Equation Coefficients:
In a quadratic equation like "ax^2 + bx + c = 0," the coefficients are "a," "b," and "c." These coefficients are used to determine the roots or solutions of the quadratic equation, which can be found using the quadratic formula:
$x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$
Coefficient of Determination (R-squared):
In statistics, the coefficient of determination (often denoted as R-squared) is a measure of how well a regression model fits the data. The formula for R-squared is:
$R^2 = 1 - \frac{\text{Sum of Squares of Residuals}}{\text{Total Sum of Squares}}$
Binomial Coefficients:
In combinatorics, the binomial coefficient, denoted as "C(n, k)" or "n choose k," represents the number of ways to choose "k" items from a set of "n" distinct items without regard to order. The formula for binomial coefficients is:
$C(n, k) = \frac{n!}{k!(n-k)!}$
Fourier Coefficients:
In Fourier analysis, the Fourier coefficients represent the amplitude and phase of the sine and cosine terms in a Fourier series. The formulas for these coefficients depend on the specific function being analyzed and can involve integrals or summations.
Thermal Expansion Coefficient:
In physics and engineering, the coefficient of thermal expansion (α) represents how a material's dimensions change with temperature. The formula for thermal expansion is often material-specific and involves measuring the change in length, area, or volume as a function of temperature change.
Coefficient of Friction:
The coefficient of friction (μ) is a value that describes the relationship between the force of friction between two objects and the normal force pressing them together. The formula for frictional force is:
$F_f = \mu \cdot F_n$

These are just a few examples of coefficients in different mathematical and scientific contexts. The formula for a coefficient depends on the specific context and what it represents. It's important to understand the context in which you are working to use the correct formula.

The Formula for Calculating the Coefficient in Math and Science

The formula for calculating the coefficient in math and science varies depending on the context in which it is being used. However, a general formula can be expressed as follows:

Coefficient = (Constant value) / (Variable value)

For example, in the equation y = 2x + 5, the coefficient of x is 2. This is because the constant value in the equation is 5 and the variable value is x.

Types of Coefficients and Their Mathematical Representations

There are many different types of coefficients, each with its own mathematical representation. Some common types of coefficients include:

Linear coefficients: These coefficients represent the slope of a line in an equation. They are typically represented by the letter m.
Quadratic coefficients: These coefficients represent the leading coefficient, x-coefficient, and constant term in a quadratic equation. They are typically represented by the letters a, b, and c.
Binomial coefficients: These coefficients represent the coefficients in the binomial expansion of a binomial expression. They are typically represented by the letter n and the binomial coefficient symbol, nCk.

Practical Applications of Coefficients in Various Fields

Coefficients are used in a wide variety of fields, including math, science, engineering, and economics. Here are a few examples of practical applications of coefficients:

In math, coefficients are used to represent the coefficients of a polynomial, the slope of a line, and the y-intercept of a line.
In science, coefficients are used to represent the coefficients of friction, the coefficients of restitution, and the coefficients of thermal expansion.
In engineering, coefficients are used to represent the coefficients of drag, the coefficients of lift, and the coefficients of thrust.
In economics, coefficients are used to represent the coefficients of production, the coefficients of consumption, and the coefficients of investment.

Understanding Coefficients in Algebra and Calculus

In algebra, coefficients are used to represent the coefficients of a polynomial and the slope of a line. Coefficients can also be used to represent the constants in other algebraic expressions, such as the constant term in a quadratic equation.

In calculus, coefficients are used to represent the coefficients of a polynomial function and the slope of a tangent line. Coefficients can also be used to represent the constants in other calculus expressions, such as the constant term in a differential equation.

The Role of Coefficients in Equations and Formulas

Coefficients play an important role in equations and formulas. They can be used to represent the different components of a mathematical expression, such as the slope, y-intercept, and constant term in a linear equation. Coefficients can also be used to represent the different physical quantities that are involved in a physical phenomenon.

For example, in the equation F = ma, the coefficient of m is 1. This means that the force (F) acting on an object is directly proportional to its mass (m) and acceleration (a).

Coefficients are an essential part of mathematics and science. They allow us to represent and understand complex mathematical expressions and physical phenomena.