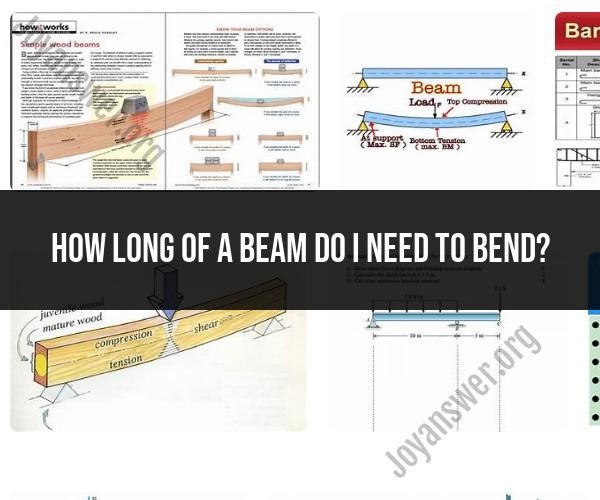
Determining Beam Length for Bending: Considerations

_{October 15, 2023 by JoyAnswer.org, Category : Engineering}

How long of a beam do I need to bend? Explore the factors and considerations for determining the necessary length of a beam when designing structures with bending requirements.

Table of Contents

How long of a beam do I need to bend?

The length of a beam required for bending depends on several factors, including the type of material, the amount of weight or load the beam will need to support, the type of bending (e.g., cantilever, simply supported), and the acceptable level of deflection or sag. Here are some considerations when determining the length of a beam for bending:

Load and Weight Distribution:
- The most critical factor in determining the beam length is the weight or load that the beam needs to support. Heavier loads require longer beams. Consider not only the total weight but also how the load is distributed along the length of the beam.
Material and Strength:
- The material of the beam plays a significant role. Different materials have different load-bearing capacities. Consult engineering tables and guidelines for specific materials to determine their load capacities and bending moments.
Type of Bending:
- The type of bending the beam will experience affects the required length. For example, a simply supported beam has different requirements than a cantilever beam. The supports and loads will dictate the appropriate length.
Deflection Limits:
- The amount of acceptable deflection or sag in the beam is an important consideration. Excessive deflection can lead to structural issues. Check building codes and standards for acceptable deflection limits.
Safety Factors:
- Engineering codes and standards typically require the use of safety factors. This means that the calculated length should provide a significant margin of safety to ensure the beam can handle variations in load and other unforeseen factors.
Span-to-Depth Ratio:
- The span-to-depth ratio is an important consideration. A deeper beam, relative to its span, can better resist bending. If a beam is too shallow for its span, it may sag or deflect excessively.
Design Software:
- For complex or critical structural applications, consider using structural analysis and design software or consulting a structural engineer to determine the appropriate beam length.
Construction and Support Methods:
- The way the beam is supported and the construction methods used can also impact the required length. For example, a beam supported at both ends may require less length than one supported in the center (cantilever).
Environmental Factors:
- Environmental factors such as wind, seismic activity, and temperature fluctuations can influence beam design. Consider the potential impact of these factors on the beam's structural integrity.
Legal and Regulatory Requirements:
- Compliance with local building codes and regulations is essential. Consult with local building authorities or a structural engineer to ensure your beam design adheres to all relevant requirements.

In summary, determining the appropriate length for a bending beam involves a complex set of considerations, including load, material, type of bending, deflection limits, safety factors, span-to-depth ratios, and compliance with codes and regulations. The design of such structures should ideally be performed by a structural engineer or a professional with expertise in structural analysis and design to ensure the safety and stability of the structure.

Calculating Beam Length for Bending: What You Need to Know

To calculate the beam length required for bending, you need to consider the following factors:

The load to be supported: The greater the load, the longer the beam needs to be to prevent it from bending too much.
The material of the beam: Different materials have different strengths and weaknesses. For example, steel beams are stronger than wooden beams, so they can be shorter for the same load.
The shape of the beam: Beams with a cross-section that is deeper and wider are stronger and can be shorter for the same load.
The supports for the beam: Beams that are supported at both ends are stronger than beams that are supported at only one end.

Factors Affecting the Required Beam Length for Bending

In addition to the factors listed above, the following factors can also affect the required beam length for bending:

The safety factor: The safety factor is a number that is used to account for uncertainties in the design and construction of the beam and the load it will support. A higher safety factor means that the beam needs to be longer.
The deflection limit: The deflection limit is the maximum amount that the beam is allowed to bend before it fails. A lower deflection limit means that the beam needs to be longer.
The dynamic load: A dynamic load is a load that is applied to the beam suddenly, such as the impact of a falling object. A dynamic load requires a longer beam than a static load.

Using Formulas and Calculators for Beam Bending

There are a number of formulas and calculators that can be used to calculate the beam length required for bending. The most common formula is the Euler-Bernoulli beam equation:

L = π√(EI/(P * f))

where:

L is the length of the beam in meters
E is the modulus of elasticity of the beam material in Pascals
I is the moment of inertia of the beam cross-section in meters^4
P is the load on the beam in Newtons
f is the safety factor

Beam bending calculators are also available online and in engineering software programs. These calculators can be used to calculate the beam length required for bending, as well as other design parameters, such as the beam deflection and stress.

Safety Precautions When Working with Bent Beams

When working with bent beams, it is important to take the following safety precautions:

Always wear safety glasses and gloves.
Use proper lifting techniques to avoid back injuries.
Be aware of the load capacity of the beam and do not overload it.
Inspect the beam for any cracks or damage before using it.

Common Applications of Bent Beams in Engineering

Bent beams are used in a wide variety of engineering applications, including:

Buildings: Bent beams are used to support the floors and roofs of buildings.
Bridges: Bent beams are used to support the decks of bridges.
Cranes: Bent beams are used to support the booms of cranes.
Airplanes: Bent beams are used to support the wings and tail of airplanes.
Cars: Bent beams are used to support the chassis of cars.

Bent beams are an essential component of many different engineering structures. By understanding how to calculate the beam length required for bending, engineers can design safe and reliable structures.