Unraveling Noise Power Density: Calculating and Understanding

Noise power density, often referred to as noise spectral density or simply noise density, is a critical concept in various fields, including electronics, communications, and signal processing. It quantifies the power of noise (random fluctuations) per unit of bandwidth and is commonly expressed in units like dBm/Hz or Watts/Hz. Understanding and calculating noise power density is essential for designing and analyzing systems where noise plays a significant role. Here's how to calculate and understand noise power density:

1. Definition of Noise Power Density:

• Noise power density, denoted as N0 or Pn, represents the power of random noise in a given frequency range. It quantifies how much noise power is present per unit of frequency bandwidth.

2. Formula for Noise Power Density:

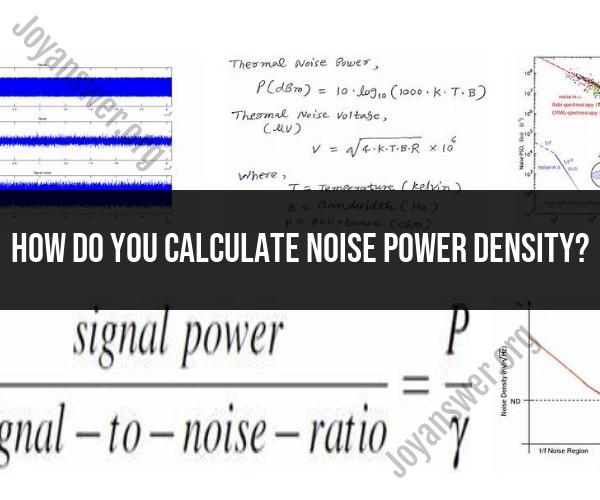
• The formula for noise power density (N0) is given by:

  N0 = k * T * B

  Where:

  • N0: Noise power density (Watts/Hz)
  • k: Boltzmann's constant (approximately 1.38 × 10⁻²³ J/K)
  • T: Temperature in Kelvin (K)
  • B: Bandwidth in Hertz (Hz)

3. Units of Noise Power Density:

• Noise power density is typically expressed in units such as Watts/Hz or dBm/Hz.
• In dBm/Hz, it is often measured in decibels referenced to 1 milliwatt per hertz.

4. Interpretation:

• Noise power density is directly proportional to temperature (T) and bandwidth (B). As either temperature or bandwidth increases, noise power density also increases.

5. Applications:

• Noise power density is crucial in electronic and communication systems design, where it affects the performance of receivers, amplifiers, and transmitters.
• It is used to calculate the noise floor of a system, which represents the minimum detectable signal level in the presence of noise.
• In telecommunications, understanding noise power density helps in designing systems with the desired signal-to-noise ratio (SNR) for reliable communication.

6. Example Calculation:

• Suppose you have a resistor at room temperature (T ≈ 300 K) and you want to calculate the noise power density over a 10 kHz bandwidth.
• Using the formula, N0 = (1.38 × 10⁻²³ J/K) * (300 K) * (10,000 Hz) = 4.14 × 10⁻15 Watts/Hz.
• If you want the result in dBm/Hz, you can convert it using the appropriate formula.

Understanding noise power density is fundamental in optimizing the performance of systems where signal quality is essential. By calculating and analyzing noise power density, engineers and scientists can make informed design decisions to minimize the impact of noise and improve the overall performance of electronic and communication systems.