Formula for Calculating a Standard Score: Explanation and Calculation

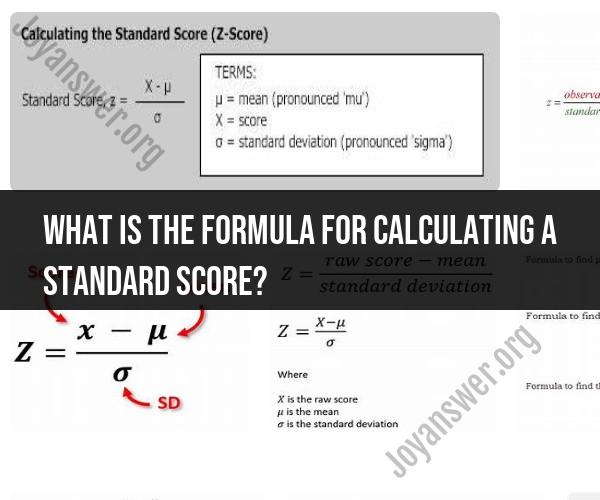
A standard score, also known as a z-score, is a measure used in statistics to determine how far a particular data point is from the mean (average) of a data set in terms of standard deviations. It helps in understanding the relative position of a data point within a distribution. The formula for calculating a standard score (z-score) is:

$z = \frac{x - \mu}{\sigma}$

Where:

• $z$ is the standard score (z-score) you want to calculate.
• $x$ is the individual data point you are interested in.
• $\mu$ (mu) is the mean (average) of the data set.
• $\sigma$ (sigma) is the standard deviation of the data set.

Here's how to calculate a standard score (z-score) step by step:

Step 1: Calculate the Mean ($\mu$)Find the mean (average) of the data set. Sum up all the values in the data set and then divide by the total number of values.

$\mu = \frac{\sum{x}}{n}$

Where:

• $\mu$ is the mean.
• $\sum{x}$ represents the sum of all the data points.
• $n$ is the total number of data points.

Step 2: Calculate the Standard Deviation ($\sigma$)Determine the standard deviation of the data set. The standard deviation measures the spread or dispersion of the data. You can use the following formula:

$\sigma = \sqrt{\frac{\sum{(x - \mu)^2}}{n}}$

Where:

• $\sigma$ is the standard deviation.
• $\sum{(x - \mu)^2}$ represents the sum of the squared differences between each data point ($x$) and the mean ($\mu$).
• $n$ is the total number of data points.

Step 3: Calculate the Standard Score ($z$)Now that you have the mean ($\mu$) and standard deviation ($\sigma$), you can calculate the standard score ($z$) for a specific data point ($x$) using the formula:

$z = \frac{x - \mu}{\sigma}$

The resulting $z$-score tells you how many standard deviations a data point is from the mean. If $z$ is positive, it means the data point is above the mean, and if $z$ is negative, it means the data point is below the mean.

Interpretation:

• A $z$-score of 0 indicates that the data point is exactly at the mean.
• A positive $z$-score means the data point is above the mean.
• A negative $z$-score means the data point is below the mean.
• The magnitude of the $z$-score indicates how many standard deviations the data point is from the mean. A larger magnitude suggests a greater deviation from the mean.

Standard scores (z-scores) are valuable for comparing data points from different distributions and understanding their relative positions within those distributions. They are commonly used in various fields of statistics and data analysis.