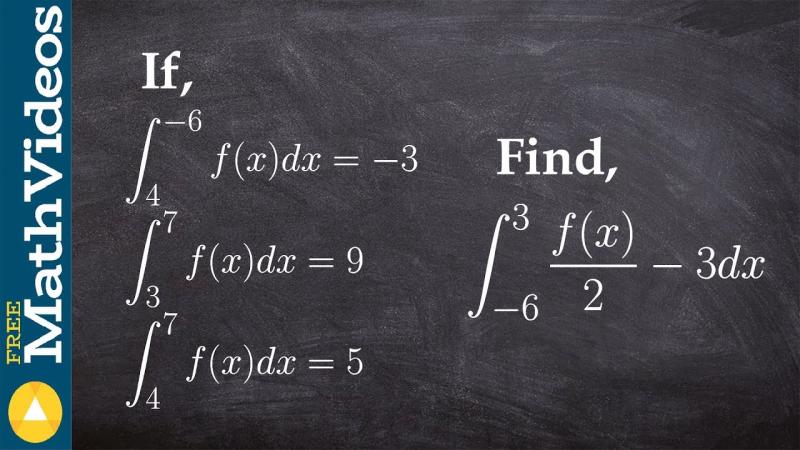Meaning of Definite Integral: Mathematical Explanation

_{January 25, 2024 by JoyAnswer.org, Category : Mathematics}

What does definite integral mean? Explore the meaning of a definite integral through a mathematical explanation. This article provides insights into the interpretation and significance of definite integrals.

What does definite integral mean?

The definite integral is a concept in calculus that represents a specific quantity, often interpreted as the signed area under a curve between two points on the x-axis. It has several interpretations and applications, and its meaning can be explained in different ways:

Signed Area Under a Curve:
- One of the primary interpretations of the definite integral is as the signed area between the curve $y = f(x)$ and the x-axis over a specified interval $[a, b]$ . The sign of the area is determined by whether the curve is above or below the x-axis.
Accumulation of Quantities:
- The definite integral can represent the accumulated quantity of a varying rate. If $f(x)$ represents a rate of change (e.g., velocity, flow rate, or any rate), then the definite integral of $f(x)$ from $a$ to $b$ represents the accumulated quantity of that rate over the interval $[a, b]$ .
Net Change:
- In the context of physics or other dynamic systems, the definite integral can represent the net change in a quantity over a given time period. For example, if $f(t)$ represents the rate of change of a quantity with respect to time, then the definite integral of $f(t)$ over a time interval gives the net change in the quantity during that time.
Displacement or Distance Traveled:
- When dealing with motion along a straight line, the definite integral of a velocity function gives the displacement of an object over a specified time interval. If the velocity is always positive or zero, the integral represents the distance traveled.
Average Value of a Function:
- The definite integral can also be used to find the average value of a function over an interval. The average value is calculated by dividing the integral of the function by the width of the interval.

Mathematically, the definite integral of a function $f(x)$ from $a$ to $b$ is denoted as $\int_{a}^{b} f(x) \,dx$ and can be calculated using various techniques, including the Fundamental Theorem of Calculus.

In summary, the definite integral provides a powerful tool for analyzing and quantifying quantities that change over time or space, making it a fundamental concept in calculus with broad applications in science, engineering, economics, and other fields.

What is the meaning of definite integral?

The meaning of a definite integral can be approached from two different perspectives: geometrical and mathematical.

Geometrical Meaning:

Imagine a function plotted on a graph, creating a shape between itself and the x-axis. A definite integral tells you the net signed area of that shape within a specific interval on the x-axis. This means:

If the function is above the x-axis, the area is positive.

If the function is below the x-axis, the area is negative.

The integral sums up all these positive and negative areas, accounting for their signs, to give you the net signed area enclosed within the chosen interval.

Mathematical Meaning:

A definite integral is a mathematical notation that quantifies the accumulation of a function's values over a specific interval. This means it captures the total sum of changes the function undergoes as you move along the x-axis within the chosen interval.

Here's an analogy: imagine walking along a winding path uphill and downhill. The definite integral tells you the net vertical distance you've climbed or descended at the end of your journey, taking into account all the ups and downs along the way.

In essence, a definite integral connects two ideas: