Finding and Simplifying the Difference Quotient: Step-by-Step Instructions

_{December 5, 2023 by JoyAnswer.org, Category : Mathematics}

How to find and simplify the difference quotient? Learn how to find and simplify the difference quotient effectively. This article provides step-by-step instructions and examples, guiding you through the process of computing and simplifying the difference quotient in calculus problems.

How to find and simplify the difference quotient?

Finding and simplifying the difference quotient involves several steps. Let's go through the process step by step using a specific example. Suppose you have a function $f(x) = 3x^2 + 2x + 1$ , and you want to find and simplify the difference quotient for this function.

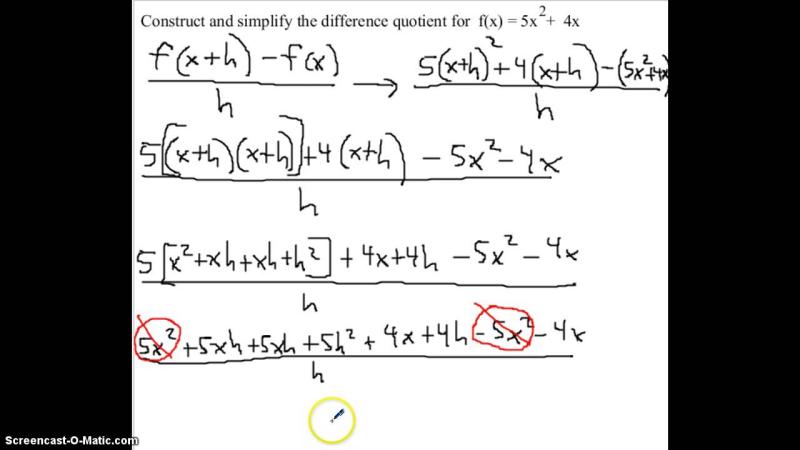
Write Down the Function:Start with the given function: $f(x) = 3x^2 + 2x + 1$ .
Set Up the Difference Quotient:The difference quotient is given by: $\frac{f(x + h) - f(x)}{h}$ Substitute the function $f(x)$ into this formula: $\frac{(3(x + h)^2 + 2(x + h) + 1) - (3x^2 + 2x + 1)}{h}$
Expand and Simplify:Expand and simplify the expressions within the numerator: $\frac{(3(x^2 + 2hx + h^2) + 2x + 2h + 1) - (3x^2 + 2x + 1)}{h}$ $\frac{3x^2 + 6hx + 3h^2 + 2x + 2h + 1 - 3x^2 - 2x - 1}{h}$ $\frac{6hx + 3h^2 + 2h}{h}$
Cancel Common Terms:Cancel any common terms in the numerator and denominator: $\frac{h(6x + 3h + 2)}{h}$ Cancel $h$ in the numerator and denominator: $6x + 3h + 2$
Remove the Limit Notation (Optional):If you're looking for the derivative at a specific point, you can substitute $h = 0$ to find the instantaneous rate of change: $f'(x) = 6x + 3(0) + 2 = 6x + 2$

So, the simplified difference quotient for the given function is $6x + 3h + 2$ , and the derivative is $6x + 2$ .

This is a general approach that can be applied to different functions. Just plug in the function into the difference quotient formula, expand, simplify, and then cancel common terms.

How can the difference quotient be located and simplified in mathematical problems?

Locating and simplifying the difference quotient in mathematical problems involves identifying the function to be differentiated, the point of evaluation, and the small interval around that point. The difference quotient formula is then applied to calculate the average rate of change over that interval.

Locating the Difference Quotient:

Identify the Function: Determine the function for which you want to approximate the instantaneous rate of change. This is typically given in the problem statement or context.

Choose the Point of Evaluation: Select the point (x) at which you want to approximate the instantaneous rate of change. This point represents the input value for the function.

Determine the Interval: Specify a small interval (h) around the point of evaluation. This interval represents the change in the input value.

Simplifying the Difference Quotient:

Substitute Values: Replace the function name (f) with the actual function expression, and substitute the point of evaluation (x) and the interval (h) into the difference quotient formula.

Simplify Expressions: Expand any parentheses, distribute terms, and simplify any algebraic expressions within the numerator and denominator.

Combine Like Terms: Combine any terms with similar factors or patterns to simplify the expression further.

Factor Out Common Factors: If possible, factor out common factors from the numerator or denominator to obtain a simplified form.

Cancel Common Factors: If the numerator and denominator have common factors, cancel them to further simplify the expression.

Rewrite as Rationalized Form: If the expression involves fractions with radicals, rationalize the denominators to eliminate any radicals from the simplified form.