Calculating Expected Return and Standard Deviation

_{December 5, 2023 by JoyAnswer.org, Category : Financial}

How do you calculate expected return and standard deviation? Learn how to calculate expected return and standard deviation. This article provides formulas and methodologies for determining these key financial metrics.

How do you calculate expected return and standard deviation?

Certainly! Calculating the expected return and standard deviation are crucial steps in analyzing the risk and potential rewards of an investment portfolio.

Expected Return:
- The expected return of an investment is the anticipated gain or loss from that investment over a specific period. It's calculated as the weighted average of all possible returns, with each return weighted by its respective probability.
- The formula for the expected return ( $E(R)$ ) is: $E(R) = \sum_{i=1}^{n} (R_i \times P_i)$ Where:
  - $R_i$ = Return of the ith possible outcome
  - $P_i$ = Probability of the ith outcome
  - $n$ = Number of possible outcomes
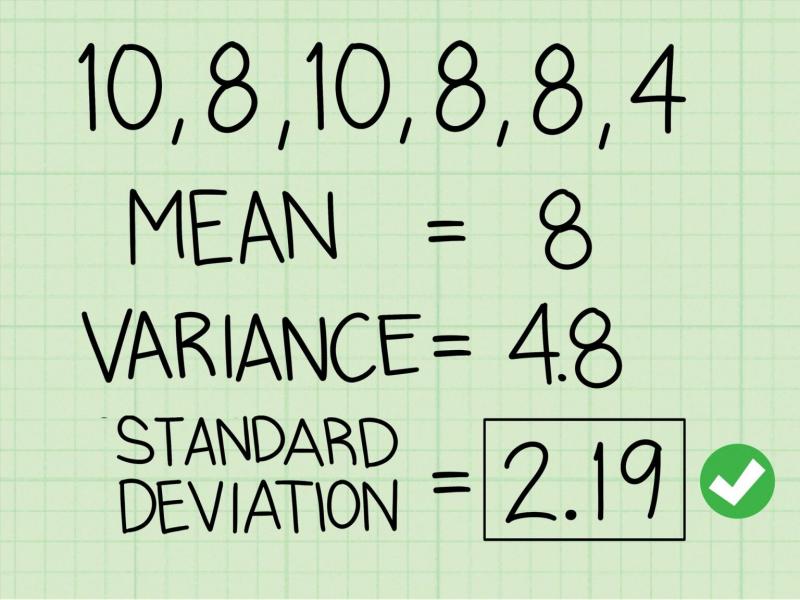
Standard Deviation:
- Standard deviation measures the dispersion or volatility of returns around the expected return. It gives an indication of the investment's risk. A higher standard deviation implies higher volatility and vice versa.
- The formula for standard deviation ( $\sigma$ ) involves several steps:
  - Calculate the expected return using the formula mentioned earlier.
  - Calculate the variance ( $\sigma^2$ ) by finding the average of the squared differences between each return and the expected return: $\sigma^2 = \sum_{i=1}^{n} [(R_i - E(R))^2 \times P_i]$
  - Finally, take the square root of the variance to get the standard deviation: $\sigma = \sqrt{\sigma^2}$

These calculations are fundamental in evaluating the risk and potential reward of investments or portfolios. They help investors make informed decisions by quantifying the expected performance and volatility of their investments based on historical data or expected outcomes.

How are expected return and standard deviation computed in financial analysis?

Expected return and standard deviation are two key measures used in financial analysis to evaluate the performance and risk of an investment.

Expected Return

Expected return is the average rate of return that investors expect to earn on an investment over a specified period of time. It is calculated by taking the weighted average of all possible returns, where the weights reflect the probability of each return occurring.

For instance, if there is a 50% chance of an investment returning 10% and a 50% chance of an investment returning 20%, the expected return would be 15%.

There are several methods for calculating expected return, including the following:

Arithmetic mean: This is the most common method and simply involves averaging the historical returns of an investment.
Geometric mean: This method is more appropriate for investments where returns are compounding over time. It takes into account the concept of compound interest, which means that each year's return is based on the previous year's return.
Dominant mean: This method takes into account both the expected return and the risk of the investment. It is calculated by weighting the expected returns of different investments by their probability of occurrence.

Standard Deviation

Standard deviation is a measure of volatility or risk. It measures the dispersion of returns around the expected return. A higher standard deviation indicates greater volatility, which means that the investment's returns are more likely to vary from the expected return.

Standard deviation is calculated using the following formula:

Standard deviation = √Σ(R - E)^2 / n

where:

R is the actual return of the investment
E is the expected return
n is the number of periods of data

For example, if an investment has an expected return of 10% and has historical returns of 5%, 15%, 10%, and 12%, the standard deviation would be 2.25%.

Standard deviation is a valuable tool for assessing the risk of an investment. It can help investors understand the likelihood of experiencing losses and make informed decisions about their investments.