Unveiling Simple Harmonic Motion: Conditions and Characteristics

_{February 3, 2024 by JoyAnswer.org, Category : Physics}

What are the conditions of simple harmonic motion? Explore the conditions that define simple harmonic motion. This article provides insights into the characteristics and requirements for a system to exhibit simple harmonic motion.

What are the conditions of simple harmonic motion?

Simple Harmonic Motion (SHM) is a type of periodic motion where an object oscillates back and forth around a stable equilibrium position. Several conditions must be met for a motion to be considered simple harmonic:

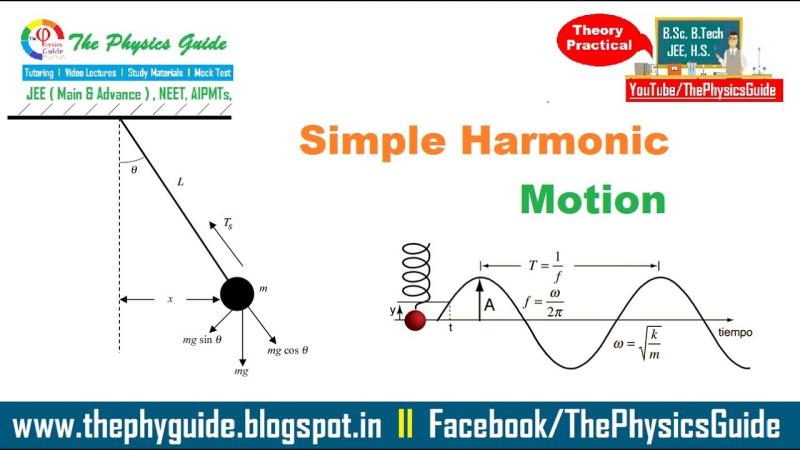
Restoring Force Proportional to Displacement: The restoring force acting on the object should be directly proportional to its displacement from the equilibrium position and always directed towards that position. Mathematically, this can be expressed as $F = -kx$ , where $F$ is the restoring force, $k$ is the force constant (spring constant), and $x$ is the displacement from equilibrium.
Linear Restoring Force: The restoring force should be linearly proportional to the displacement. This means that the graph of force against displacement should be a straight line passing through the origin.
Direction of Force Opposite to Displacement: The restoring force acting on the object should always be directed opposite to the displacement from the equilibrium position. This ensures that the object is pulled back towards the equilibrium position.
Periodic Motion: The motion should be periodic, meaning that the object repeats its motion after a regular interval of time.
No Energy Loss: In an ideal scenario, there should be no energy loss due to friction or other dissipative forces. This is rarely achieved in real-world situations, but it helps in maintaining a constant amplitude of oscillation.

These conditions ensure that the motion is simple harmonic, allowing for a sinusoidal or cosine-like behavior over time.

Characteristics of Simple Harmonic Motion:

Amplitude ( $A$ ): The maximum displacement from the equilibrium position to either side.
Period ( $T$ ): The time taken for one complete oscillation or cycle.
Frequency ( $f$ ): The number of oscillations per unit time. It is the reciprocal of the period ( $f = \frac{1}{T}$ ).
Angular Frequency ( $\omega$ ): The rate of change of angular displacement with respect to time. It is related to frequency by the equation $\omega = 2\pi f$ .
Phase Constant ( $\phi$ ): Represents the phase angle or initial phase of the motion. It determines the starting position of the oscillation.
Velocity ( $v$ ): The rate of change of displacement with respect to time. It is zero at the equilibrium position and maximum at the amplitude.
Acceleration ( $a$ ): The rate of change of velocity with respect to time. It is maximum at the equilibrium position and zero at the amplitude.

Understanding these conditions and characteristics helps in analyzing and describing simple harmonic motion in various physical systems.

Understanding Simple Harmonic Motion:

1. Defining Simple Harmonic Motion (SHM):

SHM is a specific type of periodic motion where an object experiences a restoring force proportional to its displacement from equilibrium and directed towards the equilibrium position. Key characteristics include:

Proportionality: The restoring force gets stronger as the object moves further away from its equilibrium point. Think of a stretched rubber band pulling back harder the more it's stretched.

Direction: The force always acts in the opposite direction of the displacement, pushing the object back towards its equilibrium position.

Periodicity: The motion repeats itself in regular intervals called the period (T), meaning the object takes the same time to complete one cycle of back-and-forth movement.

2. Distinguishing SHM from Other Oscillations:

While SHM exhibits repeating back-and-forth motion, it differs from other oscillations in two main ways:

Restoring force: In SHM, the restoring force is proportional to the displacement, whereas other oscillations might have different force relationships, like a constant force (e.g., gravity pulling down on a pendulum).

Energy conservation: An ideal SHM system experiences no energy loss due to friction or other dissipative forces. This means its total mechanical energy (kinetic + potential) remains constant throughout the motion. Real-world oscillations typically have some energy loss, making them damped oscillations.

3. Real-World Examples of SHM:

While ideal SHM exists primarily in theory, several real-world systems exhibit close approximations:

Mass-spring system: A mass attached to a spring stretched from its equilibrium. As the mass moves away, the spring pulls it back with a force proportional to the displacement.

Simple pendulum: For small angles, the restoring force due to gravity acts approximately proportional to the pendulum's angular displacement.

Tuning forks: The metal prongs vibrate with SHM when struck, producing a pure tone.

Acoustical waves in air: Sound waves, under certain conditions, can be approximated as traveling SHM disturbances in air pressure.

It's important to remember that real-world systems often involve additional factors like friction, damping, and non-linear forces, leading to deviations from perfect SHM. However, understanding the principles of SHM helps analyze and predict the behavior of many oscillating systems found in nature and technology.