What is the formula for average angular acceleration?
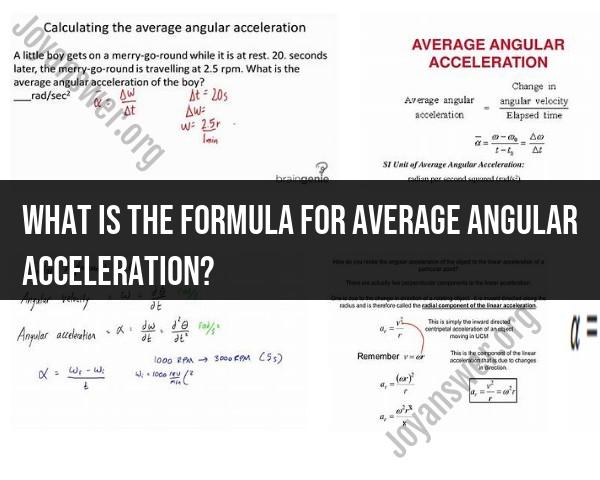
The formula for average angular acceleration () is given by:
Where:
- is the average angular acceleration,
- is the change in angular velocity,
- is the change in time.
Angular acceleration is a measure of how quickly the angular velocity of an object changes over time. The unit of angular acceleration is typically radians per second squared (rad/s²). If the angular velocity is measured in radians per second (rad/s) and time in seconds (s), then the unit of angular acceleration is .
Average Angular Acceleration Equation
The average angular acceleration is defined by the following equation:
α = Δω / Δt
where:
- α is the average angular acceleration (measured in radians per second squared)
- Δω is the change in angular velocity (measured in radians per second)
- Δt is the change in time (measured in seconds)
This equation essentially calculates the rate of change of angular velocity over time. The average angular acceleration tells us how quickly an object is speeding up or slowing down its rotation.
Calculating Average Angular Acceleration
Here are the steps to calculate average angular acceleration:
- Identify the initial and final angular velocities (ω₁ and ω₂) of the object.
- Calculate the change in angular velocity by subtracting the initial from the final angular velocity: Δω = ω₂ - ω₁.
- Identify the time interval (Δt) during which the angular velocity changed.
- Substitute the values of Δω and Δt into the equation: α = Δω / Δt.
Example:
A spinning object initially has an angular velocity of 10 radians per second. After 5 seconds, its angular velocity increases to 20 radians per second. What is the average angular acceleration?
Solution:
- ω₁ = 10 rad/s
- ω₂ = 20 rad/s
- Δω = ω₂ - ω₁ = 20 rad/s - 10 rad/s = 10 rad/s
- Δt = 5 seconds
- α = Δω / Δt = 10 rad/s / 5 s = 2 rad/s²
Therefore, the average angular acceleration of the object is 2 rad/s².