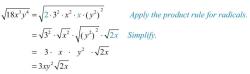Solving Two Triangle Trigonometry Problems: Step-by-Step Approach

I'll provide a step-by-step approach to solving two triangle trigonometry problems. These problems involve using trigonometric ratios (sine, cosine, and tangent) to find missing side lengths and angles in triangles.

Problem 1: Finding a Missing Side Length

Problem Statement: In a right triangle, the length of one leg (adjacent to the angle θ) is 8 units, and the measure of angle θ is 30 degrees. Find the length of the hypotenuse.

Solution:

1. Identify the given information:

   • Length of one leg (adjacent to θ) = 8 units
   • Angle θ = 30 degrees

2. Determine which trigonometric ratio to use. Since we know the adjacent side length and the angle θ, we can use the cosine ratio (cosθ), which is defined as:

   cosθ = adjacent side / hypotenuse

3. Substitute the known values into the cosine ratio equation:

   cos(30°) = 8 / hypotenuse

4. Solve for the hypotenuse:

   hypotenuse = 8 / cos(30°)

   Use a calculator to find the value of cos(30°), which is √3/2.

   hypotenuse = 8 / (√3/2)

   To simplify further, multiply the numerator and denominator by 2:

   hypotenuse = (8 * 2) / √3

   hypotenuse = 16 / √3

   Rationalize the denominator by multiplying both the numerator and denominator by √3:

   hypotenuse = (16 * √3) / (3)

5. Calculate the value of the hypotenuse:

   hypotenuse ≈ 9.24 units (rounded to two decimal places)

Problem 2: Finding an Angle Measure

Problem Statement: In a right triangle, the length of one leg is 5 units, and the length of the hypotenuse is 13 units. Find the measure of the acute angle θ.

Solution:

1. Identify the given information:

   • Length of one leg = 5 units
   • Length of the hypotenuse = 13 units

2. Determine which trigonometric ratio to use. Since we know the length of the leg and the hypotenuse, we can use the sine ratio (sinθ), which is defined as:

   sinθ = opposite side / hypotenuse

3. Substitute the known values into the sine ratio equation:

   sinθ = 5 / 13

4. Solve for the angle θ:

   To find θ, take the inverse sine (or arcsin) of the value:

   θ = arcsin(5/13)

   Use a calculator to find the approximate value of θ:

   θ ≈ 22.62 degrees (rounded to two decimal places)

So, the measure of the acute angle θ is approximately 22.62 degrees.

To solve two triangle trigonometry problems, you can follow these steps:

1. Identify the givens and the unknowns. For each triangle, identify the sides and angles that are given, as well as the sides and angles that you need to solve for.
2. Choose the appropriate trigonometric functions. The trigonometric functions that you use will depend on the givens and the unknowns. For example, if you know two sides and need to solve for an angle, you would use the law of sines or the law of cosines.
3. Set up equations. Use the givens and the trigonometric functions to set up equations that relate the sides and angles of the triangle.
4. Solve the equations. Use algebraic methods to solve the equations for the unknowns.
5. Check your answers. Once you have solved for the unknowns, check your answers to make sure that they make sense.

Here is an example of how to solve two triangle trigonometry problems:

Problem 1:

Triangle ABC is a right triangle, with angle A = 90 degrees, angle B = 30 degrees, and side BC = 10 cm. Find the length of side AC.

Solution:

We know the side opposite to angle B, and we need to solve for the side adjacent to angle B. Therefore, we can use the tan function.

tan B = AC / BC

tan 30 degrees = AC / 10 cm

AC = tan 30 degrees * 10 cm

AC = 5.77 cm

Problem 2:

Triangle DEF is a right triangle, with angle D = 90 degrees, angle E = 60 degrees, and side DE = 12 cm. Find the length of side EF.

Solution:

We know the side opposite to angle E, and we need to solve for the side adjacent to angle E. Therefore, we can use the sqrt(3) / 2 function.

sqrt(3) / 2 = EF / DE

EF = sqrt(3) / 2 * 12 cm

EF = 6 cm

These are just two examples of how to solve two triangle trigonometry problems. There are many other types of triangle problems that can be solved using trigonometry. The key is to identify the givens, choose the appropriate trigonometric functions, set up equations, solve the equations, and check your answers.