The Six Trigonometry Functions of a Triangle: Explained

What are the six trigonometry functions of Triangel?

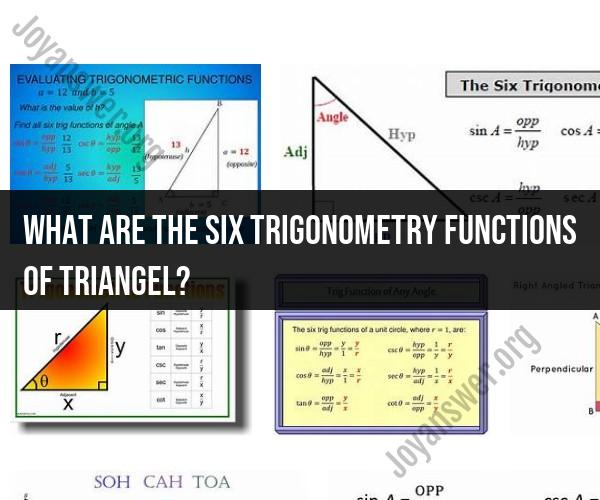
In trigonometry, there are six trigonometric functions that are commonly used to describe the relationships between the sides and angles of a right triangle. These functions are sine, cosine, tangent, cosecant, secant, and cotangent, often abbreviated as sin, cos, tan, csc, sec, and cot. Here's an explanation of each function:

1. Sine (sin):

   • The sine of an angle in a right triangle is the ratio of the length of the side opposite that angle to the length of the hypotenuse (the longest side of the triangle). It is represented as:
   • sin(θ) = Opposite side / Hypotenuse

2. Cosine (cos):

   • The cosine of an angle is the ratio of the length of the adjacent side (the side adjacent to the angle but not the hypotenuse) to the length of the hypotenuse. It is represented as:
   • cos(θ) = Adjacent side / Hypotenuse

3. Tangent (tan):

   • The tangent of an angle is the ratio of the length of the side opposite that angle to the length of the adjacent side. It is represented as:
   • tan(θ) = Opposite side / Adjacent side

4. Cosecant (csc):

   • The cosecant of an angle is the reciprocal of the sine function. It is represented as:
   • csc(θ) = 1 / sin(θ)

5. Secant (sec):

   • The secant of an angle is the reciprocal of the cosine function. It is represented as:
   • sec(θ) = 1 / cos(θ)

6. Cotangent (cot):

   • The cotangent of an angle is the reciprocal of the tangent function. It is represented as:
   • cot(θ) = 1 / tan(θ)

These trigonometric functions are fundamental in trigonometry and are used to solve a wide range of problems involving triangles, angles, and periodic phenomena. They are particularly useful in fields such as physics, engineering, and navigation for calculating distances, angles, and various other measurements.