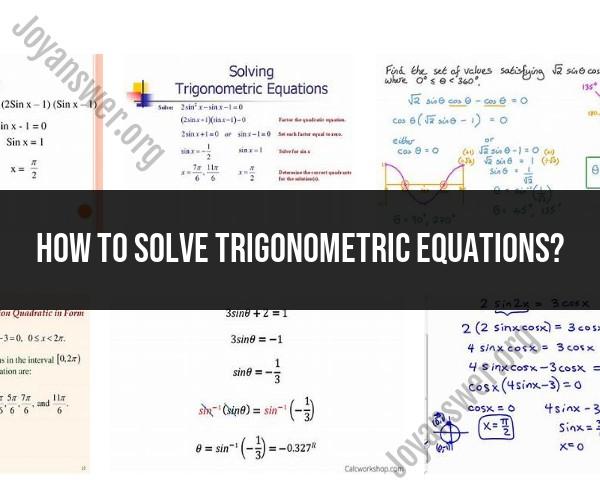
Solving Trigonometric Equations: Strategies and Techniques

Solving trigonometric equations involves finding the values of the unknown variable(s) that satisfy a given trigonometric equation. Here are some common strategies and techniques for solving trigonometric equations:

1. Use Trigonometric Identities:

   • Sometimes, trigonometric identities like the Pythagorean identities (e.g., sin²θ + cos²θ = 1) can be used to simplify equations or express one trigonometric function in terms of another. This can be particularly helpful for transforming complex equations into simpler ones.

2. Isolate the Trigonometric Function:

   • If the equation contains a single trigonometric function, you can often isolate it by performing inverse trigonometric operations (e.g., taking the arcsin, arccos, or arctan) on both sides of the equation. This can help you find the values of the variable within a specific interval.

3. Use Trigonometric Properties:

   • Trigonometric properties such as periodicity and symmetry can be useful for solving equations. For example, if you know that sin(θ) = sin(α), you can use the periodicity of sine to find other solutions by adding integer multiples of 2π to α.

4. Factor and Simplify:

   • Factor the equation and look for common terms that can be canceled out or simplified. This can lead to a simpler equation that is easier to solve.

5. Apply Trigonometric Rules:

   • Familiarize yourself with trigonometric rules and equations such as the double-angle, half-angle, and sum/difference identities. These can often be applied to transform equations into more manageable forms.

6. Use Trigonometric Substitution:

   • In some cases, you may need to use trigonometric substitution to transform a complex equation into a simpler algebraic equation. This is commonly used in calculus, especially when dealing with integrals.

7. Check for Extraneous Solutions:

   • When solving trigonometric equations, it's essential to check your solutions to ensure they are valid within the specified domain. Some solutions may be extraneous and not satisfy the original equation due to restricted domains or mathematical operations.

8. Graphical Approach:

   • Graph the trigonometric functions involved in the equation and identify the points of intersection with a horizontal line representing the value on the right side of the equation. The x-coordinates of these intersections are the solutions.

9. Use a Calculator:

   • For complex equations, a graphing calculator or a computer algebra system (CAS) can be invaluable for finding numerical solutions or verifying algebraic solutions.

10. Practice:

    • Solving trigonometric equations becomes more comfortable with practice. Familiarity with trigonometric functions, identities, and techniques will help you become more efficient in solving various types of equations.

Remember that trigonometric equations can have multiple solutions, and it's essential to consider all possible solutions within the given domain. Additionally, some equations may have no real solutions or may require numerical methods to approximate solutions.