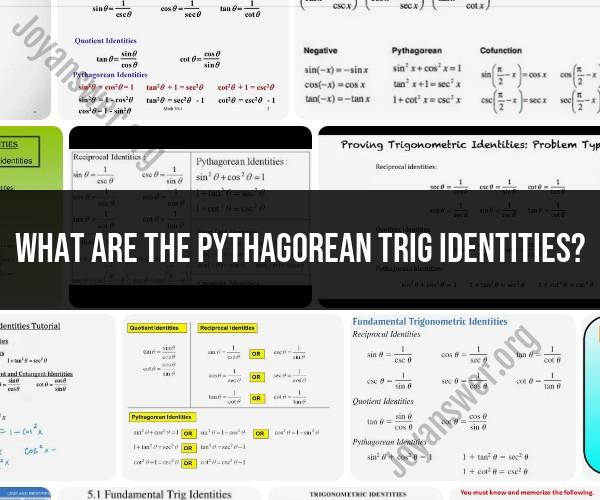
Exploring Pythagorean Trig Identities: Applications and Insights

The Pythagorean trigonometric identities are a set of three fundamental trigonometric identities that are derived from the Pythagorean theorem. These identities relate the trigonometric functions sine (sin), cosine (cos), and tangent (tan) of an angle in a right triangle. The Pythagorean trigonometric identities are as follows:

1. Pythagorean Identity:

   $$\sin^2(\theta) + \cos^2(\theta) = 1$$

   This identity states that the square of the sine of an angle plus the square of the cosine of the same angle is always equal to 1. It's one of the most fundamental trigonometric identities and is derived directly from the Pythagorean theorem in a unit circle.

2. Reciprocal Identity:

   $$\sec^2(\theta) = 1 + \tan^2(\theta) \quad \text{and} \quad \csc^2(\theta) = 1 + \cot^2(\theta)$$

   These two identities express the relationships between the secant (sec), cosecant (csc), and tangent (tan) and cotangent (cot) functions. They are derived from the Pythagorean identity by dividing each term by $\cos^2(\theta)$ or $\sin^2(\theta)$, respectively.

3. Tangent Identity:

   $$\tan(\theta) = \frac{\sin(\theta)}{\cos(\theta)}$$

   This identity defines the tangent of an angle as the ratio of the sine to the cosine of that angle.

These Pythagorean trigonometric identities are essential in trigonometry and are used to simplify trigonometric expressions, solve trigonometric equations, and establish relationships between trigonometric functions. They provide a foundation for many other trigonometric identities and calculations.

Applications and Insights:Pythagorean trig identities are foundational in trigonometry and have various applications, including:

• Simplifying trigonometric expressions: These identities allow you to rewrite complex trigonometric expressions in simpler forms.
• Solving trigonometric equations: You can use these identities to transform equations involving trigonometric functions into equations that are easier to solve.
• Deriving other trigonometric identities: Many other trigonometric identities can be derived from the Pythagorean identities.
• Analyzing periodic behavior: Pythagorean trig identities are crucial in understanding the periodic behavior of sine, cosine, and tangent functions.

These identities are essential tools for solving trigonometric problems, working with triangles, and understanding the relationships between trigonometric functions. They provide insights into the geometric properties of the unit circle and are fundamental in various fields such as physics, engineering, and mathematics.