Definition of Definite Integral: Understanding Mathematical Concepts

_{January 25, 2024 by JoyAnswer.org, Category : Mathematics}

What is the definition of a definite integral? Understand the definition of a definite integral and delve into mathematical concepts. This article provides insights into the meaning and applications of definite integrals.

What is the definition of a definite integral?

In calculus, the definite integral is a mathematical concept that represents the signed area under a curve between two specific points on the x-axis. It is denoted by the symbol ∫ (integral sign) with limits of integration.

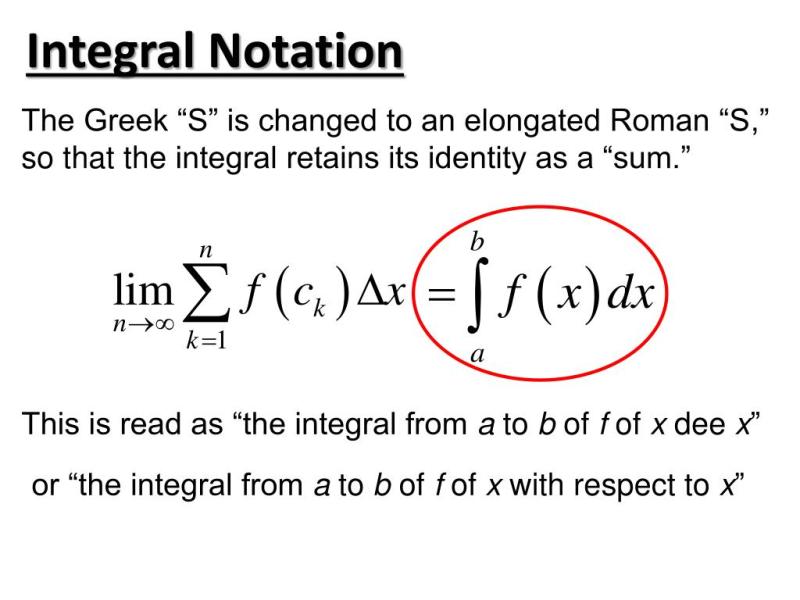
The definite integral of a function $f(x)$ from $a$ to $b$ is written as: $\int_{a}^{b} f(x) \,dx$

Here's a breakdown of the components in this expression:

The symbol $\int$ represents the integral.
$a$ and $b$ are the lower and upper limits of integration, respectively.
$f(x)$ is the function being integrated with respect to $x$ .
$dx$ indicates that the integration is with respect to the variable $x$ .

The process of finding a definite integral involves dividing the area under the curve into small rectangles, determining the area of each rectangle, and summing up these areas. As the width of the rectangles approaches zero (in a limiting process), the sum of these areas converges to the definite integral.

Mathematically, the definite integral is often interpreted as the limit of a Riemann sum and is defined as: $\int_{a}^{b} f(x) \,dx = \lim_{n \to \infty} \sum_{i=1}^{n} f(x_i) \Delta x$

where $x_i$ are sample points in the subintervals, and $\Delta x$ is the width of each subinterval.

In practical terms, the definite integral represents the net signed area under the curve $y = f(x)$ between the points $x = a$ and $x = b$ . The sign of the area is determined by the behavior of the function above and below the x-axis.

Applications of definite integrals include calculating areas, finding displacement, and determining accumulated quantities in various fields such as physics, economics, and engineering.

Could you provide a definition for definite integral?

In calculus, a definite integral represents the net signed area between a function and the x-axis over a specific interval. It's a powerful tool for calculating various quantities, like accumulated change, total work done, or the mass of an object with varying density.

Here's a breakdown of the key concepts:

Function: A mathematical rule that assigns a unique output (y-value) for each input (x-value).

Interval: A defined range of values on the x-axis, like [a, b].

Net signed area: The total area bounded by the curve of the function, the x-axis, and the interval endpoints, considering positive areas above the x-axis and negative areas below it.

Integral notation: Represented by the symbol ∫ with the function and interval limits inside: ∫_a^b f(x) dx.

The definite integral of f(x) over the interval [a, b] is evaluated through a process called integration. This involves Riemann sums, where the area under the curve is approximated by dividing the interval into smaller rectangles and summing their areas. As the number of rectangles increases, this approximation becomes more accurate and converges to the true definite integral value.

The definite integral has numerous applications in physics, engineering, economics, and other fields. It allows us to:

Calculate work done by a force over a distance

Find the total displacement of an object over time

Measure the total fluid flow through a pipe

Determine the center of mass of an object with non-uniform density

Understanding the concept of the definite integral opens doors to exploring various real-world phenomena and solving complex problems related to change, accumulation, and areas under curves.

I hope this definition clarifies the essence of the definite integral! Feel free to ask if you have further questions or want to delve deeper into specific aspects.