Equation for Reflection: Light Phenomenon

_{November 23, 2023 by JoyAnswer.org, Category : Physics}

What is the equation for reflection? Explore the equation governing the phenomenon of reflection, particularly in the context of light. Understand how the reflection of light is mathematically described.

What is the equation for reflection?

The law of reflection describes the relationship between the angle of incidence and the angle of reflection when light reflects off a surface. The law of reflection is typically expressed mathematically as follows:

$\theta_{\text{incident}} = \theta_{\text{reflected}}$

where:

$\theta_{\text{incident}}$ is the angle of incidence (the angle between the incident ray and the normal to the surface at the point of incidence),
$\theta_{\text{reflected}}$ is the angle of reflection (the angle between the reflected ray and the normal to the surface at the point of reflection).

This law holds true for specular reflection, where light reflects off a smooth surface, such as a mirror. It does not necessarily apply to diffuse reflection, which occurs when light reflects off a rough or irregular surface. In the case of diffuse reflection, the angles of incidence and reflection are not well-defined as they are in specular reflection.

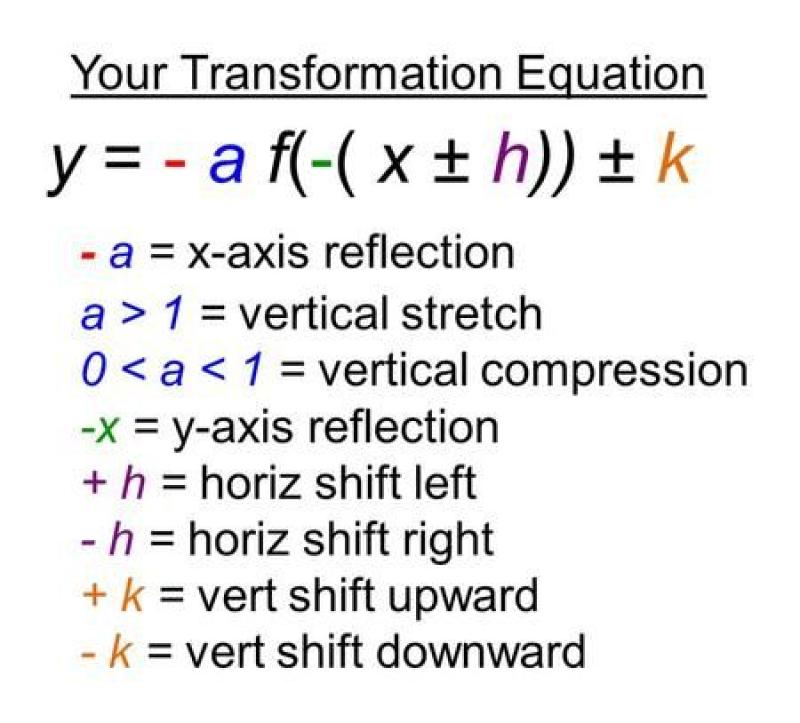
Reflection is a fundamental concept in physics and mathematics, representing the process of flipping an object or a point across a mirror or a line. It involves preserving the distance between the original object and its reflected image while reversing the direction of that distance.

In mathematics, reflection is often represented by a transformation matrix, which is a mathematical operator that encodes the geometric changes involved in reflecting an object or a point. The transformation matrix for reflection depends on the specific type of reflection, such as reflection over a line or reflection over a plane.

Here's a breakdown of your questions:

Equation for Reflection: The equation for reflection depends on the type of reflection being considered. For instance, reflection over the x-axis involves negating the y-coordinate of the original point, while reflection over the y-axis involves negating the x-coordinate.

Mathematical Formula for Reflection: The general mathematical formula for reflection over a line is:

(x', y') = R(x, y)

where:

(x, y) represents the original point

(x', y') represents the reflected point

R is the reflection matrix

The specific form of the reflection matrix depends on the orientation and position of the line of reflection. For example, reflection over the x-axis can be represented by the matrix:

R_x = [-1, 0; 0, 1]

Similarly, reflection over the y-axis can be represented by the matrix:

R_y = [1, 0; 0, -1]

Expressing Reflection in an Equation: The concept of reflection is expressed in an equation by defining the transformation matrix that maps the original point to its reflected image. This transformation matrix encodes the geometric changes involved in reflecting the point across the line or plane of reflection.

Remember, the specific form of the reflection equation depends on the type of reflection and the coordinate system being used.