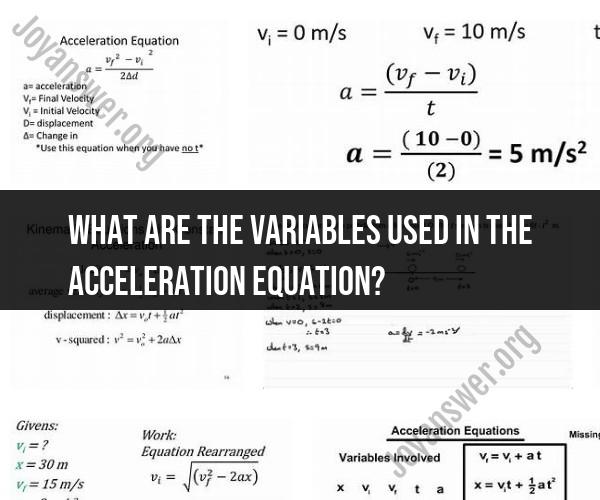
What are the variables used in the acceleration equation?
The acceleration equation relates the acceleration of an object to the net force applied to it and its mass. This equation is expressed as:
Where:
- represents the net force applied to the object (measured in newtons, N).
- is the mass of the object (measured in kilograms, kg).
- is the acceleration of the object (measured in meters per second squared, m/s²).
Here's an explanation of each variable:
Net Force ():
- Net force refers to the overall force acting on an object, considering both the magnitude and direction. It's the vector sum of all the forces acting on the object. For example, if an object is subject to multiple forces in different directions, you calculate the net force by adding up these forces, taking into account their directions.
Mass ():
- Mass is a measure of the amount of matter in an object. It quantifies how much material an object is made of and is typically measured in kilograms (kg) in the International System of Units (SI). Mass is a scalar quantity, meaning it only has magnitude and no direction.
Acceleration ():
- Acceleration is the rate of change of an object's velocity with respect to time. It tells you how quickly the velocity of an object is changing, as well as the direction of that change. Acceleration is a vector quantity, meaning it has both magnitude (how much) and direction (which way). It is measured in meters per second squared (m/s²).
In practical terms, the acceleration equation (Newton's second law of motion) tells us that the acceleration of an object is directly proportional to the net force applied to it and inversely proportional to its mass. This means that a larger net force will result in greater acceleration, and a larger mass will result in less acceleration for the same force.
In summary, the acceleration equation is a fundamental equation in physics that relates the net force applied to an object, its mass, and its resulting acceleration. Understanding this equation is crucial for analyzing the motion of objects and predicting their behavior under the influence of forces.
The acceleration equation describes how an object's velocity changes over time due to the influence of forces acting upon it. In physics, acceleration (a) is defined as the rate of change of velocity (v) with respect to time (t). The standard formula for acceleration is:
a = (Δv) / (Δt)
Here are the key variables used in the acceleration equation:
a (Acceleration): This is the quantity you're trying to calculate. Acceleration is a vector quantity, meaning it has both magnitude and direction. It is measured in units like meters per second squared (m/s²) in the International System of Units (SI).
Δv (Change in Velocity): This represents the change in an object's velocity over a specific time interval. It is typically measured in units of velocity, such as meters per second (m/s) or kilometers per hour (km/h). Δv can be calculated as the final velocity (vf) minus the initial velocity (vi):
Δv = vf - vi
Δt (Change in Time): This is the time interval over which the change in velocity occurs. It is typically measured in seconds (s).
In summary, the acceleration equation relates the change in velocity (Δv) to the time interval (Δt) over which this change occurs, allowing you to calculate the acceleration (a) experienced by an object. This equation is fundamental in understanding the motion of objects under the influence of forces, as it helps quantify how quickly an object's velocity is changing.