Converting Equations to Slope-Intercept Form: Step-by-Step Process

_{November 15, 2023 by JoyAnswer.org, Category : Mathematics}

How do you turn an equation into slope intercept form? Master the step-by-step process of turning an equation into slope-intercept form with this comprehensive guide. Understand the key principles behind this transformation for better mathematical insights.

Table of Contents

How do you turn an equation into slope intercept form?

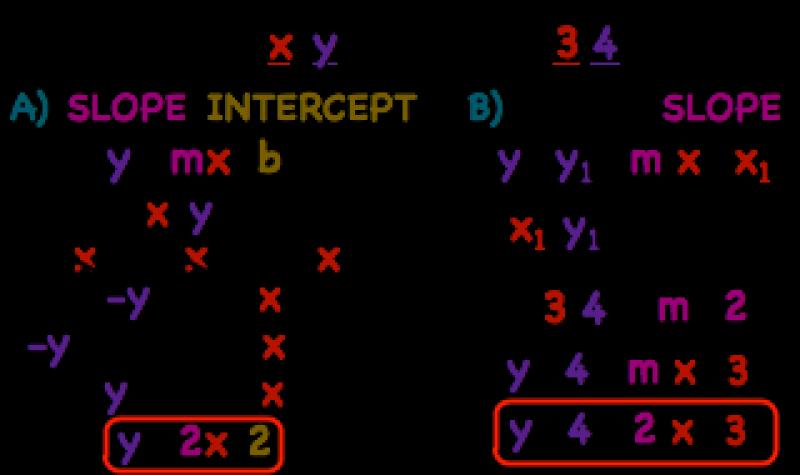
The slope-intercept form of a linear equation is given by:

$y = mx + b$

where:

$y$ is the dependent variable (output),
$x$ is the independent variable (input),
$m$ is the slope of the line,
$b$ is the y-intercept, which is the value of $y$ when $x$ is 0.

To turn an equation into slope-intercept form, you generally need to solve for $y$ and express the equation in the form $y = mx + b$ . Here's a step-by-step process:

Start with the Equation:
- Begin with the given equation, which may be in a different form, such as standard form or point-slope form.
Isolate $y$ :
- If the equation is not already solved for $y$ , isolate $y$ on one side of the equation. This might involve performing various algebraic operations.
Rearrange Terms:
- Rearrange the equation to get $y$ on one side and all other terms on the other side. The goal is to have the equation in the form $y = mx + b$ .
Identify the Slope ( $m$ ):
- Once the equation is in the form $y = mx + b$ , identify the coefficient of $x$ . This coefficient is the slope ( $m$ ) of the line.
Identify the Y-Intercept ( $b$ ):
- Identify the constant term (the term without $x$ ). This constant term is the y-intercept ( $b$ ).
Write in Slope-Intercept Form:
- Write the equation in the slope-intercept form $y = mx + b$ , substituting the values of $m$ and $b$ that you identified.

Here's an example:

Suppose you have the equation $2x - 3y = 6$ , and you want to write it in slope-intercept form.

Start with the Equation:
- $2x - 3y = 6$
Isolate $y$ :
- Subtract $2x$ from both sides: $-3y = -2x + 6$
Rearrange Terms:
- Divide both sides by -3 to isolate $y$ : $y = \frac{2}{3}x - 2$
Identify the Slope ( $m$ ):
- The coefficient of $x$ is $\frac{2}{3}$ , so the slope ( $m$ ) is $\frac{2}{3}$ .
Identify the Y-Intercept ( $b$ ):
- The constant term is -2, so the y-intercept ( $b$ ) is -2.
Write in Slope-Intercept Form:
- The equation in slope-intercept form is $y = \frac{2}{3}x - 2$ .

Now, the equation is in the slope-intercept form, making it easy to identify the slope and y-intercept.

Equation transformation: How to turn an equation into slope-intercept form

In mathematics, the slope-intercept form is a widely used method for representing linear equations. It provides a clear and straightforward way to visualize and analyze linear relationships between variables. To transform an equation into slope-intercept form, follow these steps:

Understanding slope-intercept form: The basics of y = mx + b

The slope-intercept form of a linear equation is expressed as:

y = mx + b

where:

y represents the dependent variable, which is the variable being measured or predicted.
x represents the independent variable, which is the variable that is manipulated or controlled.
m represents the slope of the line, which indicates the steepness and direction of the line.
b represents the y-intercept, which is the point where the line crosses the y-axis.

Step-by-step conversion: Converting equations to slope-intercept form

To convert an equation to slope-intercept form, follow these steps:

Isolate y: Move all terms containing y to one side of the equation.
Combine like terms: Combine any constant terms on the right side of the equation.
Express the equation in y = mx + b format: Rewrite the equation in the form y = mx + b, where m is the slope and b is the y-intercept.

Graphical insights: Visualizing equations in slope-intercept form

The slope-intercept form provides valuable graphical insights into linear relationships. The slope, represented by m, determines the steepness and direction of the line. A positive slope indicates that the line rises from left to right, while a negative slope indicates that the line falls from left to right. The y-intercept, represented by b, indicates the point where the line intersects the y-axis. This point is always located on the y-axis at the value of b.

Applications in real life: Practical uses of slope-intercept form in various fields

The slope-intercept form has numerous applications in various fields, including:

Physics: Modeling motion and relationships between variables such as velocity and time.
Engineering: Designing structures and analyzing forces and their effects.
Economics: Studying trends in financial markets and analyzing supply and demand relationships.
Biology: Investigating growth rates and population dynamics.
Education: Assessing student performance and evaluating the effectiveness of teaching methods.