What is an example of slope intercept form?
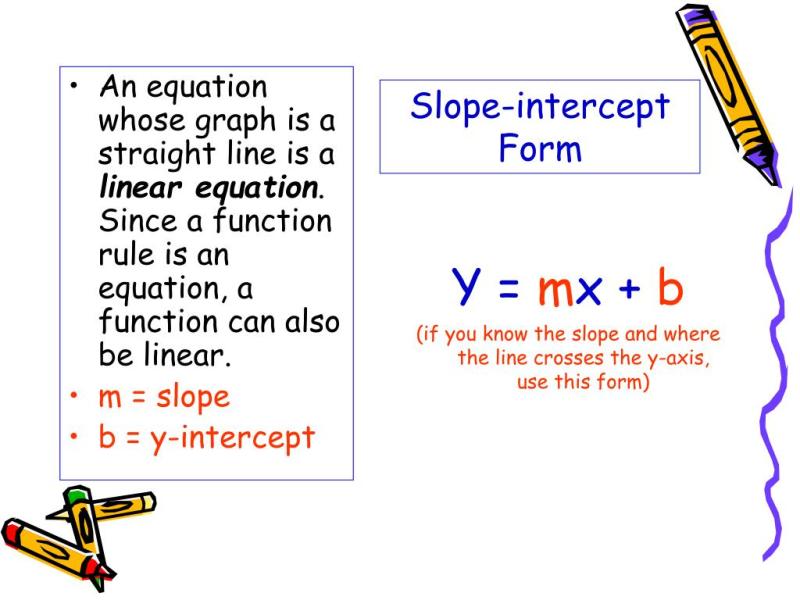
The slope-intercept form of a linear equation is given by:
where:
- is the dependent variable (output),
- is the independent variable (input),
- is the slope of the line,
- is the y-intercept, which is the value of when is 0.
Here's an example of an equation in slope-intercept form:
In this equation:
- The slope () is 2.
- The y-intercept () is 3.
This equation represents a line in the Cartesian coordinate system with a slope of 2 and a y-intercept at the point (0, 3). You can interpret this as follows:
- The slope of 2 means that for every one-unit increase in , will increase by 2 units.
- The y-intercept of 3 means that the line crosses the y-axis at the point (0, 3).
You can use this equation to find the value of for a given or to graph the line on a coordinate plane.
For example, if , you can substitute into the equation:
So, when , is 5. This point (1, 5) lies on the graph of the line represented by the equation .
Mathematical representation: What is an example of slope-intercept form?
In mathematics, the slope-intercept form is a way of representing the equation of a straight line. It is expressed as:
y = mx + b
where:
- y is the y-coordinate of any point on the line
- x is the x-coordinate of any point on the line
- m is the slope of the line, which represents the steepness of the line
- b is the y-intercept, which represents the point where the line crosses the y-axis
Illustrating the slope-intercept form with a concrete mathematical example
Consider the equation:
y = 2x + 3
In this equation, m = 2 and b = 3. This means that the line has a slope of 2, which indicates that it rises 2 units for every 1 unit it moves horizontally. The line also intersects the y-axis at the point (0, 3), which is represented by the y-intercept of 3.
Tips for students in understanding and using the slope-intercept form in linear equations
Here are some tips for students in understanding and using the slope-intercept form in linear equations:
Remember the meaning of slope and y-intercept. The slope represents the steepness of the line, while the y-intercept represents the point where the line crosses the y-axis.
Use the slope to determine the direction of the line. A positive slope indicates a line that rises from left to right, while a negative slope indicates a line that falls from left to right. A slope of 0 represents a horizontal line, and a slope that is undefined represents a vertical line.
Use the y-intercept to find a point on the line. The y-intercept is the point where the line crosses the y-axis, so it represents the coordinates (0, b).
Practice graphing lines using the slope-intercept form. Plotting points and understanding the relationship between the slope and the y-intercept will help you visualize and understand linear equations.
The slope-intercept form is a fundamental concept in linear algebra and is widely used in various fields, including mathematics, physics, engineering, and economics. By understanding and using the slope-intercept form, students can effectively analyze and represent linear relationships between variables.