Explaining Negative Times Negative: Understanding the Positive Result

_{January 19, 2024 by JoyAnswer.org, Category : Mathematics}

Why does negative times a negative equal a positive? Explore why negative times negative equals a positive result in mathematics. This article provides an explanation of the rules governing the multiplication of negative numbers.

Why does negative times a negative equal a positive?

The rule that a negative times a negative equals a positive is a fundamental concept in arithmetic and algebra. It might seem counterintuitive at first, but it can be explained using various approaches. One common explanation involves considering multiplication in terms of repeated addition.

Explanation:

Positive Times Positive:
- When you multiply two positive numbers, you are essentially adding one of the numbers to itself repeatedly. For example, $3 \times 4$ means adding $3$ four times: $3 + 3 + 3 + 3 = 12$ .
Negative Times Positive:
- If you multiply a negative number by a positive number, it's like adding the negative number to itself repeatedly. For example, $(-3) \times 4$ means adding $-3$ four times: $(-3) + (-3) + (-3) + (-3) = -12$ .
Negative Times Negative:
- Now, when you multiply a negative number by a negative number, you are essentially adding the opposite of the negative number to itself repeatedly. For example, $(-3) \times (-4)$ means adding the opposite of $-3$ (which is $3$ ) four times: $3 + 3 + 3 + 3 = 12$ .

So, the rule emerges from the idea that multiplying by a negative number is like repeatedly adding the opposite of that number. When you do this twice (as in negative times negative), you end up with a positive result.

This rule is consistent with the properties of numbers and helps maintain mathematical consistency and coherence in various algebraic expressions and equations.

Why does negative times negative equal positive?

The idea that a negative times a negative equals a positive can seem counterintuitive at first. But there's a perfectly logical explanation behind it!

Multiplication is essentially repeated addition. So, what if we think of multiplying a negative number (-a) by another negative number (-b) as subtracting -b a total of -a times?

For example, -2 * -3 = 6. We can think of this as subtracting -3 from -2 two times. The first time, we have -5. The second time, we have -2. So, the answer is 6.

Here's another way to think about it. Imagine you have -a apples. You owe someone a certain number of apples. Now, let's say you subtract this quantity of apples a total of -b times. Here, the negative sign of -b indicates you're actually getting apples back (subtracting a negative is like adding).

So, if you take away -3 apples a total of -2 times, you end up with a positive 6 apples!

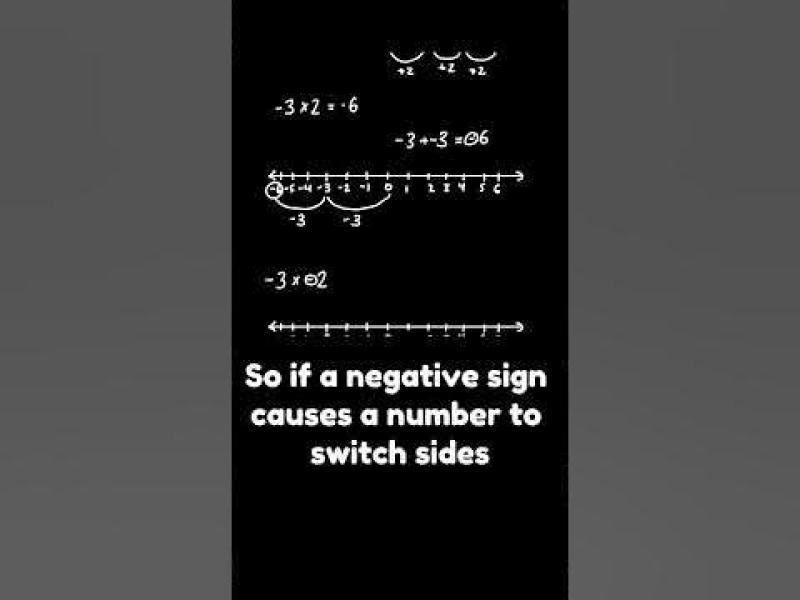
Visualizing it on a number line can also help. A number line is a horizontal line with zero in the middle. Positive numbers are to the right of zero, and negative numbers are to the left of zero.

Multiplication by a negative number can be visualized as jumping -a steps to the left (subtracting -a). So, multiplying -2 by -3 is like jumping -2 steps to the left, which is -5.

Now, multiplying another negative number (-b) by the result is like jumping -b steps further to the left. But remember, subtracting a negative is like adding! So, it's like taking -b steps to the right.

This double "leftward" movement (adding two negatives) actually ends up taking you to the right side of the number line, resulting in a positive product!

In conclusion, negative times negative equals positive because it's essentially the same as adding positive numbers together.

I hope this explanation helps demystify the seemingly paradoxical rule of negative times negative equals positive!