Calculating Velocity Vectors: Methods and Formulas

_{November 6, 2023 by JoyAnswer.org, Category : Physics}

How to calculate velocity vector? Understand how to calculate velocity vectors in physics using specific methods and formulas to represent motion accurately.

Table of Contents

How to calculate velocity vector?

To calculate a velocity vector, you need to determine both the magnitude (speed) and direction of the velocity. The velocity vector is a vector that represents the motion of an object in a specific direction. Here are the methods and formulas for calculating velocity vectors:

1. Magnitude of Velocity (Speed):The magnitude of velocity represents the object's speed and can be calculated using the formula:

Magnitude of Velocity (V) = Total Distance Traveled (D) / Time Interval (Δt)

Where:

V is the magnitude of the velocity vector.
D is the total distance traveled by the object.
Δt is the time interval during which the motion occurred.

2. Direction of Velocity:The direction of velocity indicates the object's path or trajectory. It can be represented using angles or vector notations. To calculate the direction, you'll often need to use trigonometric functions if the motion is not along one of the cardinal directions (e.g., north, east, etc.). Some common methods include:

Using Angles: Measure the angle between the velocity vector and a reference direction, such as north or east.
Vector Notation: Represent the direction using vector components. If you're working in a coordinate system (e.g., Cartesian coordinates), the direction can be specified by the ratios of the vector components. For example, if you have a velocity vector V with components V_x and V_y in the x and y directions, respectively, you can calculate the direction angle (θ) using the following formula:
θ = arctan(V_y / V_x)

3. Vector Representation:Once you have calculated the magnitude and direction of the velocity, you can represent it as a velocity vector. In two dimensions (x and y), a velocity vector can be represented as:

Velocity Vector (V) = V_x * i + V_y * j

Where:

V is the velocity vector.
V_x is the magnitude of the velocity in the x-direction.
V_y is the magnitude of the velocity in the y-direction.
"i" and "j" are unit vectors representing the x and y directions, respectively.

4. Combining Magnitude and Direction:To calculate the velocity vector, you combine the magnitude and direction:

Velocity Vector (V) = V * cos(θ) * i + V * sin(θ) * j

Where:

V is the magnitude of velocity.
θ is the angle of direction measured from the reference direction.
"i" and "j" are unit vectors.

It's important to note that the velocity vector describes not only the magnitude and direction of motion but also the change in position over time. Calculating velocity vectors is a fundamental concept in physics and engineering, especially when dealing with objects moving in multiple directions or undergoing complex motions. It allows for a comprehensive understanding of an object's motion in both speed and direction.

Calculating Velocity Vector: Techniques and Applications

Velocity vector, a crucial concept in kinematics, represents the rate of change of an object's position, encompassing both magnitude (speed) and direction. Calculating velocity vectors is essential for analyzing motion, solving physics problems, and understanding various real-world phenomena.

Techniques for Calculating Velocity Vectors:

Instantaneous Velocity:

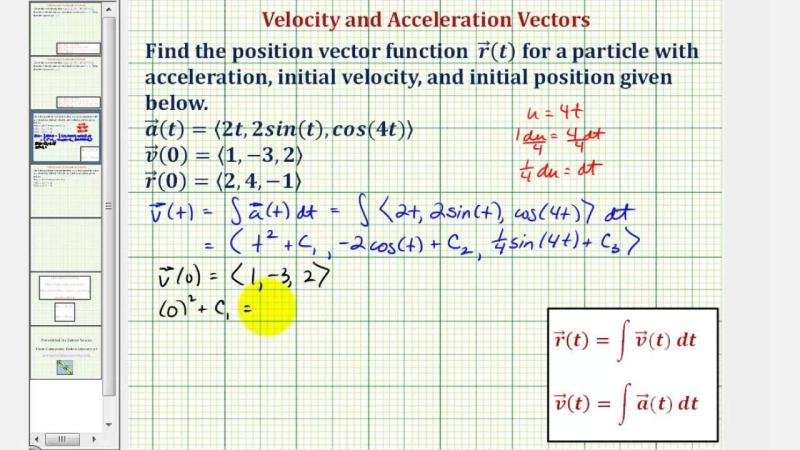
Instantaneous velocity represents the object's velocity at a specific moment in time. It is calculated using the derivative of the position vector with respect to time:

Instantaneous velocity = v(t) = dr(t)/dt

where:

v(t) is the instantaneous velocity vector at time t
r(t) is the position vector at time t

Average Velocity:

Average velocity represents the overall rate and direction of an object's displacement over a specified time interval:

Average velocity = Δr / Δt

where:

Δr is the displacement vector, representing the change in position of the object
Δt is the time interval over which the displacement occurs

Applications of Velocity Vector Calculations:

Motion Analysis: Velocity vectors are used to analyze the motion of objects, including their speed, direction, and acceleration.
Physics Problems: Velocity vectors are essential for solving various physics problems, such as determining the distance traveled, the time required to reach a specific location, or the forces acting on an object.
Real-World Applications: Velocity vector calculations have applications in various fields, including engineering, aviation, robotics, and sports performance analysis.

Velocity Vector Computation: A Comprehensive Approach

Calculating velocity vectors involves understanding the concept of vectors and applying appropriate mathematical techniques.

Position Vectors:

Position vectors represent an object's location in space relative to a chosen reference point. They are typically expressed as components in two or three dimensions.

Displacement Vectors:

Displacement vectors represent the change in position of an object from its initial to its final location. They are calculated by subtracting the initial position vector from the final position vector.

Time Intervals:

Time intervals represent the duration of the object's motion. They are typically measured in seconds.

How to Determine Velocity Vectors in Different Scenarios

The method for calculating velocity vectors depends on the specific motion scenario.

Constant Velocity Motion:

If an object moves with constant velocity, its instantaneous velocity and average velocity are the same. The velocity vector can be calculated using either method.

Accelerated Motion:

If an object is accelerating, its instantaneous velocity changes over time. The instantaneous velocity at a specific time can be calculated using the derivative of the position vector. The average velocity over a time interval can be calculated using the displacement vector and time interval.

Curvilinear Motion:

If an object moves along a curved path, its velocity vector has both magnitude and direction. The direction of the velocity vector is always tangent to the path of motion.

Conclusion:

Calculating velocity vectors is a fundamental skill in physics and has numerous applications in various fields. By understanding the concept of vectors, applying appropriate mathematical techniques, and considering the specific motion scenario, one can accurately determine velocity vectors and gain insights into the motion of objects.