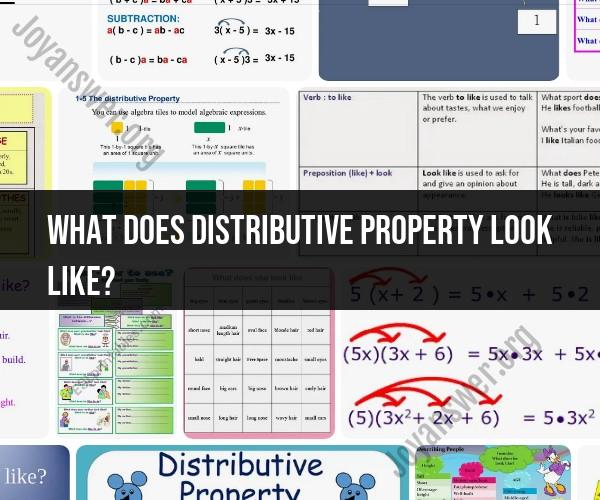
Visualizing the Distributive Property: What It Looks Like

_{October 19, 2023 by JoyAnswer.org, Category : Mathematics}

What does distributive property look like? Gain insight into what the distributive property looks like through visual representations and real-world examples.

Table of Contents

What does distributive property look like?

The distributive property is a fundamental mathematical concept that explains how multiplication interacts with addition or subtraction. While it's an abstract concept, it can be visualized and represented in various ways to make it more tangible. Here are some visual representations of the distributive property:

1. Rectangle Area Model:

One of the most common visualizations of the distributive property is through the use of rectangles to represent numbers. For example, if you have a rectangle that represents the area of (b + c) and you want to multiply it by a, you can break it down into two smaller rectangles: one representing the area of a * b and the other representing the area of a * c. This visually demonstrates the distributive property.

2. Number Line:

You can also use a number line to illustrate the distributive property. For example, if you want to calculate 3 * (2 + 4), you can represent this on a number line. Start at 0, then move 2 units to the right to reach 2. From there, move 4 more units to the right to reach 6. This shows that 3 * (2 + 4) is equivalent to 3 * 2 + 3 * 4, demonstrating the distributive property visually.

3. Arrays and Grids:

Another way to visualize the distributive property is by using arrays or grids. Create a grid where the rows represent one factor, the columns represent the other factor, and each cell represents the product of those factors. For example, if you have 3 rows and (2 + 4) columns, you can distribute the multiplication as 3 * 2 in the first part of the grid and 3 * 4 in the second part, visually demonstrating the distributive property.

4. Algebraic Expression Diagrams:

In algebra, you can use diagrams to represent the distributive property with variables. For example, if you want to illustrate a * (x + y), you can draw a diagram with one box labeled "a," another box labeled "x," and a third box labeled "y." Then, draw arrows to show that "a" is distributed to both "x" and "y."

5. Number Tiles or Counters:

For hands-on learning, you can use physical objects like number tiles or counters. Place tiles or counters to represent the values of the expression, and then demonstrate how the multiplication operation is distributed over the addition or subtraction.

6. Area and Perimeter:

You can relate the distributive property to the area and perimeter of geometric shapes. For example, if you have a rectangle with sides of length (b + c) and a width of a, you can calculate its area by breaking it down into two rectangles, one with sides a and b and the other with sides a and c.

These visual representations help students understand the distributive property by showing how multiplication interacts with addition or subtraction in a concrete and tangible way. Depending on the age and level of the students, choose the visualization method that best suits your teaching goals.

What does the distributive property look like in mathematical expressions?

The distributive property in mathematics can be expressed in the following equation:

a(b + c) = ab + ac

where a, b, and c are any mathematical expressions.

This equation means that when you multiply a number (a) by a sum of two numbers (b and c), you can distribute the multiplication over the addition so that the product is equal to the sum of the products.

Here is an example:

2(3 + 5) = 2 * 3 + 2 * 5

= 6 + 10

= 16

How can you visually recognize the distributive property in equations and calculations?

You can visually recognize the distributive property in equations and calculations by looking for the following patterns:

A multiplication sign followed by a sum or difference
A sum or difference followed by a multiplication sign

For example, the following equations and calculations all demonstrate the distributive property:

2(3 + 5) = 6 + 10 = 16

(x + 2)y = xy + 2y

-5(a - b) = -5a + 5b

Can you provide examples of the distributive property in action?

Here are some examples of the distributive property in action:

A farmer has 20 cows and 15 pigs. He wants to know how many animals he has in total. He can use the distributive property to solve this problem:

Total animals = cows + pigs
= 20 cows + 15 pigs
= (20 + 15) * 1
= 35 * 1
= 35

A student is multiplying a trinomial by a monomial. She can use the distributive property to distribute the multiplication over the trinomial:

(x + 2)(x + 3) = x(x + 3) + 2(x + 3)
= x^2 + 3x + 2x + 6
= x^2 + 5x + 6

What are the different forms and representations of the distributive property?

The distributive property can be represented in a variety of ways, including:

Algebraic form: a(b + c) = ab + ac
Verbal form: When you multiply a number by a sum, the product is equal to the sum of the products.
Visual form: A rectangle that is divided into two smaller rectangles, with the multiplication sign in the center.

The distributive property can also be represented using concrete objects, such as blocks or marbles.

How to develop a strong visual understanding of the distributive property in mathematics?

Here are some tips for developing a strong visual understanding of the distributive property in mathematics:

Use diagrams and illustrations. Drawing diagrams and illustrations can help you to visualize the distributive property in action.
Use concrete objects. Using concrete objects, such as blocks or marbles, can help you to understand the distributive property in a more hands-on way.
Look for patterns in the distributive property. There are many patterns in the distributive property that can help you to understand it better. For example, you can notice that the distributive property is always true, regardless of the numbers involved.

By following these tips, you can develop a strong visual understanding of the distributive property in mathematics.

Here is an additional tip:

Relate the distributive property to real-world problems. This can help you to see how the distributive property is used in everyday life. For example, you can use the distributive property to calculate the total cost of items in a grocery cart or the total distance traveled in a car trip.