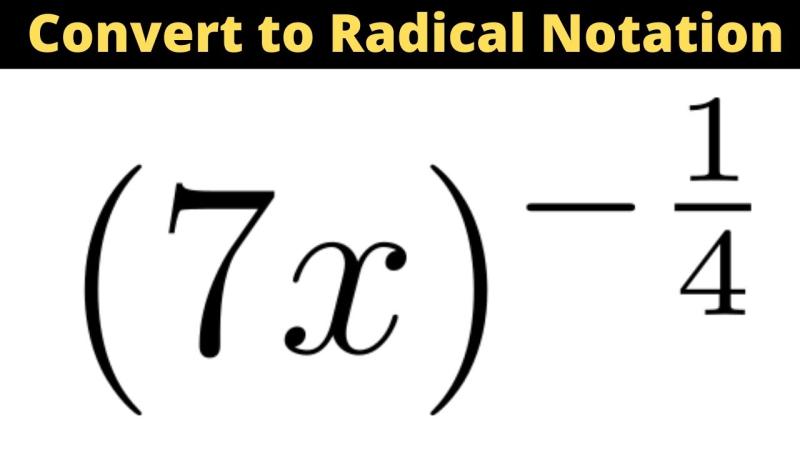
Exponents to Radical Form: Mathematical Representation

_{December 7, 2023 by JoyAnswer.org, Category : Mathematics}

How do you write exponents in radical form? Learn how to express exponents using radical notation. Explore methods to represent exponentials in radical form.

How do you write exponents in radical form?

Exponents can be expressed in radical form through a process called radical notation or using roots. The relationship between exponents and radicals is as follows:

Converting Exponents to Radicals:
- To convert an exponent to radical form, consider the expression $a^{\frac{m}{n}}$ .
- The numerator $m$ represents the power or exponent, and the denominator $n$ indicates the root.
Expressing in Radical Form:
- For positive integer exponents:
  - An expression like $a^{\frac{1}{n}}$ represents the $n$ th root of $a$ , written as $\sqrt[n]{a}$ .
  - For example, $x^{\frac{1}{2}}$ is equivalent to $\sqrt{x}$ , representing the square root of $x$ .
  - $x^{\frac{1}{3}}$ is equivalent to $\sqrt[3]{x}$ , representing the cube root of $x$ .
- For rational exponents:
  - An expression like $a^{\frac{m}{n}}$ represents the $n$ th root of $a$ raised to the power of $m$ , written as $\sqrt[n]{a^m}$ .
  - For example, $x^{\frac{2}{3}}$ is equivalent to $\sqrt[3]{x^2}$ , which represents the cube root of $x$ squared.

Here are a few examples to illustrate:

$x^{\frac{3}{2}}$ can be written as $\sqrt{x^3}$ , representing the square root of $x$ cubed.
$y^{\frac{4}{5}}$ is equivalent to $\sqrt[5]{y^4}$ , representing the fifth root of $y$ raised to the power of 4.

Remember, expressing exponents in radical form can help simplify expressions, especially when dealing with roots and fractional exponents.

How can exponents be expressed in radical form?

Converting exponents to radical form involves representing a power as a root. Here are the steps and rules to follow:

1. Identify the expression: Look for any expressions with a variable raised to an exponent.2. Determine the base and the exponent: Identify the variable (base) and the number raised to the power (exponent).3. Find the corresponding root: The root index corresponds to the exponent. For example, if the exponent is 2, the root is the square root (√), and if the exponent is 3, the root is the cube root (∛).4. Express the power as a root: Replace the exponent with the corresponding root symbol and enclose the base within the radical symbol.

For example:

x^2 becomes √x (square root of x)

y^3 becomes ∛y (cube root of y)

(a^4)^1/2 becomes √(a^4) (square root of a raised to the power of 4)

Additional rules:

x^n/m becomes n√(x^m) (nth root of x raised to the power of m)

(x^n)^(1/n) becomes x (any number raised to its own root equals itself)

Remember:

This conversion is only possible for positive integer exponents.

Fractional and negative exponents require different approaches to express them in radical form.

For more complex expressions with combined operations, follow the order of operations (PEMDAS) to simplify the expression before converting exponents to radical form.