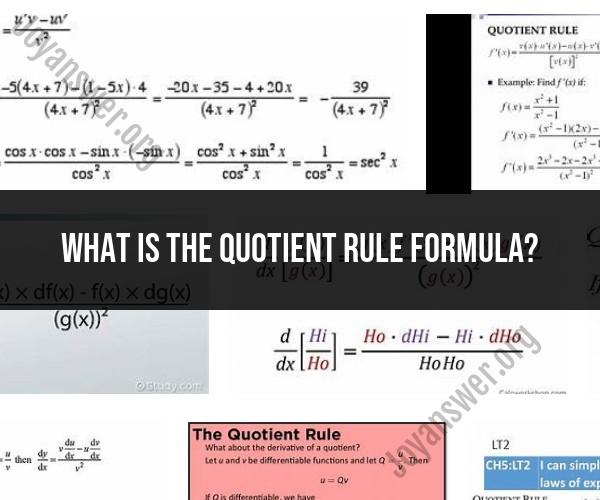
What is the quotient rule formula?
The quotient rule is a fundamental concept in calculus that allows us to find the derivative of a function that is a quotient of two functions. It provides a systematic approach for differentiating such functions. Let's unveil the quotient rule formula and explore how it works through examples:
The Quotient Rule Formula
The quotient rule states that if you have a function \( f(x) \) that can be expressed as the quotient of two functions \( u(x) \) and \( v(x) \), then its derivative \( f'(x) \) can be found using the following formula:
\( f'(x) = \frac{u'(x)v(x) - u(x)v'(x)}{(v(x))^2} \)
Applying the Quotient Rule
To apply the quotient rule, follow these steps:
- Identify \( u(x) \) and \( v(x) \).
- Find the derivatives \( u'(x) \) and \( v'(x) \).
- Substitute the values into the quotient rule formula.
- Simplify the expression if possible.
Example
Let's use the quotient rule to find the derivative of \( f(x) = \frac{x^2 + 2x}{x^3 + 1} \).
Step 1: Identify \( u(x) = x^2 + 2x \) and \( v(x) = x^3 + 1 \).
Step 2: Find \( u'(x) \) and \( v'(x) \):
\( u'(x) = 2x + 2 \)
\( v'(x) = 3x^2 \)
Step 3: Apply the quotient rule formula:
\( f'(x) = \frac{(2x + 2)(x^3 + 1) - (x^2 + 2x)(3x^2)}{(x^3 + 1)^2} \)
Step 4: Simplify the expression as needed.
Conclusion
The quotient rule formula is a powerful tool for finding the derivative of functions that are quotients of other functions. By following the steps and applying the formula, you can efficiently calculate the derivative and better understand the behavior of these types of functions in calculus.