Mastering Two-Step Inequalities: Problem-Solving Techniques

How do you solve two step inequalities?

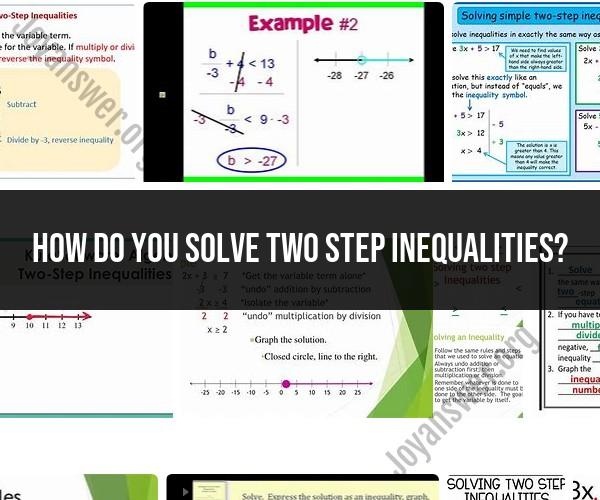
Solving two-step inequalities is a fundamental concept in algebra, and it involves similar principles to solving two-step equations. Here are the steps to solve a two-step inequality:

1. Isolate the Variable Term:Start by isolating the variable term on one side of the inequality, just as you would in an equation. To do this, perform inverse operations in the reverse order of the operations used in the inequality.

   For example, if you have the inequality:$2x + 3 ≤ 7$You should first subtract 3 from both sides:$2x ≤ 7 - 3$$2x ≤ 4$

2. Divide (or Multiply) by the Coefficient of the Variable:After isolating the variable term, you need to get the variable by itself. If the coefficient of the variable is positive, divide both sides of the inequality by that coefficient. If the coefficient is negative, multiply both sides by the negative coefficient.

   In the example above, the coefficient of x is 2 (positive), so you should divide both sides by 2:$\frac{2x}{2} ≤ \frac{4}{2}$$x ≤ 2$

3. Check the Direction of the Inequality:Remember to consider the direction of the inequality symbol. If you multiply or divide both sides by a negative number, you should reverse the direction of the inequality. In this case, since you divided by a positive 2, the direction remains the same (≤).

4. Simplify and Write the Solution:Simplify the expression on the right-hand side of the inequality, if necessary. In this case, 4 ÷ 2 equals 2. So, the solution to the inequality is:$x ≤ 2$

Now, let's consider a slightly different example with a negative coefficient:

Example:$-3y + 5 > 11$

1. Subtract 5 from both sides:$-3y > 11 - 5$$-3y > 6$

2. Divide both sides by -3 (remember to reverse the inequality direction since you're dividing by a negative number):$\frac{-3y}{-3} < \frac{6}{-3}$$y < -2$

So, the solution to this inequality is:$y < -2$

Always remember to pay attention to the direction of the inequality and perform the necessary operations to isolate the variable. These steps should help you solve two-step inequalities effectively.