Generating Irrational Numbers with a Calculator: Myth or Reality?

_{November 13, 2023 by JoyAnswer.org, Category : Mathematics}

Can I get an irrational number by using a calculator? Explore the limitations of calculators in generating irrational numbers. This guide provides insights into the mathematical nature of irrational numbers and whether they can be accurately obtained using calculators.

Table of Contents

Can I get an irrational number by using a calculator?

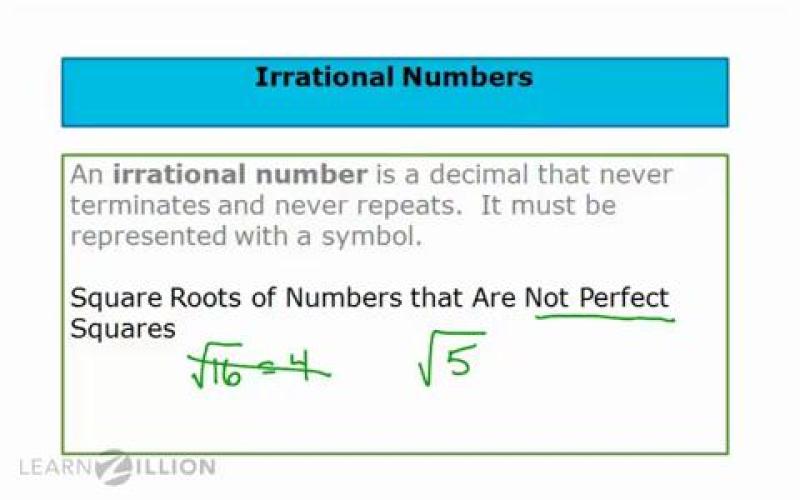
Irrational numbers are mathematical constants that cannot be expressed as fractions of two integers and have non-repeating, non-terminating decimal expansions. Examples of irrational numbers include the square root of 2 (√2), pi (π), and the golden ratio (φ).

When you use a calculator to find the square root of a number, such as √2, you will get an approximate decimal representation. The calculator will display as many decimal places as its precision allows. However, it's important to note that calculators, being finite machines, cannot truly represent irrational numbers in their entirety.

For example, if you calculate the square root of 2 on a standard calculator, you might see something like 1.41421356. This is an approximation, and the decimal representation will continue indefinitely without repeating, as √2 is irrational.

So, while a calculator can provide you with a numerical approximation of an irrational number, it cannot give you the exact, infinite decimal expansion. The true nature of irrational numbers lies in their inability to be expressed as a finite decimal or a fraction. If you were to write out the decimal expansion of an irrational number manually, you would never reach the end, and the digits would not repeat.

In summary, calculators can generate decimal approximations of irrational numbers, but the true nature of irrationality, being non-repeating and non-terminating, cannot be fully captured in a finite decimal representation.

Calculating irrationality: Can I get an irrational number by using a calculator?

No, you cannot get an irrational number by using a calculator. Calculators are limited to representing rational numbers, which are numbers that can be expressed as a fraction of two integers. Irrational numbers, on the other hand, cannot be expressed as a fraction of two integers.

Exploring the limitations and capabilities of calculators in generating irrational numbers

Calculators can be used to approximate irrational numbers, but they cannot represent them exactly. For example, you can use a calculator to approximate the value of pi, but you cannot type in the exact value of pi.

Calculators are limited by the number of digits that they can display. For example, a calculator that can display 10 digits can only approximate irrational numbers to 10 decimal places.

Insights into mathematical principles and the representation of irrational numbers

Irrational numbers are numbers that cannot be expressed as a fraction of two integers. They are also non-terminating and non-repeating decimals. Some examples of irrational numbers include pi, the square root of 2, and the golden ratio.

Irrational numbers are important in mathematics because they have many applications in geometry, trigonometry, and calculus. For example, pi is used to calculate the circumference and area of a circle, and the square root of 2 is used to calculate the length of the diagonal of a square.

Irrational numbers can be represented in a variety of ways, including:

Decimals: Irrational numbers can be approximated as decimals, but they cannot be represented exactly. For example, pi can be approximated as 3.141592653589793, but this is not the exact value of pi.
Fractions: Irrational numbers can also be represented as fractions, but these fractions are typically very complex and difficult to work with. For example, pi can be represented as the fraction 22/7, but this fraction is only an approximation of pi.
Continued fractions: Continued fractions are a way of representing irrational numbers as a series of rational numbers. Continued fractions are often more accurate than decimals and fractions, but they can be difficult to work with.
Infinite series: Infinite series are another way of representing irrational numbers. Infinite series are typically more accurate than decimals, fractions, and continued fractions, but they can be even more difficult to work with.

Conclusion

Calculators are useful tools, but they have limitations. Calculators cannot represent irrational numbers exactly, but they can be used to approximate them to a high degree of accuracy.