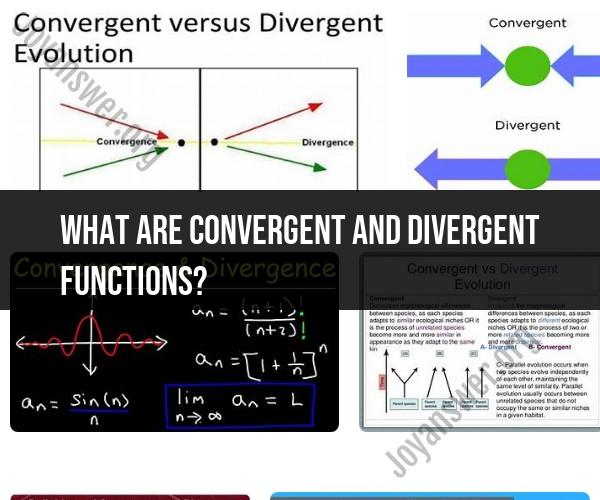
What are convergent and divergent functions?
In mathematics, "convergent" and "divergent" are terms used to describe the behavior of sequences and series, particularly in the context of limits and sums. These terms help mathematicians understand whether a sequence or series approaches a particular value (convergent) or does not (divergent). Here's what each term means:
Convergent:
- A sequence or series is said to be "convergent" if it approaches a finite limit as the number of terms increases without bound.
- For a sequence, this means that as you go further along in the sequence (i.e., as you consider more terms), the terms get closer and closer to a specific value.
- For a series, this means that as you add more terms of the series together, the sum approaches a specific finite value.
Example 1 (Sequence): The sequence is convergent because as you go further along, the terms get closer to zero.
Example 2 (Series): The series (a geometric series) is convergent because the sum approaches 2.
Divergent:
- A sequence or series is said to be "divergent" if it does not approach a finite limit as the number of terms increases without bound.
- In a divergent sequence, the terms may become increasingly large in magnitude without approaching a specific value.
- In a divergent series, the sum may become infinitely large (positive or negative) or oscillate without settling on a finite value.
Example 3 (Sequence): The sequence is divergent because the terms keep increasing without limit.
Example 4 (Series): The series (an infinite sum of ones) is divergent because the sum goes to infinity.
Convergence and divergence are fundamental concepts in calculus and real analysis, and they play a crucial role in determining whether certain mathematical operations are valid. Mathematicians use various tests and criteria to establish the convergence or divergence of sequences and series, such as the limit comparison test, ratio test, integral test, and more. These concepts are also essential in understanding the behavior of functions and their limits as they approach specific values or infinity.
Understanding Mathematical Functions: Convergent and Divergent Functions Explained
A mathematical function is a relationship between two sets of numbers, called the domain and the range. The domain of a function is the set of all input values, and the range of a function is the set of all output values.
A function can be convergent or divergent. A convergent function is a function whose output values approach a specific limit as the input values approach a specific value. A divergent function is a function whose output values do not approach a specific limit as the input values approach a specific value.
Convergence and Divergence in Calculus: Differentiating Between Function Behavior
In calculus, convergence and divergence are studied in the context of limits. A limit is a value that a function approaches as its input values approach a specific value.
A function is convergent at a point if its limit at that point exists. A function is divergent at a point if its limit at that point does not exist, or if the limit is equal to positive or negative infinity.
Mathematical Behavior: Analyzing Convergent and Divergent Functions
The behavior of convergent and divergent functions can be analyzed using a variety of mathematical techniques. One common technique is to use graphical analysis.
To analyze the behavior of a function graphically, you can plot the function on a coordinate plane. The plot of a convergent function will typically approach a specific point, called the limit point, as the input values approach a specific value. The plot of a divergent function will typically diverge to infinity or negative infinity as the input values approach a specific value.
Another common technique for analyzing the behavior of convergent and divergent functions is to use analytical analysis. Analytical analysis involves using mathematical formulas and equations to study the behavior of functions.
For example, you can use the derivative of a function to analyze its behavior at a specific point. The derivative of a function at a point gives you the slope of the line tangent to the function's graph at that point. If the derivative of a function at a point is equal to zero, then the function is at an extremum point (a maximum or minimum point). If the derivative of a function at a point is positive, then the function is increasing at that point. If the derivative of a function at a point is negative, then the function is decreasing at that point.
You can also use the integral of a function to analyze its behavior between two points. The integral of a function over an interval gives you the area under the function's graph over that interval. If the integral of a function over an interval is positive, then the function is increasing over that interval. If the integral of a function over an interval is negative, then the function is decreasing over that interval.
By using graphical analysis and analytical analysis, you can learn a lot about the behavior of convergent and divergent functions. This knowledge can be used to solve a variety of problems in mathematics and other fields.