What is the definition of complement in math?
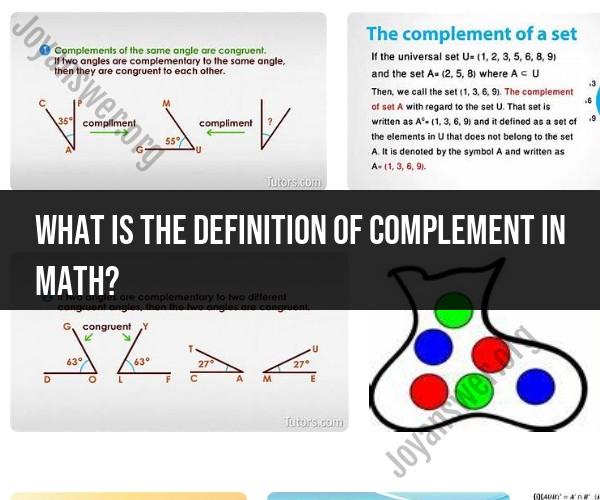
In mathematics, the complement refers to a fundamental concept that is often used in set theory and probability theory. The complement of a set A, denoted as A', is a new set that contains all the elements from a universal set U that are not in set A. In other words, it consists of all the elements in the universal set that do not belong to set A.
Mathematically, you can define the complement of set A as follows:
A' = {x ∈ U : x ∉ A}
Here's a breakdown of the symbols used in this definition:
- A' represents the complement of set A.
- U represents the universal set, which is the set containing all the elements under consideration.
- x is an element.
- ∈ means "is an element of."
- ∉ means "is not an element of."
For example, if we have a universal set U that contains the numbers 1 through 10, and set A is defined as A = {2, 4, 6, 8}, then the complement of set A, denoted as A', would consist of all the numbers from the universal set U that are not in set A. In this case:
A' = {1, 3, 5, 7, 9, 10}
So, the complement of set A contains all the elements of the universal set U that are not part of set A. Complements are a fundamental concept in set theory and are used in various mathematical and statistical applications.
Understanding the Mathematical Concept of Complement
The complement of a set in mathematics is the collection of elements that are not in the set. If a set A is a subset of a universal set U, the complement of A, A', is the set of all elements in U that are not in A. In other words, the complement of A is the set of elements that belong to U but do not belong to A.
The complement of a set can be denoted by A', or sometimes by Ac, where the apostrophe or the letter c indicates the complement operation.
Exploring the Role of Complements in Mathematics
Complements play an important role in many areas of mathematics. For example, they are used in set theory, probability, and logic.
In set theory, complements are used to define other set operations, such as the union and intersection of sets. For example, the union of two sets A and B is the set of all elements that are in A or in B or in both A and B. The intersection of two sets A and B is the set of all elements that are in both A and B.
In probability, complements are used to calculate the probability of events. For example, if the probability of event A happening is P(A), then the probability of event A not happening is 1 - P(A).
In logic, complements are used to define logical operators, such as negation and disjunction. For example, the negation of a statement is the statement that is the opposite of the original statement. The disjunction of two statements is the statement that is true if either statement is true or if both statements are true.
Complement in Math: Definition and Applications
Definition:
The complement of a set A in a universal set U is the set of all elements in U that are not in A. The complement of A is denoted by A' or Ac.
Applications:
Complements are used in a variety of mathematical applications, including:
- Set theory: Complements are used to define other set operations, such as the union and intersection of sets.
- Probability: Complements are used to calculate the probability of events.
- Logic: Complements are used to define logical operators, such as negation and disjunction.
- Computer science: Complements are used in a variety of computer science applications, such as bitwise operations and logic circuits.
Here are some specific examples of how complements are used in mathematics:
- In set theory, the complement of the set of all even numbers is the set of all odd numbers.
- In probability, the complement of the event of getting a head on a coin toss is the event of getting a tail.
- In logic, the complement of the statement "All cats are mammals" is the statement "Some cats are not mammals."
Complements are a powerful tool that can be used to solve a variety of mathematical problems.