Calculating the Magnitude of Resultant Force: Formulas and Steps
October 6, 2023 by JoyAnswer.org, Category : Physics
How to calculate magnitude resultant force? Learn the formulas and steps to calculate the magnitude of the resultant force when multiple forces act on an object.
- 1. How to calculate magnitude resultant force?
- 2. Calculating the Magnitude of a Resultant Force: Step-by-Step
- 3. Finding the Magnitude of Resultant Forces: Mathematical Approach
- 4. Determining the Magnitude of a Resultant Force Vector
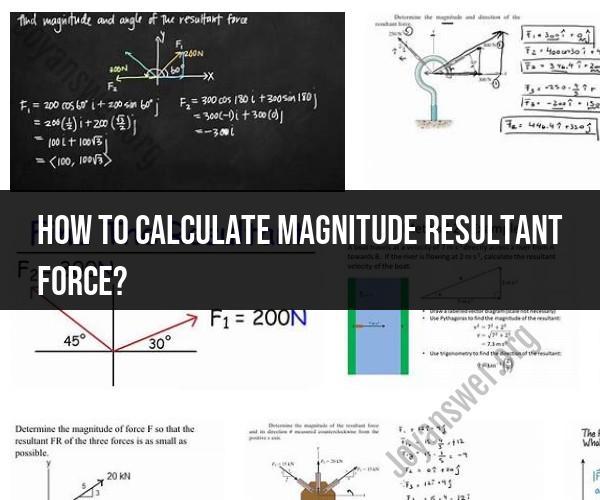
How to calculate magnitude resultant force?
To calculate the magnitude of the resultant force (the overall force) when multiple forces act on an object, you can use vector addition. The magnitude of the resultant force can be calculated using either the graphical method (such as the parallelogram or triangle method) or the trigonometric method. I'll provide you with the steps for both methods:
Method 1: Graphical Method (Parallelogram or Triangle Rule)
Draw a Scale Vector Diagram: Start by drawing a scaled vector diagram. This means representing each force as an arrow with its length proportional to the magnitude of the force and its direction indicating the direction of the force.
Place Vectors Head-to-Tail: Arrange the vectors head-to-tail. This means that you place the tail (starting point) of the second vector at the head (end point) of the first vector, and so on, until you have all the vectors in the diagram.
Complete the Parallelogram or Triangle: If you have two vectors, use the parallelogram rule. If you have three vectors, use the triangle rule. For the parallelogram rule, draw a parallelogram with the two vectors as adjacent sides. For the triangle rule, connect the ends of the vectors to form a triangle.
Find the Resultant Vector: Measure the length of the diagonal of the parallelogram or the third side of the triangle. This represents the magnitude of the resultant force.
Calculate the Magnitude: If you're using a scaled diagram, use the scale to convert the length of the diagonal or third side into the actual magnitude of the resultant force.
Method 2: Trigonometric Method (Component Method)
Resolve Forces into Components: Break down each force into its horizontal (x) and vertical (y) components. To do this, use trigonometry. If you have a force F with an angle θ from the positive x-axis, then the x-component is Fx = F * cos(θ), and the y-component is Fy = F * sin(θ).
Add the Component Forces: Sum up all the x-components and y-components separately to get the total x-component (ΣFx) and total y-component (ΣFy).
Calculate the Resultant Force: Use the Pythagorean theorem to find the magnitude of the resultant force (R):
R = sqrt((ΣFx)^2 + (ΣFy)^2)
Determine the Direction: To find the direction (θ) of the resultant force, use trigonometry:
θ = atan(ΣFy / ΣFx)
These are the steps to calculate the magnitude of the resultant force when multiple forces are involved. Choose the method that suits your problem and the information you have about the forces, and then follow the steps accordingly.
Calculating the Magnitude of a Resultant Force: Step-by-Step
To calculate the magnitude of a resultant force, you can follow these steps:
- Draw a free-body diagram. This is a diagram of the object showing all of the forces acting on it.
- Resolve each force into its components. This means breaking down each force into its horizontal and vertical components. You can use trigonometry to do this.
- Add the horizontal and vertical components of all the forces. This will give you the horizontal and vertical components of the resultant force.
- Use the Pythagorean theorem to calculate the magnitude of the resultant force.
Pythagorean theorem:
a^2 + b^2 = c^2
where:
- a and b are the two legs of a right triangle
- c is the hypotenuse of the right triangle
In this case, the horizontal and vertical components of the resultant force are the two legs of the triangle, and the magnitude of the resultant force is the hypotenuse.
Example:
Two forces, F1 and F2, are acting on an object. F1 is pointing to the right and has a magnitude of 10 N. F2 is pointing upwards and has a magnitude of 5 N.
To calculate the magnitude of the resultant force, we first need to resolve each force into its components.
F1 components:
- Horizontal component: 10 N
- Vertical component: 0 N
F2 components:
- Horizontal component: 0 N
- Vertical component: 5 N
Now, we can add the horizontal and vertical components of all the forces.
Resultant force components:
- Horizontal component: 10 N
- Vertical component: 5 N
Finally, we can use the Pythagorean theorem to calculate the magnitude of the resultant force.
Resultant force magnitude = √(10 N)^2 + (5 N)^2 = √125 N^2 = 11.18 N
Therefore, the magnitude of the resultant force is 11.18 N.
Finding the Magnitude of Resultant Forces: Mathematical Approach
The mathematical approach to finding the magnitude of resultant forces is based on the Pythagorean theorem.
Resultant force magnitude = √(∑Fx)^2 + (∑Fy)^2
where:
- ∑Fx is the sum of the horizontal components of all the forces
- ∑Fy is the sum of the vertical components of all the forces
Example:
Two forces, F1 and F2, are acting on an object. F1 is pointing to the right and has a magnitude of 10 N. F2 is pointing upwards and has a magnitude of 5 N.
To calculate the magnitude of the resultant force, we can use the following equation:
Resultant force magnitude = √(10 N)^2 + (5 N)^2 = √125 N^2 = 11.18 N
Therefore, the magnitude of the resultant force is 11.18 N.
Determining the Magnitude of a Resultant Force Vector
The magnitude of a resultant force vector is the length of the vector. It can be calculated using the Pythagorean theorem, as shown above.
Example:
Two forces, F1 and F2, are acting on an object. F1 is pointing to the right and has a magnitude of 10 N. F2 is pointing upwards and has a magnitude of 5 N.
The resultant force vector can be represented by the following diagram:
F1
/
/
F2
The magnitude of the resultant force vector is equal to the length of the diagonal of the rectangle. We can use the Pythagorean theorem to calculate the length of the diagonal:
Resultant force magnitude = √(10 N)^2 + (5 N)^2 = √125 N^2 = 11.18 N
Therefore, the magnitude of the resultant force vector is 11.18 N.
