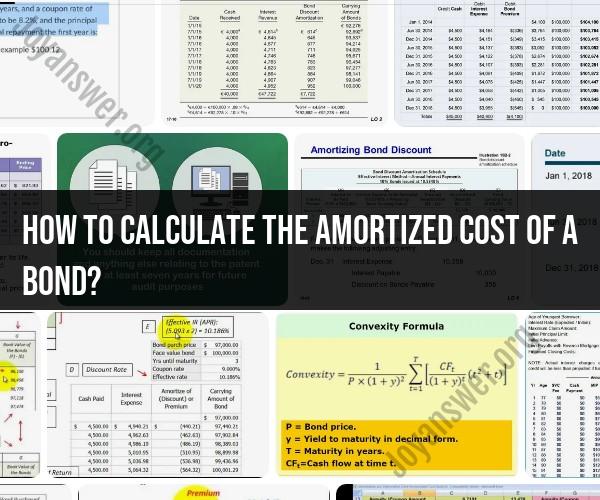
Calculating the Amortized Cost of a Bond

_{November 3, 2023 by JoyAnswer.org, Category : Finance}

How to calculate the amortized cost of a bond? Learn the process of calculating the amortized cost of a bond, a crucial aspect of bond accounting and financial analysis.

Table of Contents

How to calculate the amortized cost of a bond?

The amortized cost of a bond represents the book value or carrying value of the bond on the issuer's balance sheet. It reflects the bond's cost over time as it is gradually paid down or amortized. To calculate the amortized cost of a bond, you can follow these steps:

Start with the bond's initial par value (face value) - This is the amount the bond was issued for.
Adjust for any premium or discount - If the bond was issued at a premium (higher than par) or a discount (lower than par), you need to adjust the initial par value accordingly.
- For a premium bond, subtract the premium amount from the par value.
- For a discount bond, add the discount amount to the par value.
The adjusted value is often called the "carrying amount" or "book value" of the bond.
Add or subtract any accumulated amortization - This is the total amortization expense recorded on the issuer's books up to the present time. For each accounting period, amortization is calculated based on the difference between the carrying amount and the bond's face value, and this amount is added to or subtracted from the carrying amount.
- If amortization expense is added, it reduces the carrying amount.
- If amortization expense is subtracted, it increases the carrying amount.
The result is the amortized cost of the bond - This is the book value of the bond as of the current date.

Here's the formula for calculating the amortized cost of a bond:

Amortized Cost = Initial Par Value ± (Premium or Discount) ± Accumulated Amortization

For example, let's say you have a bond with an initial par value of $1,000, issued at a premium of $50, and you have recorded $20 of accumulated amortization. The amortized cost of the bond would be:

Amortized Cost = $1,000 - $50 + $20 = $970

So, the amortized cost of the bond is $970.

It's important to note that the amortized cost of a bond changes over time as it is amortized, and it is used for accounting purposes to reflect the bond's value on the issuer's balance sheet. This is different from the market price of the bond, which may fluctuate based on market interest rates and other factors.

How to calculate the amortized cost of a bond in finance?

The amortized cost of a bond is the price of a bond that has been adjusted to take into account the premium or discount at which it was purchased and the interest that is earned over the life of the bond. It is calculated using the following formula:

Amortized Cost = Purchase Price + Accrued Interest - Amortized Premium/Discount

Accrued interest is the interest that has accrued on the bond since the last interest payment was made. It is calculated by multiplying the bond's coupon rate by the number of days since the last interest payment was made, divided by the number of days in a year.

Amortized premium/discount is the difference between the bond's purchase price and its face value, spread out over the life of the bond. It is calculated using the following formula:

Amortized Premium/Discount = (Purchase Price - Face Value) / Number of Periods to Maturity

Key components and variables involved in bond amortization calculations

The key components and variables involved in bond amortization calculations are:

Purchase price: The price at which the bond was purchased.
Face value: The principal amount of the bond, which is repaid to the bondholder at maturity.
Coupon rate: The annual interest rate that the bond pays.
Number of periods to maturity: The number of years or months until the bond matures.
Amortized premium/discount: The difference between the bond's purchase price and its face value, spread out over the life of the bond.

The importance of understanding amortized cost for bond investors

Understanding amortized cost is important for bond investors because it helps them to accurately track the performance of their investments. It also allows them to calculate their after-tax returns and to make informed decisions about when to buy and sell bonds.

Examples and step-by-step guides for computing the amortized cost of a bond

Example 1:

An investor purchases a 10-year bond with a face value of $1,000 and a coupon rate of 5% for $950. The bond's amortized cost is calculated as follows:

Amortized Cost = Purchase Price + Accrued Interest - Amortized Premium/Discount

Amortized Cost = $950 + $50 - $50

Amortized Cost = $950

Step-by-step guide for computing the amortized cost of a bond:

Determine the purchase price of the bond.
Calculate the accrued interest.
Calculate the amortized premium/discount.
Add the purchase price and accrued interest and subtract the amortized premium/discount to determine the amortized cost of the bond.

How changes in interest rates affect the amortized cost of bonds

Changes in interest rates can affect the amortized cost of bonds. When interest rates rise, the value of existing bonds falls. This is because investors can buy new bonds with higher coupon rates, making older bonds less attractive. As a result, the amortized cost of existing bonds will decrease.

Conversely, when interest rates fall, the value of existing bonds rises. This is because investors are willing to pay a premium for older bonds with higher coupon rates. As a result, the amortized cost of existing bonds will increase.

It is important to note that the amortized cost of a bond is only one factor to consider when making investment decisions. Investors should also consider the bond's credit quality, yield to maturity, and liquidity.