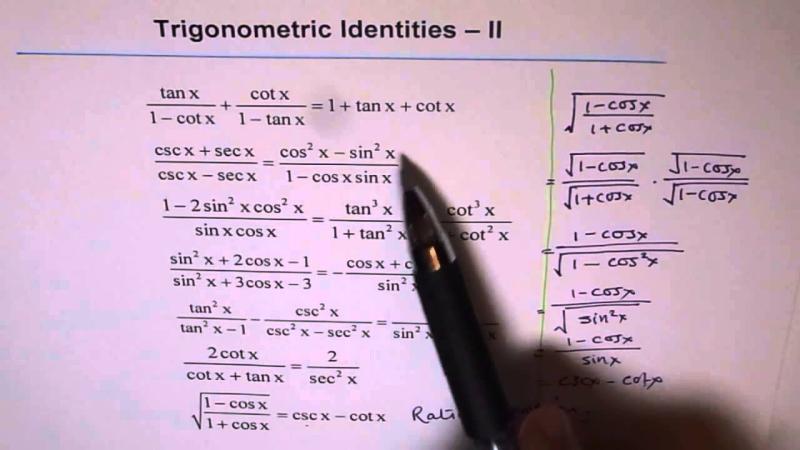
Verifying Trigonometric Identities: Step-by-Step Process

_{November 11, 2023 by JoyAnswer.org, Category : Mathematics}

How to verify trigonometric identities? Discover how to verify trigonometric identities with a step-by-step process. This guide provides insights into the systematic approach for confirming the equality of trigonometric expressions.

Table of Contents

How to verify trigonometric identities?

Verifying trigonometric identities involves confirming that the expression on one side of the equation is equal to the expression on the other side for all valid values of the variables involved. Here is a step-by-step process for verifying trigonometric identities:

General Steps:

Start with the More Complex Side:
- Begin with the side of the equation that appears more complex or has more terms. This is often the side with more trigonometric functions or expressions.
Use Basic Trigonometric Identities:
- Utilize basic trigonometric identities to simplify expressions. Common identities include:
  - Pythagorean Identities: $\sin^2(\theta) + \cos^2(\theta) = 1$
  - Reciprocal Identities: $\csc(\theta) = \frac{1}{\sin(\theta)}$ , $\sec(\theta) = \frac{1}{\cos(\theta)}$ , $\cot(\theta) = \frac{1}{\tan(\theta)}$
  - Quotient Identities: $\tan(\theta) = \frac{\sin(\theta)}{\cos(\theta)}$ , $\cot(\theta) = \frac{\cos(\theta)}{\sin(\theta)}$
Simplify Both Sides:
- Simplify each side of the equation independently using algebraic manipulations and trigonometric identities. Aim to make both sides look as similar as possible.
Manipulate to Match:
- Manipulate one side of the equation to make it look like the other side. Use algebraic manipulations and trigonometric identities to create similarities between the two sides.
Use Fundamental Identities:
- Employ fundamental trigonometric identities like the double-angle, half-angle, and sum/difference identities when necessary to simplify expressions.
Check for Equivalent Forms:
- Utilize trigonometric identities to transform expressions into equivalent forms. This may involve factoring, simplifying fractions, or combining like terms.
Be Aware of Domain Restrictions:
- Be mindful of domain restrictions for trigonometric functions. Some identities may not be valid for all values of the variable, so ensure that the domain is consistent on both sides of the equation.
Verify Equality for All Values:
- Verify that the simplified expressions on both sides of the equation are equal for all values of the variable within the specified domain. This often involves substituting specific values and confirming equality.

Example:

Let's verify the identity $\tan^2(\theta) + 1 = \sec^2(\theta)$ .

Start with the More Complex Side:
- Start with the left side: $\tan^2(\theta) + 1$ .
Use Basic Trigonometric Identities:
- Recall that $\tan^2(\theta) + 1 = \sec^2(\theta)$ is a Pythagorean identity.
Simplify Both Sides:
- The left side becomes $\tan^2(\theta) + 1$ .
Manipulate to Match:
- The right side is $\sec^2(\theta)$ , which is already in a simplified form.
Use Fundamental Identities:
- No additional fundamental identities are needed in this case.
Check for Equivalent Forms:
- The expressions are already equivalent.
Be Aware of Domain Restrictions:
- Ensure that both sides are valid for all values of $\theta$ .
Verify Equality for All Values:
- Substitute specific values of $\theta$ and confirm that both sides are equal.

By following these steps, you can systematically verify trigonometric identities and gain confidence in your understanding of the relationships between trigonometric functions.

How do you go about verifying trigonometric identities?

There are a few different ways to verify trigonometric identities. One common approach is to start with one side of the identity and use various trigonometric identities and algebraic manipulations to transform it into the other side of the identity. If you are able to successfully do this, then you have verified the identity.

Another approach to verifying trigonometric identities is to use graphical methods. This involves graphing both sides of the identity and seeing if they overlap perfectly. If they do, then the identity is true.

Here are some tips for verifying trigonometric identities:

Memorize the fundamental trigonometric identities. This will make it easier to transform expressions and manipulate equations.
Use the Pythagorean identity and the reciprocal identities whenever possible. These identities can be very helpful in simplifying expressions.
Factor expressions and simplify fractions whenever possible. This can make expressions easier to manipulate and can help to reveal hidden trigonometric identities.
Use graphical methods to verify identities, especially if you are stuck. Graphing both sides of an identity can help you to see if the identity is true, even if you cannot prove it algebraically.

What are the common techniques for proving trigonometric equations?

There are a few common techniques for proving trigonometric equations. One common approach is to use trigonometric identities to simplify the equation and then use algebraic methods to solve the equation.

Another approach to proving trigonometric equations is to use graphical methods. This involves graphing both sides of the equation and finding the points of intersection. If the two sides of the equation intersect at the same point, then the equation is true.

Here are some tips for proving trigonometric equations:

Identify the type of equation. Is the equation linear, quadratic, or exponential? Once you know the type of equation, you can use the appropriate algebraic methods to solve the equation.
Use trigonometric identities to simplify the equation. This can make the equation easier to solve.
Factor expressions and simplify fractions whenever possible. This can make expressions easier to manipulate and can help to reveal hidden trigonometric identities.
Use graphical methods to prove equations, especially if you are stuck. Graphing both sides of an equation can help you to see if the equation is true, even if you cannot prove it algebraically.

Are there specific rules or strategies for simplifying trigonometric expressions?

Yes, there are a few specific rules and strategies for simplifying trigonometric expressions. Here are a few tips:

Use the Pythagorean identity and the reciprocal identities whenever possible. These identities can be very helpful in simplifying expressions.
Factor expressions and simplify fractions whenever possible. This can make expressions easier to manipulate and can help to reveal hidden trigonometric identities.
Use the angle addition and subtraction identities to simplify expressions that involve multiple angles.
Use the double angle and half angle identities to simplify expressions that involve powers of trigonometric functions.
Use the trigonometric tables to evaluate trigonometric expressions.

Here are some examples of how to simplify trigonometric expressions:

Expression: sin(θ) + cos(θ)Simplification: √2sin(θ + 45°)

Expression: tan(θ) + sec(θ)Simplification: sec(θ)(tan(θ) + 1)

Expression: sin^2(θ) + cos^2(θ)Simplification: 1

Expression: sin(2θ)Simplification: 2sin(θ)cos(θ)

Expression: tan(θ/2)Simplification: (sin(θ)/(1 + cos(θ)))

By following these tips, you can learn to verify trigonometric identities, prove trigonometric equations, and simplify trigonometric expressions.