Solving Problems of Direct and Inverse Variation: Techniques and Examples

_{December 11, 2023 by JoyAnswer.org, Category : Mathematics}

How to solve direct and inverse variation problems? Explore techniques and examples to solve problems related to direct and inverse variation. This guide provides a clear understanding of these mathematical concepts.

How to solve direct and inverse variation problems?

Solving problems involving direct and inverse variation involves understanding the relationships between variables and using mathematical techniques to find unknown values. Here are explanations and examples for both types of variation:

1. Direct Variation:

In a direct variation, two variables change in such a way that if one variable doubles, the other variable also doubles. The relationship can be expressed as $y = kx$ , where $k$ is the constant of variation.

Steps to Solve Direct Variation Problems:

Identify the Variables:
- Identify the two variables involved in the direct variation problem.
Write the Direct Variation Equation:
- Express the relationship between the variables using the equation $y = kx$ , where $y$ and $x$ are the variables, and $k$ is the constant of variation.
Use Given Information:
- Plug in the given values for one set of variables and solve for the unknown constant ( $k$ ).
Apply the Relationship:
- Once $k$ is determined, use the relationship to solve for other unknowns.

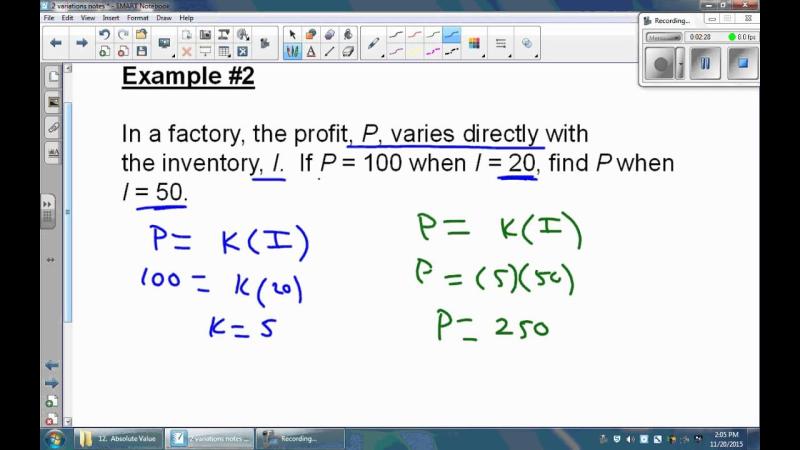
Example:If $y$ varies directly with $x$ , and $y = 15$ when $x = 5$ , find the equation and the value of $y$ when $x = 8$ .

Identify Variables:
- $y$ and $x$
Write the Equation:
- $y = kx$
Use Given Information:
- Plug in $y = 15$ and $x = 5$ : $15 = k \times 5$
Apply the Relationship:
- Solve for $k$ : $k = 3$
- Now that $k = 3$ , use the relationship to find $y$ when $x = 8$ : $y = 3 \times 8 = 24$

2. Inverse Variation:

In an inverse variation, two variables change in such a way that if one variable doubles, the other variable halves. The relationship can be expressed as $y = \frac{k}{x}$ , where $k$ is the constant of variation.

Steps to Solve Inverse Variation Problems:

Identify the Variables:
- Identify the two variables involved in the inverse variation problem.
Write the Inverse Variation Equation:
- Express the relationship between the variables using the equation $y = \frac{k}{x}$ , where $y$ and $x$ are the variables, and $k$ is the constant of variation.
Use Given Information:
- Plug in the given values for one set of variables and solve for the unknown constant ( $k$ ).
Apply the Relationship:
- Once $k$ is determined, use the relationship to solve for other unknowns.

Example:If $y$ varies inversely with $x$ , and $y = 12$ when $x = 4$ , find the equation and the value of $y$ when $x = 6$ .

Identify Variables:
- $y$ and $x$
Write the Equation:
- $y = \frac{k}{x}$
Use Given Information:
- Plug in $y = 12$ and $x = 4$ : $12 = \frac{k}{4}$
Apply the Relationship:
- Solve for $k$ : $k = 48$
- Now that $k = 48$ , use the relationship to find $y$ when $x = 6$ : $y = \frac{48}{6} = 8$

By following these steps, you can solve problems involving direct and inverse variation. The key is to correctly identify the type of variation and set up the appropriate equation.

What methods can be utilized to solve problems involving direct and inverse variation?

Here are some methods to solve problems involving direct and inverse variation:

1. Identify the relationship:

Direct Variation: If two quantities change proportionally in the same direction, they are in direct variation. This can be represented by the formula y = kx, where k is the constant of variation, y is the dependent variable, and x is the independent variable.

Inverse Variation: If two quantities change proportionally in opposite directions, they are in inverse variation. This can be represented by the formula y = k/x, where k is the constant of variation, y is the dependent variable, and x is the independent variable.

2. Find the constant of variation:

In both cases, you need to know the constant of variation k to solve problems. You can find it using one of two ways:
- Given information: If you have information about two corresponding values of the variables, you can substitute them into the formula and solve for k.
- Proportionality statement: Sometimes, the problem might provide a statement about proportional change. This statement can be used to establish an equation and solve for k.

3. Solve for the unknown variable:

Once you have the constant of variation, you can use the appropriate formula (y = kx for direct variation and y = k/x for inverse variation) to solve for the unknown variable.

4. Analyze and interpret the solution:

Check if the solution makes sense in the context of the problem and ensure it adheres to the relationship of direct or inverse variation.

Here are some additional tips for solving problems involving direct and inverse variation:

Pay attention to units of measurement throughout the calculations.

Look for keywords in the problem that indicate direct or inverse variation, such as "proportional," "inversely proportional," "varies directly with," or "varies inversely with."