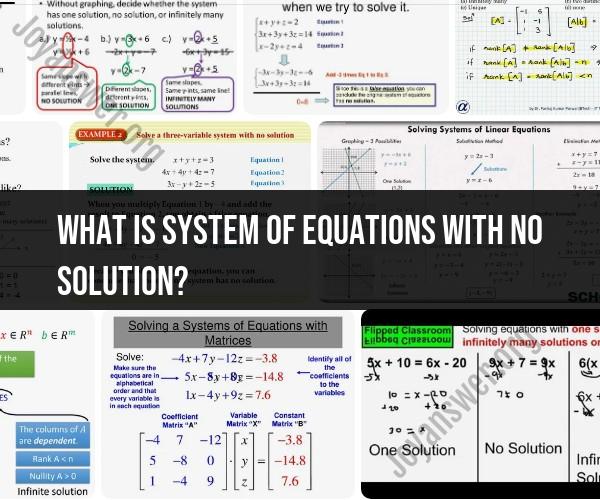
Understanding System of Equations with No Solution

_{October 24, 2023 by JoyAnswer.org, Category : Mathematics}

What is system of equations with no solution? Learn what a system of equations with no solution means and how to recognize and interpret such cases in mathematics.

Table of Contents

What is system of equations with no solution?

A system of equations with no solution is a set of linear equations where there is no common solution that simultaneously satisfies all the equations. In other words, the system is inconsistent, and there are no values for the variables that can make all the equations true at the same time.

Mathematically, a system of linear equations can be represented in the form:

$a₁x + b₁y = c₁$
$a₂x + b₂y = c₂$

If there is no solution to this system, it means that the lines represented by these equations are parallel and do not intersect. In graphical terms, they are two distinct lines that run side by side and never cross each other.

Inconsistent systems typically arise when the equations describe contradictory relationships. For example, consider the following system:

$2x + 3y = 10$
$4x + 6y = 15$

If you attempt to solve this system, you'll find that there are no values of $x$ and $y$ that can simultaneously satisfy both equations. The first equation can be simplified to $2x + 3y = 10$ , and the second equation can be simplified to $2x + 3y = 7$ . Since these equations have different right-hand sides, they cannot represent the same line on the coordinate plane, and there is no solution that makes both equations true.

In summary, a system of equations with no solution occurs when the equations are inconsistent, and there are no values for the variables that can satisfy all the equations simultaneously. These systems often involve equations that represent parallel lines in the coordinate plane or equations that contradict each other mathematically.

System of Equations with No Solution: An Overview

A system of equations with no solution is a system of two or more equations that cannot be solved simultaneously. This means that there is no combination of values for the variables that satisfies all of the equations in the system.

When and Why Systems of Equations Lead to No Solution

There are two main reasons why a system of equations may have no solution:

The two equations are parallel. This means that the two equations have the same slope but different y-intercepts. Parallel lines never intersect, so there is no point of intersection where both equations are satisfied.
The two equations are opposites. This means that the two equations have the same slope and y-intercept. This means that the two equations are essentially the same equation, and there are infinitely many solutions to the system. However, we are only considering systems with a single solution, so a system with two opposite equations is considered to have no solution.

Identifying Inconsistent Systems and Their Characteristics

There are a few ways to identify an inconsistent system of equations:

Look at the slopes of the equations. If the two equations have the same slope but different y-intercepts, then the system is inconsistent.
Try solving the system using elimination. If you eliminate one of the variables and end up with a contradiction (such as 0 = 1), then the system is inconsistent.
Look at the graphical representation of the equations. If the two lines are parallel, then the system is inconsistent.

Strategies for Handling Systems of Equations with No Solution

If you are faced with a system of equations with no solution, there are a few things you can do:

Identify the type of inconsistency. Is the system inconsistent because the equations are parallel or because the equations are opposites?
If the system is inconsistent because the equations are parallel, then you can simply state that the system has no solution.
If the system is inconsistent because the equations are opposites, then you can state that the system has infinitely many solutions.

Theoretical and Practical Implications of No Solution Systems

No solution systems have both theoretical and practical implications.

On a theoretical level, no solution systems show that not all systems of equations can be solved. This is an important mathematical concept that has implications for many different areas of mathematics, such as linear algebra and calculus.

On a practical level, no solution systems can arise in a variety of real-world applications. For example, if you are trying to solve a system of equations to find the optimal solution to a problem, and the system has no solution, then it means that there is no optimal solution to the problem. This can be a valuable insight, as it can help you to avoid wasting time and resources trying to find a solution that does not exist.

Here are some examples of systems of equations with no solution: