Trigonometry Identity: Exploring Fundamental Mathematical Relationships

_{November 11, 2023 by JoyAnswer.org, Category : Mathematics}

What is trigonometry identity? Understand what a trigonometry identity is and explore the fundamental mathematical relationships within this branch of mathematics. This guide provides insights into the significance and application of trigonometry identities.

Table of Contents

What is trigonometry identity?

In trigonometry, an identity is an equation that is true for all values of the variables within its domain. Trigonometric identities involve the six trigonometric functions: sine (sin), cosine (cos), tangent (tan), cosecant (csc), secant (sec), and cotangent (cot).

There are three types of trigonometric identities:

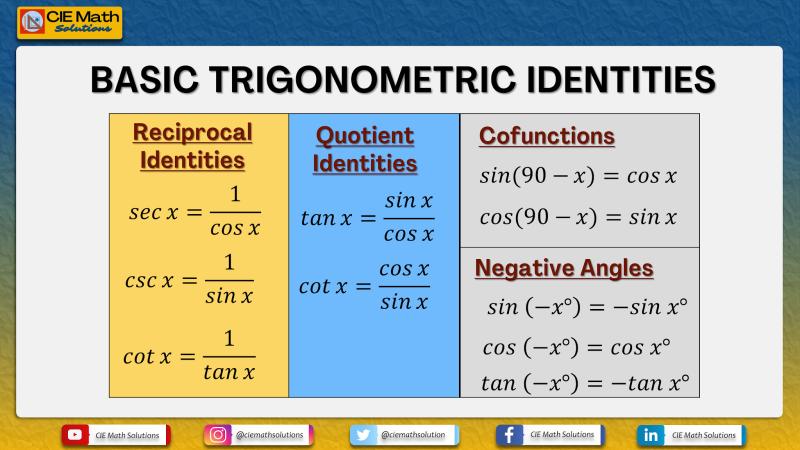
Reciprocal Identities:
- These involve the reciprocal functions. For any angle $\theta$ where the expressions are defined:
  - $\csc(\theta) = \frac{1}{\sin(\theta)}$
  - $\sec(\theta) = \frac{1}{\cos(\theta)}$
  - $\cot(\theta) = \frac{1}{\tan(\theta)}$
Quotient Identities:
- These involve the ratios of the trigonometric functions. For any angle $\theta$ where the expressions are defined:
  - $\tan(\theta) = \frac{\sin(\theta)}{\cos(\theta)}$
  - $\cot(\theta) = \frac{\cos(\theta)}{\sin(\theta)}$
Pythagorean Identities:
- These are derived from the Pythagorean Theorem and are some of the most fundamental trigonometric identities. For any angle $\theta$ where the expressions are defined:
  - $\sin^2(\theta) + \cos^2(\theta) = 1$
  - $1 + \tan^2(\theta) = \sec^2(\theta)$
  - $1 + \cot^2(\theta) = \csc^2(\theta)$

Trigonometric identities are fundamental tools in solving trigonometric equations, simplifying expressions, and proving mathematical relationships involving angles and triangles. They are crucial in various branches of mathematics and physics where trigonometric functions are used to model and analyze periodic phenomena, oscillatory motion, and waveforms.

What defines a trigonometry identity?

A trigonometry identity is an equality that holds true for all values of the variables involved. The trigonometric ratios of sine, cosine, tangent, secant, cosecant, and cotangent are the variables that are typically involved in trigonometry identities.

Trigonometry identities are derived from the definitions of the trigonometric ratios and the Pythagorean theorem. They can be used to simplify trigonometric expressions and to solve trigonometric equations.

Can you provide examples of fundamental trigonometric identities?

Here are some examples of fundamental trigonometric identities:

Pythagorean identity: sin^2(θ) + cos^2(θ) = 1
Reciprocal identities: sec(θ) = 1/cos(θ), csc(θ) = 1/sin(θ), cot(θ) = 1/tan(θ)
Quotient identities: tan(θ) = sin(θ)/cos(θ), cot(θ) = cos(θ)/sin(θ)
Angle addition and subtraction identities: sin(a + b) = sin(a)cos(b) + cos(a)sin(b)
Double angle identities: sin(2θ) = 2sin(θ)cos(θ), cos(2θ) = cos^2(θ) - sin^2(θ)

How do trigonometric identities contribute to solving mathematical problems?

Trigonometric identities can be used to solve a variety of mathematical problems, including:

Simplifying trigonometric expressions: Trigonometric identities can be used to simplify trigonometric expressions, which can make them easier to evaluate and understand.
Solving trigonometric equations: Trigonometric identities can be used to solve trigonometric equations, which are equations that involve trigonometric functions.
Proving geometric theorems: Trigonometric identities can be used to prove geometric theorems, such as the law of cosines and the law of sines.
Solving real-world problems: Trigonometric identities can be used to solve real-world problems that involve trigonometry, such as determining the distance to a star or the height of a building.

Here are some examples of how trigonometric identities can be used to solve mathematical problems:

Simplifying the expression sin(2θ) + cos(2θ): Using the double angle identity for sin(2θ) and cos(2θ), we can simplify the expression sin(2θ) + cos(2θ) to 2sin(θ)cos(θ) + cos^2(θ) - sin^2(θ).
Solving the equation sin(x) = cos(x): Using the Pythagorean identity, we can solve the equation sin(x) = cos(x) to get x = 45° or x = 315°.
Proving the law of cosines: The law of cosines states that c^2 = a^2 + b^2 - 2abcos(C). This theorem can be proven using trigonometric identities.
Solving a real-world problem: A surveyor needs to determine the distance to a tree that is located on the other side of a river. The surveyor measures the angle between the tree and the riverbank on both sides of the river. Using trigonometric identities, the surveyor can calculate the distance to the tree.

Trigonometric identities are a powerful tool that can be used to solve a variety of mathematical problems. By understanding and using trigonometric identities, you can simplify trigonometric expressions, solve trigonometric equations, prove geometric theorems, and solve real-world problems.