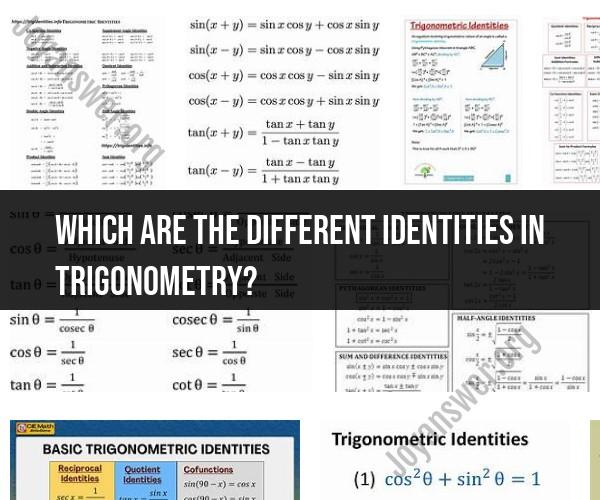
Trigonometry Identities: Fundamental Trig Equations

_{September 24, 2023 by JoyAnswer.org, Category : Mathematics}

Which are the different identities in trigonometry? Explore different trigonometric identities and equations used in trigonometry, including sine, cosine, and tangent identities.

Table of Contents

Which are the different identities in trigonometry?

Trigonometry identities are mathematical equations that relate the angles and side lengths of triangles. There are several fundamental trigonometric identities, including:

Pythagorean Identity:
- sin²(θ) + cos²(θ) = 1
- This identity relates the sine and cosine of an angle in a right triangle to the length of the hypotenuse.
Reciprocal Identities:
- sin(θ) = 1/csc(θ)
- cos(θ) = 1/sec(θ)
- tan(θ) = 1/cot(θ)
- These identities express the three primary trigonometric functions (sine, cosine, and tangent) in terms of their reciprocals (cosecant, secant, and cotangent).
Quotient Identities:
- tan(θ) = sin(θ) / cos(θ)
- cot(θ) = cos(θ) / sin(θ)
- These identities express the tangent and cotangent functions in terms of the sine and cosine functions.
Pythagorean Trigonometric Identities:
- sin²(θ) = 1 - cos²(θ)
- cos²(θ) = 1 - sin²(θ)
- These identities are derived from the Pythagorean theorem and relate the sine and cosine of an angle to each other.
Even and Odd Identities:
- sin(-θ) = -sin(θ)
- cos(-θ) = cos(θ)
- tan(-θ) = -tan(θ)
- These identities describe the symmetry properties of the trigonometric functions.
Cofunction Identities:
- sin(π/2 - θ) = cos(θ)
- cos(π/2 - θ) = sin(θ)
- tan(π/2 - θ) = 1/tan(θ)
- These identities relate the trigonometric functions of an angle to the functions of its complementary angle.
Double Angle Identities:
- sin(2θ) = 2sin(θ)cos(θ)
- cos(2θ) = cos²(θ) - sin²(θ)
- tan(2θ) = (2tan(θ)) / (1 - tan²(θ))
- These identities express the sine, cosine, and tangent of double angles in terms of the sine and cosine of the original angle.
Half-Angle Identities:
- sin(θ/2) = ±√[(1 - cos(θ)) / 2]
- cos(θ/2) = ±√[(1 + cos(θ)) / 2]
- tan(θ/2) = ±√[(1 - cos(θ)) / (1 + cos(θ))]
- These identities express the sine, cosine, and tangent of half angles in terms of the original angle's cosine.

These fundamental trigonometric identities are essential tools for solving trigonometric equations and simplifying trigonometric expressions. They play a crucial role in various branches of mathematics and science, including calculus, physics, and engineering.

Trigonometry Identities: A Comprehensive Guide to the Basics

Trigonometric identities are equations that relate the trigonometric functions of angles. They are true for all angles and can be used to simplify trigonometric expressions, solve trigonometric equations, and prove trigonometric theorems.

Some of the most common trigonometric identities include:

Pythagorean identities:
- sin²θ + cos²θ = 1
- tan²θ + 1 = sec²θ
- cot²θ + 1 = csc²θ
Angle addition and subtraction identities:
- sin(α + β) = sinα cosβ + cosα sinβ
- cos(α + β) = cosα cosβ - sinα sinβ
- sin(α - β) = sinα cosβ - cosα sinβ
- cos(α - β) = cosα cosβ + sinα sinβ

Angle doubling and halving identities:
- sin2θ = 2sinθ cosθ
- cos2θ = cos²θ - sin²θ = 1 - 2sin²θ
- tan2θ = sin²θ / cos²θ = sec²θ - 1

Mastering Trigonometric Identities: Key Concepts and Principles

There are a few key concepts and principles to keep in mind when using trigonometric identities:

Identities are true for all angles. This means that you can use identities to solve trigonometric problems involving any angle, acute, obtuse, or right.
Identities can be used to simplify trigonometric expressions. For example, you can use the Pythagorean identity sin²θ + cos²θ = 1 to simplify the expression sin²θ + cos²θ + sin²θ - cos²θ.
Identities can be used to solve trigonometric equations. For example, you can use the angle addition identity sin(α + β) = sinα cosβ + cosα sinβ to solve the equation sin(x + 30°) = 1/2.
Identities can be used to prove trigonometric theorems. For example, you can use the Pythagorean identity to prove the Law of Cosines.

Trigonometry Essentials: Understanding Different Identities

There are many different types of trigonometric identities, including:

Pythagorean identities: These identities are based on the Pythagorean theorem and relate the trigonometric functions of angles in a right triangle.
Angle addition and subtraction identities: These identities relate the trigonometric functions of the sum and difference of two angles.
Angle doubling and halving identities: These identities relate the trigonometric functions of a double and half angle.
Product-to-sum and sum-to-product identities: These identities relate the products and sums of trigonometric functions.
Negative angle identities: These identities relate the trigonometric functions of negative angles.

Each type of trigonometric identity has its own uses and applications. For example, the Pythagorean identities can be used to simplify trigonometric expressions and solve trigonometric equations involving right triangles. The angle addition and subtraction identities can be used to simplify trigonometric expressions and solve trigonometric equations involving angles in any position.

Trig identities can be a bit daunting at first, but with practice, you can learn to use them effectively to solve a wide variety of trigonometric problems.

_{Tags Trigonometry Identities , Fundamental Trig Equations , Trig Math Concepts}