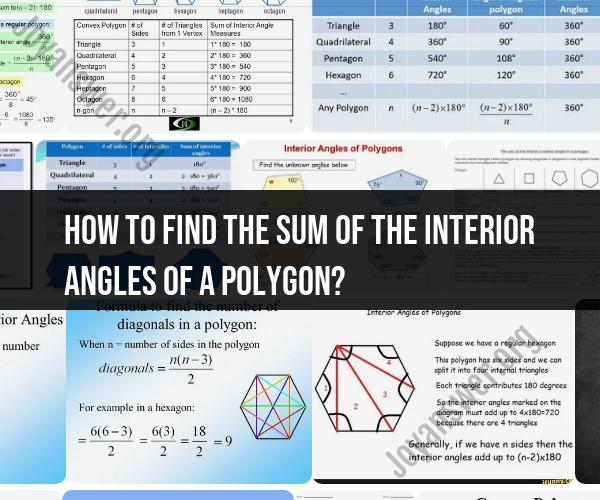
How to find the sum of the interior angles of a polygon?
Sum of Interior Angles of a Polygon: Polygonal Geometry
In polygonal geometry, the sum of the interior angles of a polygon can be calculated using the following formula:
Sum of Interior Angles = (n - 2) * 180 degrees
Where:
- n is the number of sides (or vertices) of the polygon.
This formula applies to both regular and irregular polygons, as long as you know the number of sides. Here are a few examples:
1. Triangle (n = 3):
Sum of Interior Angles = (3 - 2) * 180 = 1 * 180 = 180 degrees
2. Quadrilateral (n = 4):
Sum of Interior Angles = (4 - 2) * 180 = 2 * 180 = 360 degrees
3. Pentagon (n = 5):
Sum of Interior Angles = (5 - 2) * 180 = 3 * 180 = 540 degrees
This formula can be useful for finding the total measure of the interior angles of a polygon without needing to calculate each angle individually. It applies to polygons of any size, as long as the polygon is closed and has distinct vertices.