Solving Direct Variation Problems: Practical Techniques

_{October 9, 2023 by JoyAnswer.org, Category : Mathematics}

How to solve direct variation problems? Discover practical techniques for solving direct variation problems, including real-world mathematical applications.

Table of Contents

How to solve direct variation problems?

Solving direct variation problems involves finding the relationship between two variables and using that relationship to make predictions or solve for unknown values. Direct variation problems typically involve the equation $y = kx$ , where $y$ is the dependent variable, $x$ is the independent variable, and $k$ is the constant of proportionality. Here's a step-by-step approach to solving direct variation problems:

Identify the Variables: Determine which variables are involved in the problem and which one depends on the other. In direct variation problems, one variable will be directly proportional to the other.
Write Down the Direct Variation Equation: Express the relationship between the variables using the general form of a direct variation equation: $y = kx$ , where $k$ is the constant of proportionality. Make sure to label which variable is $y$ and which one is $x$ .
Find the Constant of Proportionality ( $k$ ):
- If you're given specific values for both $x$ and $y$ in the problem, you can calculate $k$ by rearranging the equation and solving for $k$ : $k = \frac{y}{x}$
- If you're not given specific values for $x$ and $y$ but are provided with other information (e.g., a description of the relationship between the variables), use that information to determine $k$ .
Use the Direct Variation Equation:
- If you need to find the value of $y$ for a given value of $x$ , simply substitute $x$ into the equation and solve for $y$ : $y = kx$
- If you need to find the value of $x$ for a given value of $y$ , rearrange the equation and solve for $x$ : $x = \frac{y}{k}$
Check for Units: Be mindful of units if applicable. Ensure that both $x$ and $y$ have consistent units, and use appropriate units in your final answer.
Solve the Problem: Apply the equation to the specific problem at hand. This might involve finding missing values, making predictions, or solving for unknowns based on the given information.
Check Your Solution: Always double-check your solution to ensure it makes sense in the context of the problem. Check for errors in calculations and verify that your answer aligns with the problem's requirements.
State Your Conclusion: Clearly state your answer and what it represents in the context of the problem. If applicable, include units in your answer.

Here's an example to illustrate the steps:

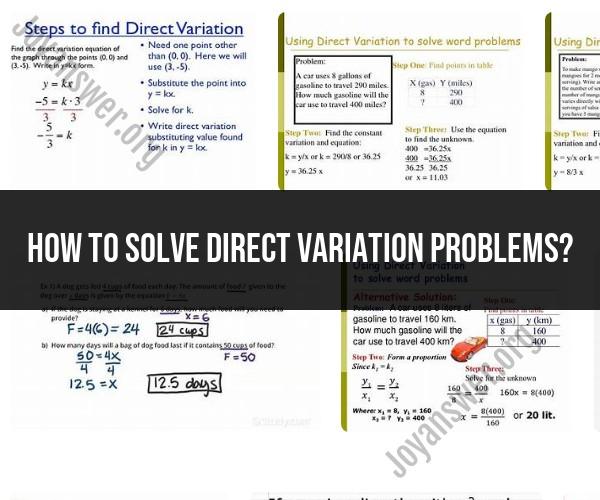
Problem: If the distance traveled ( $d$ ) is directly proportional to the time taken ( $t$ ), and a car travels 200 miles in 4 hours, find the constant of proportionality and the distance it travels in 6 hours.

Solution:

Identify the variables: $d$ (distance) depends on $t$ (time).
Write the direct variation equation: $d = kt$ .
Calculate the constant of proportionality ( $k$ ): $k = \frac{d}{t} = \frac{200 \text{ miles}}{4 \text{ hours}} = 50 \text{ miles/hour}$ .
Use the equation to find the distance traveled in 6 hours: $d = 50 \text{ miles/hour} \cdot 6 \text{ hours} = 300 \text{ miles}.$

So, the constant of proportionality ( $k$ ) is 50 miles/hour, and the car travels 300 miles in 6 hours.

Mastering Direct Variation Problems: Step-by-Step Solutions

To master direct variation problems, it is important to understand the basic concept of direct variation and the formula that represents it:

y = kx

where:

y is the dependent variable
x is the independent variable
k is the constant of variation

Here is a step-by-step guide to solving direct variation problems:

Identify the dependent and independent variables. The dependent variable is the variable that is being affected by the independent variable. The independent variable is the variable that is causing the change in the dependent variable.
Write down the direct variation equation. The direct variation equation is y = kx.
Substitute known values into the equation and solve for the unknown variable. If you are given two known values, you can substitute them into the equation and solve for the unknown variable. If you are given only one known value, you will need to find another known value before you can solve for the unknown variable.
Check your answer. Make sure that your answer makes sense in the context of the problem.

Problem-Solving with Direct Variation: Practical Examples and Tips

Here are some practical examples and tips for solving direct variation problems:

Example 1: A car travels 100 miles in 2 hours. How many miles will the car travel in 5 hours?

To solve this problem, we can use the following steps:

Identify the dependent and independent variables. The dependent variable is the distance traveled (y). The independent variable is the time spent traveling (x).
Write down the direct variation equation. The direct variation equation is y = kx.
Substitute known values into the equation and solve for the unknown variable. We know that when x = 2, y = 100. Substituting these values into the equation, we get:

100 = k(2)
k = 50

Now that we know the value of k, we can solve for the distance traveled in 5 hours:

y = kx
y = 50(5)
y = 250

Therefore, the car will travel 250 miles in 5 hours.

Tip: It is important to note that the constant of variation, k, is always positive. This is because direct variation relationships are always proportional, meaning that when one variable increases, the other variable increases proportionally.
Tip: If you are asked to find the constant of variation, k, you can simply substitute any two known values of x and y into the equation and solve for k.
Tip: If you are asked to find the value of a variable when the other variable is given, you can simply substitute the given value of x or y into the equation and solve for the unknown variable.

Navigating Mathematics with Direct Variation Relationships

Direct variation relationships are a common type of relationship in the real world. They are used in many different areas of mathematics, including algebra, geometry, and calculus.

Direct variation relationships can be used to model many different situations, such as:

The relationship between the distance traveled by a car and the time spent traveling.
The relationship between the amount of money earned and the number of hours worked.
The relationship between the weight of a bag of apples and the number of apples in the bag.

By understanding direct variation relationships and how to solve direct variation problems, you will be able to navigate many different mathematical situations with greater ease.

_{Tags Solving Direct Variation Problems , Problem-Solving Strategies , Mathematical Applications}