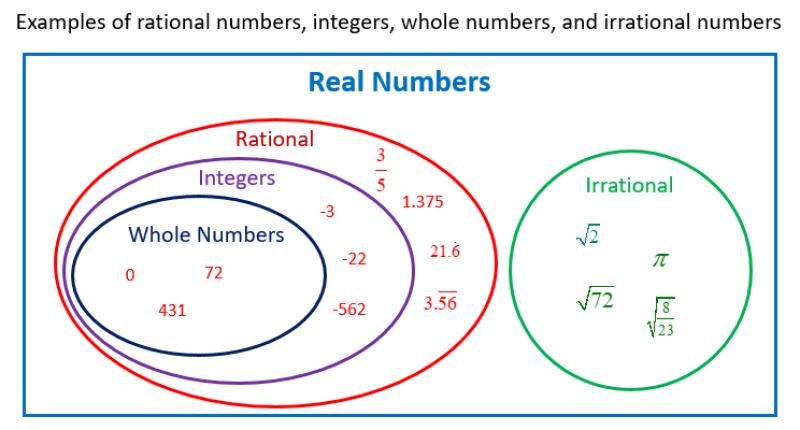
Rational vs. Irrational Numbers: How to Differentiate

_{November 12, 2023 by JoyAnswer.org, Category : Mathematics}

How to know whether a number is rational or irrational? Learn the methods to differentiate between rational and irrational numbers with this informative guide. Discover the key indicators that help determine whether a number is rational or irrational, enhancing your mathematical knowledge.

Table of Contents

1. How to know whether a number is rational or irrational?
2. How to determine whether a number is rational or irrational in mathematics?
3. What criteria or tests are used to identify and classify numbers as rational or irrational?
4. Are there real-world examples or applications illustrating rational and irrational numbers?

How to know whether a number is rational or irrational?

To differentiate between rational and irrational numbers, you can follow these key characteristics:

Rational Numbers:

Definition:
- Rational numbers are numbers that can be expressed as the ratio (fraction) of two integers, where the denominator is not zero.
Decimal Expansion:
- Rational numbers have decimal expansions that either terminate (end) or repeat.
Examples:
- Integers (e.g., -5, 0, 3), fractions (e.g., 2/3, -4/7), and terminating or repeating decimals (e.g., 0.25, 0.333...) are all examples of rational numbers.
Square Roots:
- The square root of a perfect square is rational. For example, the square root of 9 is 3, which is a rational number.
Addition and Subtraction:
- The sum or difference of two rational numbers is also a rational number.

Irrational Numbers:

Definition:
- Irrational numbers are numbers that cannot be expressed as the ratio of two integers. They have non-repeating, non-terminating decimal expansions.
Decimal Expansion:
- Irrational numbers have decimal expansions that do not terminate or repeat. The digits go on forever without forming a pattern.
Examples:
- The square root of a non-perfect square (e.g., √2, √5), transcendental numbers (e.g., π and $e$ ), and certain mathematical constants are examples of irrational numbers.
Square Roots:
- The square root of a non-perfect square is irrational. For example, the square root of 2 is irrational because 2 is not a perfect square.
Proofs by Contradiction:
- Some numbers are known to be irrational through mathematical proofs by contradiction. For instance, the square root of 2 is proven to be irrational.
Operations with Irrational Numbers:
- The sum, difference, product, or quotient of an irrational and a rational number is generally irrational. The same applies to operations between two irrational numbers.

In summary, if a number can be expressed as the ratio of two integers, has a terminating or repeating decimal expansion, or is the square root of a perfect square, it is rational. If a number has a non-repeating, non-terminating decimal expansion, is the square root of a non-perfect square, or is proven to be irrational, it is irrational.

How to determine whether a number is rational or irrational in mathematics?

There are two main ways to determine whether a number is rational or irrational in mathematics:

Check if the number can be expressed as a fraction of two integers. If it can, then it is a rational number. If it cannot, then it is an irrational number.
Check if the number has a non-repeating, non-terminating decimal expansion. If it does, then it is an irrational number. If it does not, then it is a rational number.

What criteria or tests are used to identify and classify numbers as rational or irrational?

The following criteria and tests can be used to identify and classify numbers as rational or irrational:

Rationality test: A number is rational if and only if it can be expressed as a fraction of two integers.
Irrationality test: A number is irrational if and only if it has a non-repeating, non-terminating decimal expansion.
Proof by contradiction: This method is used to prove that certain numbers are irrational. It involves assuming that the number is rational and then showing that this assumption leads to a contradiction.
Infinite series: Infinite series can be used to prove that certain numbers are irrational.
Continued fractions: Continued fractions can be used to approximate irrational numbers.

Are there real-world examples or applications illustrating rational and irrational numbers?

Rational numbers are very common in the real world. Some examples of rational numbers include:

The number of students in a class
The ratio of ingredients in a recipe
The distance between two cities
The price of a gallon of milk

Irrational numbers are less common in the real world, but they are still important in many areas of mathematics and science. Some examples of irrational numbers include:

The ratio of the circumference of a circle to its diameter (π)
The square root of two (√2)
The golden ratio (φ)
The number e

Here are some examples of real-world applications of rational and irrational numbers:

Rational numbers are used in many areas of engineering, such as designing bridges and buildings.
Rational numbers are also used in many areas of business, such as accounting and finance.
Irrational numbers are used in many areas of mathematics, such as calculus and trigonometry.
Irrational numbers are also used in many areas of science, such as physics and chemistry.