Linear vs. Quadratic Functions: Differentiation and Properties

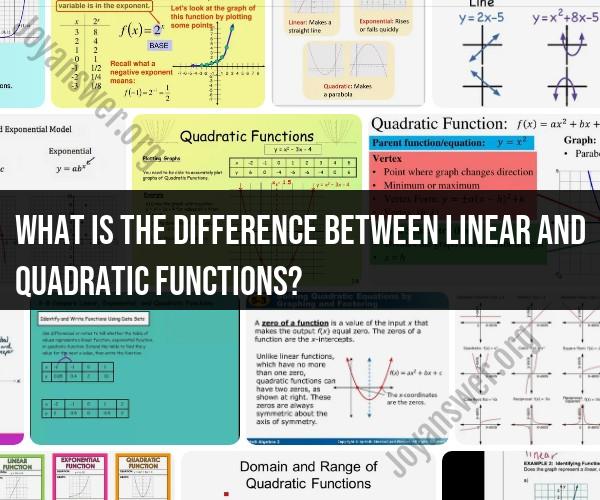
What is the difference between linear and quadratic functions?

Linear and quadratic functions are two fundamental types of mathematical functions that differ in their degree and properties. Here's a breakdown of the key differences between linear and quadratic functions:

1. Degree of the Function:

• Linear Function: A linear function has a degree of 1, which means it contains only variables raised to the first power. The general form of a linear function is $f(x) = ax + b$, where $a$ and $b$ are constants, and $x$ is the variable.
• Quadratic Function: A quadratic function has a degree of 2, which means it contains variables raised to the second power. The general form of a quadratic function is $f(x) = ax^2 + bx + c$, where $a$, $b$, and $c$ are constants, and $x$ is the variable.

2. Shape of the Graph:

• Linear Function: The graph of a linear function is a straight line. It has a constant slope, and the relationship between the dependent variable and the independent variable is always proportional.
• Quadratic Function: The graph of a quadratic function is a parabola. It has a curved shape, and the relationship between the dependent variable and the independent variable is nonlinear.

3. Rate of Change:

• Linear Function: In a linear function, the rate of change (slope) is constant. For every unit change in the independent variable, there is a constant change in the dependent variable.
• Quadratic Function: In a quadratic function, the rate of change is not constant. It varies with the value of the independent variable, leading to a curve in the graph.

4. Characteristics of the Graph:

• Linear Function: The graph of a linear function passes through the origin (0,0) if the constant term ($b$) is zero. Otherwise, it is a straight line with a slope determined by the coefficient $a$.
• Quadratic Function: The graph of a quadratic function does not necessarily pass through the origin. It has a vertex, which is the minimum or maximum point of the parabola, depending on the sign of the leading coefficient ($a$). The axis of symmetry is a vertical line that passes through the vertex.

5. Examples:

• Linear Function Example: $f(x) = 2x + 3$
  • This is a linear function with a slope of 2 and a y-intercept of 3. Its graph is a straight line.
• Quadratic Function Example: $f(x) = x^2 - 4x + 4$
  • This is a quadratic function with a vertex at (2,0). Its graph is a parabola opening upward.

In summary, the primary differences between linear and quadratic functions lie in their degree, graph shapes, rate of change, and characteristics. Linear functions produce straight-line graphs with constant slopes, while quadratic functions produce parabolic graphs with nonlinear relationships between variables.