Finding Amplitude of Trigonometric Functions: Measurement Technique

_{November 27, 2023 by JoyAnswer.org, Category : Mathematics}

How to find the amplitude of a trigonometric function? Learn how to determine the amplitude of trigonometric functions. This article explains the process and methods for amplitude calculation.

Table of Contents

How to find the amplitude of a trigonometric function?

To find the amplitude of a trigonometric function, you'll need to follow these steps:

For Sine and Cosine Functions:

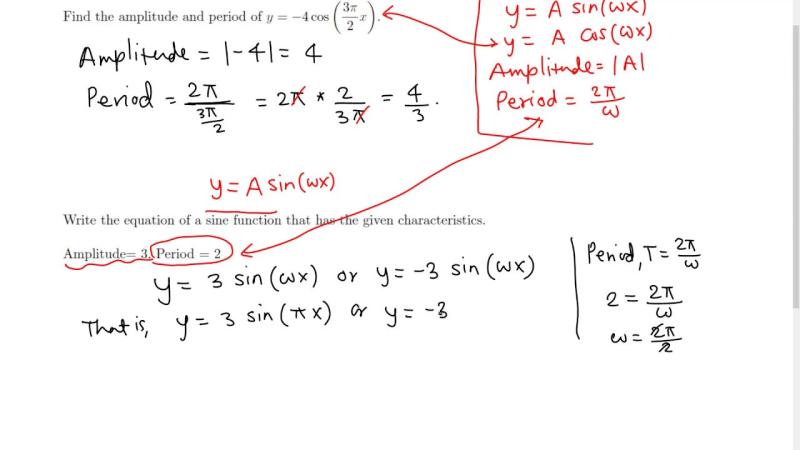
The amplitude of a sine or cosine function $f(x) = A \cdot \sin(Bx + C)$ or $g(x) = A \cdot \cos(Bx + C)$ is represented by the absolute value of $A$ .

Identify the Amplitude (A):
- For functions in the form $f(x) = A \cdot \sin(Bx + C)$ or $g(x) = A \cdot \cos(Bx + C)$ , the amplitude is the coefficient of the sine or cosine function, denoted as $A$ .
Calculate the Absolute Value of A:
- Regardless of the sign of $A$ , the amplitude is always its positive value.
- If $A$ is negative, take its absolute value to find the amplitude.

Example:

Consider the function $f(x) = 3 \cdot \sin(2x)$ .

Here, $A = 3$ . Thus, the amplitude of this function is $|A| = |3| = 3$ .

For cosine functions, the process is similar. Just extract the amplitude (the coefficient) and take its absolute value if needed.

Remember, the amplitude represents the maximum value the function reaches from its midline.

For Other Trigonometric Functions:

For other trigonometric functions such as tangent, cotangent, secant, and cosecant, they do not possess an amplitude in the same sense as sine and cosine functions. These functions have no maximum or minimum value within a bounded range and therefore do not exhibit an amplitude in the traditional sense. Instead, they have a periodic nature that follows their own unique properties.

If you encounter a function like $y = A \cdot \tan(Bx + C)$ , it doesn't have an amplitude in the way sine or cosine functions do. Instead, it has a periodic behavior with vertical asymptotes and doesn't have a fixed maximum or minimum value within its periodicity.

Amplitude of a Trigonometric Function

What is the amplitude of a trigonometric function?

The amplitude of a trigonometric function is the vertical distance between its midline and its maximum or minimum value. In other words, it is half the difference between the function's maximum and minimum values.

How to identify the amplitude of a sine or cosine function from its graph

The amplitude of a sine or cosine function can be identified by locating its maximum and minimum points and then measuring the distance between them and the midline.
The midline of a sine or cosine function is the horizontal line that passes exactly in the middle of its graph.
The maximum and minimum points of a sine or cosine function are the points where the graph reaches its highest and lowest values, respectively.

How to find the amplitude of a trigonometric function given its equation

The amplitude of a sine or cosine function can be found directly from its equation by looking for the absolute value of the coefficient multiplying the trigonometric term.
The absolute value of the coefficient represents the amplitude of the function, regardless of whether it is positive or negative.

For example, consider the sine function f(x) = 2sin(x). In this case, the amplitude is 2, as indicated by the absolute value of the coefficient 2.

What is the relationship between the amplitude and the graph of a trigonometric function

The amplitude of a trigonometric function determines the vertical stretch of its graph.
A larger amplitude results in a wider vertical oscillation of the graph, while a smaller amplitude results in a narrower vertical oscillation.
The midline of the graph remains unchanged, regardless of the amplitude.

How does the amplitude of a trigonometric function affect its real-world applications

The amplitude of a trigonometric function plays a crucial role in various real-world applications involving periodic phenomena.
In vibrating systems, the amplitude represents the maximum displacement from the equilibrium position.
In sound waves, the amplitude represents the maximum pressure variation, which determines the loudness of the sound.
In electrical signals, the amplitude represents the voltage difference, which is essential for signal transmission and processing.