Exploring Antiderivatives of Trigonometric Functions

_{October 15, 2023 by JoyAnswer.org, Category : Mathematics}

How to find antiderivatives of trig functions? Dive into the world of calculus and trigonometry by exploring how to find antiderivatives of trigonometric functions. This guide provides insights into the integration of trigonometric expressions.

Table of Contents

How to find antiderivatives of trig functions?

To find antiderivatives of trigonometric functions, you'll need to apply the rules of integration or the reverse of differentiation. The following are some common antiderivatives of trigonometric functions:

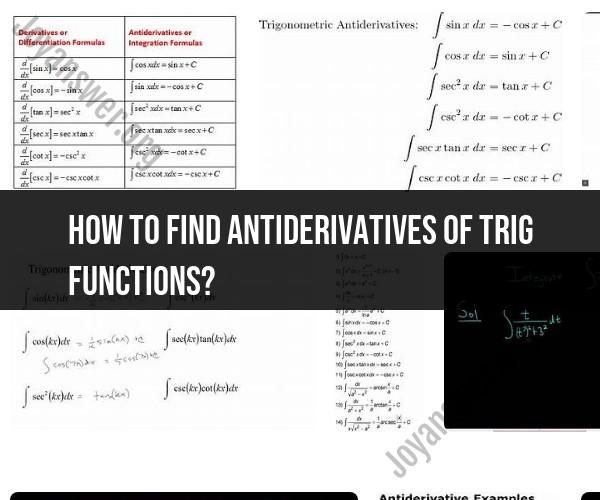
Antiderivative of sin(x):∫sin(x) dx = -cos(x) + C
Antiderivative of cos(x):∫cos(x) dx = sin(x) + C
Antiderivative of sec^2(x):∫sec^2(x) dx = tan(x) + C
Antiderivative of csc(x)cot(x):∫csc(x)cot(x) dx = -csc(x) + C
Antiderivative of sec(x)tan(x):∫sec(x)tan(x) dx = sec(x) + C
Antiderivative of csc(x):∫csc(x) dx = -ln|csc(x) + cot(x)| + C
Antiderivative of cot(x):∫cot(x) dx = -ln|sin(x)| + C
Antiderivative of sec(x):∫sec(x) dx = ln|sec(x) + tan(x)| + C
Antiderivative of sin^2(x) or cos^2(x):∫sin^2(x) dx = ∫(1 - cos^2(x)) dx = x - (1/2)sin(2x) + C
Antiderivative of tan(x):∫tan(x) dx = -ln|cos(x)| + C
Antiderivative of csc^2(x):∫csc^2(x) dx = -cot(x) + C

When finding antiderivatives of trigonometric functions, it's essential to remember the constant of integration, denoted as + C. This constant accounts for the possibility of multiple functions having the same derivative. You can also apply the linearity property of integration to antiderivatives involving sums or differences of trigonometric functions.

In some cases, you may need to use trigonometric identities to simplify the antiderivative before proceeding with the integration. Additionally, when dealing with more complex expressions or integrals, you might need to use techniques like integration by parts or substitution. Practice and familiarity with trigonometric identities and integration techniques will help you become proficient in finding antiderivatives of trig functions.

Finding Antiderivatives of Trigonometric Functions

Finding antiderivatives of trigonometric functions can be done using a variety of methods, including:

Using trigonometric identities: Many trigonometric antiderivatives can be found using trigonometric identities. For example, the antiderivative of $cos x$ is $sin x$ , and the antiderivative of $sin x$ is $- cos x$ .
Using u-substitution: U-substitution is a powerful technique for finding antiderivatives of a variety of functions, including trigonometric functions. For example, to find the antiderivative of $sec^{2} x$ , we can use the substitution $u = tan x$ .
Using integration by parts: Integration by parts is another powerful technique for finding antiderivatives of a variety of functions, including trigonometric functions. For example, to find the antiderivative of $sin x cos x$ , we can use integration by parts.

Key Trigonometric Antiderivative Formulas

Here are some key trigonometric antiderivative formulas:

$\int \sin x \, dx = -\cos x + C$

$\int cos x d x = sin x + C$

$\int sec^{2} x d x = tan x + C$

$\int csc^{2} x d x = - cot x + C$

$\int sec x tan x d x = sec x + C$

$\int csc x cot x d x = - csc x + C$

Integration Techniques for Trigonometric Expressions

In addition to the key trigonometric antiderivative formulas listed above, there are a number of other integration techniques that can be used to find the antiderivatives of trigonometric expressions. Some of these techniques include:

Integration by parts: Integration by parts can be used to find the antiderivatives of a variety of trigonometric expressions, such as $sin x cos x$ and $sec x tan x$ .
Double-angle identities: Double-angle identities can be used to find the antiderivatives of some trigonometric expressions, such as $sin^{2} x$ and $cos^{2} x$ .
Product-to-sum identities: Product-to-sum identities can be used to find the antiderivatives of some trigonometric expressions, such as $sin x cos x$ and $sec x tan x$ .

Applications of Trigonometric Antiderivatives in Calculus

Trigonometric antiderivatives have a variety of applications in calculus. For example, trigonometric antiderivatives can be used to:

Find the area under a trigonometric curve
Find the volume of a solid of revolution generated by rotating a trigonometric curve around an axis
Find the length of a trigonometric curve
Solve differential equations involving trigonometric functions
Model physical phenomena such as motion and vibration

Solving Complex Trigonometric Integration Problems

Solving complex trigonometric integration problems often requires a combination of different integration techniques. For example, to find the antiderivative of $sin^{2} x cos x$ , we can use integration by parts and then use a product-to-sum identity.

Another important skill for solving complex trigonometric integration problems is the ability to recognize and use trigonometric identities. For example, to find the antiderivative of $sec^{3} x$ , we can use the identity $sec^{3} x = sec x tan x sec x$ .

Conclusion

Finding antiderivatives of trigonometric functions can be challenging, but it is an important skill for calculus students. By understanding the key trigonometric antiderivative formulas and the various integration techniques available, students can solve a wide range of trigonometric integration problems.