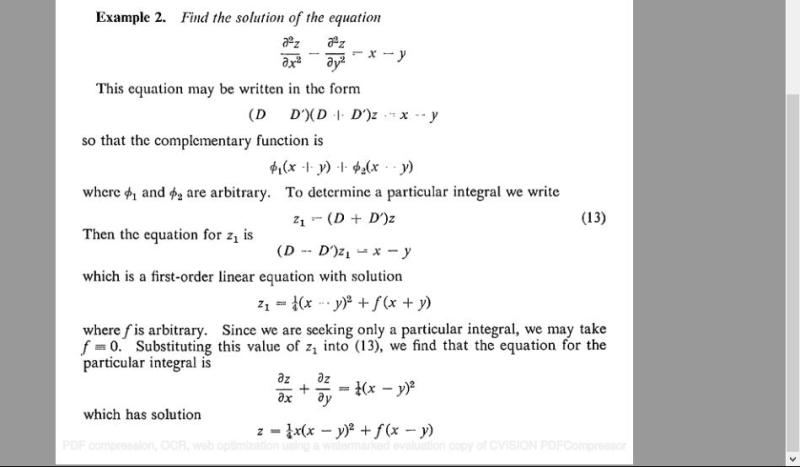
Example of First-Order Differential Equation: Mathematical Instance

_{November 24, 2023 by JoyAnswer.org, Category : Mathematics}

What is an example of a first order differential equation? An illustrative example showcasing a first-order differential equation, elucidating its form and application within mathematical contexts.

What is an example of a first order differential equation?

A first-order differential equation involves the first derivative of an unknown function with respect to the independent variable. The general form of a first-order linear ordinary differential equation (ODE) is often represented as:

$\frac{dy}{dx} = f(x, y)$

Here, $y$ is the dependent variable, $x$ is the independent variable, and $f(x, y)$ is a given function.

Let's consider a specific example of a first-order linear differential equation:

$\frac{dy}{dx} = 2x$

In this case, the rate of change of the function $y$ with respect to $x$ is given by $2x$ . To find the solution to this differential equation, you would integrate both sides with respect to $x$ :

$\int \frac{dy}{dx} \, dx = \int 2x \, dx$

The solution to this equation, after performing the integrations, would be:

$y(x) = x^2 + C$

Here, $C$ is the constant of integration, which would be determined by any initial conditions provided or through additional information about the problem.

So, the first-order differential equation $\frac{dy}{dx} = 2x$ has the solution $y(x) = x^2 + C$ .

Sure. Here is an example of a first-order differential equation:

Population growth model

The population of a city is modeled by the differential equation:

dP/dt = kP

where:

P(t) is the population of the city at time t

k is a constant growth rate

This equation states that the rate of change of the population (dP/dt) is proportional to the current population (P). The constant of proportionality k is the growth rate.

First-order differential equations are applied in a wide variety of fields, including: