Calculating the Slope Estimate: A Mathematical Guide

_{September 27, 2023 by JoyAnswer.org, Category : Mathematics}

How do you calculate slope estimate? Learn how to calculate the slope estimate, a fundamental concept in mathematics used to determine the steepness of a line or relationship between variables.

Table of Contents

How do you calculate slope estimate?

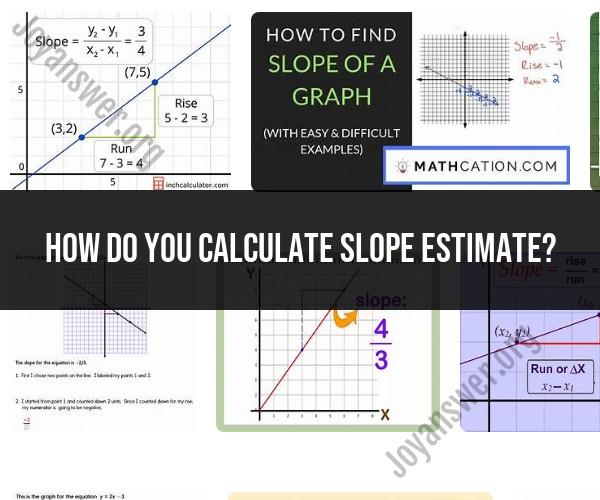
To calculate the slope estimate of a line, you need the coordinates of two points on that line. The formula for calculating the slope (often denoted as "m") is as follows:

Slope (m) = (Change in Vertical Position) / (Change in Horizontal Position)

Mathematically, it can be represented as:

m = (y2 - y1) / (x2 - x1)

Where:

(x1, y1) are the coordinates of the first point on the line.
(x2, y2) are the coordinates of the second point on the line.

Here's a step-by-step guide to calculating the slope estimate:

Identify the Two Points: Choose any two distinct points on the line for which you want to calculate the slope. Each point should have coordinates in the form (x, y).
Assign Coordinates: Label one point as (x1, y1) and the other point as (x2, y2). It doesn't matter which point you choose as (x1, y1) and which one as (x2, y2), as long as you're consistent.
Calculate the Change in Vertical Position: Subtract the y-coordinate of the first point (y1) from the y-coordinate of the second point (y2). This gives you the change in the vertical position (the rise).
Change in Vertical Position = y2 - y1
Calculate the Change in Horizontal Position: Subtract the x-coordinate of the first point (x1) from the x-coordinate of the second point (x2). This gives you the change in the horizontal position (the run).
Change in Horizontal Position = x2 - x1
Calculate the Slope: Divide the change in the vertical position (the rise) by the change in the horizontal position (the run). This gives you the slope of the line.
Slope (m) = (y2 - y1) / (x2 - x1)

The slope represents how steep the line is and the direction in which it is inclined. A positive slope indicates that the line rises from left to right, while a negative slope indicates that the line falls from left to right. A slope of zero indicates a horizontal line.

This formula is fundamental in geometry and algebra and is used to describe the relationship between two variables in various mathematical and scientific contexts, including linear equations, linear regression, and the slope-intercept form of a line equation (y = mx + b).

Estimating Slope: How to Calculate a Slope Estimate

The slope of a line is a measure of its steepness. It is calculated by dividing the change in the y-values by the change in the x-values.

For example, consider the following two points:

(1, 2)
(3, 4)

The slope of the line that passes through these two points is calculated as follows:

slope = (change in y) / (change in x)
slope = (4 - 2) / (3 - 1)
slope = 2 / 2
slope = 1

This means that the slope of the line is 1, which means that the line is increasing at a rate of 1 unit for every 1 unit increase in the x-value.

Calculating Slope in Data Analysis: Estimation Techniques

There are a number of different techniques that can be used to estimate the slope of a line in data analysis. One common technique is to use the least squares method.

The least squares method is a statistical method that finds the line that best fits a set of data points. The line that best fits the data is the line that minimizes the sum of the squared residuals. The residuals are the differences between the actual y-values and the predicted y-values for each data point.

Another common technique for estimating the slope of a line is to use a regression line. A regression line is a line that is fitted to a set of data points in order to predict the y-values for new x-values.

Statistical Slope Estimation: Methods and Applications

Statistical slope estimation is a powerful tool that can be used in a variety of applications. For example, it can be used to:

Predict future trends
Identify relationships between variables
Measure the effectiveness of interventions
Control for other variables in statistical analyses

Statistical slope estimation is used in a variety of fields, including economics, finance, medicine, and science.

Here are a few examples of how statistical slope estimation can be used in practice:

An economist might use statistical slope estimation to predict the future growth of the economy.
A financial analyst might use statistical slope estimation to predict the future performance of a stock.
A medical researcher might use statistical slope estimation to measure the effectiveness of a new drug.
A scientist might use statistical slope estimation to identify the relationship between two variables, such as temperature and climate change.

Statistical slope estimation is a valuable tool that can be used to gain insights into complex data.