Simplifying Expressions: Algebraic Techniques
September 1, 2023 by JoyAnswer.org, Category : Mathematics
What is the simplified form of expression? Learn algebraic techniques to simplify expressions effectively. This guide breaks down the process of simplifying expressions, offering examples and strategies for streamlining complex algebraic equations.
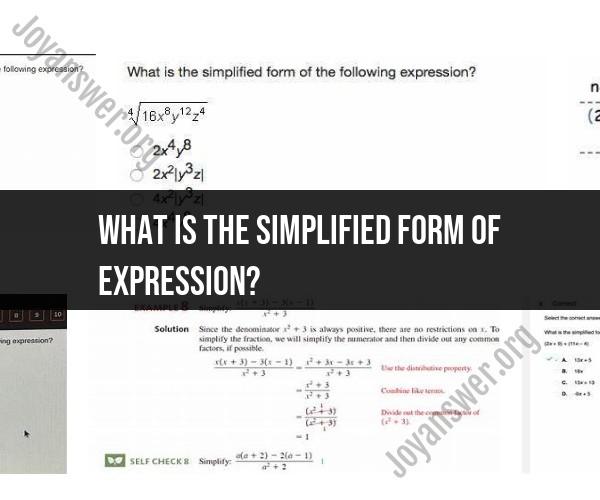
What is the simplified form of expression?
In algebra, simplifying an expression involves reducing it to its most concise and equivalent form while maintaining mathematical correctness. The simplified form of an expression typically involves combining like terms, reducing fractions, and removing unnecessary parentheses or symbols. The specific techniques for simplifying expressions depend on the nature of the expression and the rules of algebra.
Here are some common techniques for simplifying expressions:
Combine Like Terms: Combine terms that have the same variables and exponents. For example, in the expression 3x + 2x - 5x, you can combine the "x" terms to get (3 + 2 - 5)x, which simplifies to 0x or just 0.
Use the Distributive Property: Apply the distributive property to remove parentheses. For example, in the expression 2(x + 3), you can distribute the 2 to both terms inside the parentheses to get 2x + 6.
Simplify Fractions: If the expression contains fractions, simplify them by finding a common denominator and performing the necessary operations. For example, in the expression (4/6)x, you can simplify the fraction to (2/3)x.
Combine Exponents: If the expression contains exponential terms with the same base, you can combine their exponents using the rules of exponents. For example, in the expression x^2 * x^3, you can combine the exponents to get x^(2+3), which simplifies to x^5.
Factor: Factor out common factors if they exist. For example, in the expression 4x^2 + 8x, you can factor out a common factor of 4 to get 4(x^2 + 2x).
Cancel Common Factors: If there are common factors in both the numerator and denominator of a fraction, cancel them out. For example, in the expression (x^2 - 4)/(x^2 - 2x), you can cancel the common factor of (x^2 - 4) to simplify to (x + 2).
Use Identity and Zero Properties: Be aware of identity and zero properties. For instance, any number multiplied by 1 remains the same (identity property), and any number added to 0 remains the same (zero property).
Square Roots: Simplify square roots by factoring out perfect squares. For example, the square root of 12 can be simplified as 2√3 because 12 can be factored into 4 * 3, and the square root of 4 is 2.
Combine Radicals: When dealing with expressions involving square roots or other radicals, look for opportunities to combine them or rationalize the denominator.
The goal of simplification is to make the expression easier to work with, understand, and evaluate. It's an essential skill in algebra and is often used in solving equations, simplifying equations, and performing various mathematical operations.
