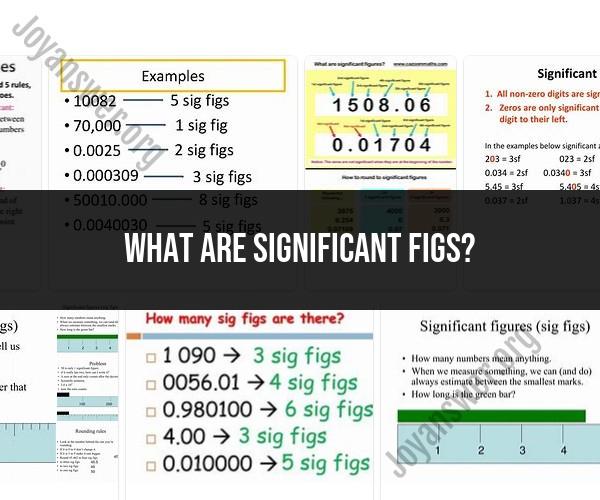
Navigating the World of Significant Figures

Significant figures (often abbreviated as "sig figs" or "SF") are a set of rules used in scientific and mathematical calculations to indicate the precision or reliability of measured or calculated numbers. Significant figures help ensure that the reported result reflects the uncertainty associated with the measurement or calculation. Here are the key principles of significant figures:

1. Non-zero Digits: All non-zero digits are considered significant. For example, in the number 1234, all four digits (1, 2, 3, and 4) are significant.

2. Leading Zeros: Leading zeros, which appear before any non-zero digit, are not considered significant. For example, in the number 0.0072, only the digits 7 and 2 are significant.

3. Captive Zeros: Captive zeros, which appear between non-zero digits, are considered significant. For example, in the number 1002, all four digits are significant.

4. Trailing Zeros: Trailing zeros, which appear after any non-zero digit, are considered significant only if they are to the right of the decimal point. For example, in the number 1200.00, all six digits are significant.

5. Exact Numbers: Exact numbers have an infinite number of significant figures. These are typically values that are defined without measurement uncertainty, such as exact integers (e.g., 12 eggs) or defined constants (e.g., the speed of light, which is precisely 299,792,458 meters per second).

The significance of significant figures lies in their use in calculations. When performing mathematical operations like addition, subtraction, multiplication, or division with numbers, it's essential to follow specific rules to ensure that the result has the correct number of significant figures. Here are some general rules:

• Addition and Subtraction: The result should have the same number of decimal places as the measurement with the fewest decimal places.

  Example: 5.67 + 2.1 = 7.77 (rounded to one decimal place)

• Multiplication and Division: The result should have the same number of significant figures as the measurement with the fewest significant figures.

  Example: 3.45 x 2.1 = 7.245 (rounded to three significant figures)

Significant figures are crucial in scientific experiments and calculations because they communicate the precision of the data and results. Using them correctly helps prevent overestimating the precision of your final answer and ensures that the level of uncertainty is appropriately conveyed.