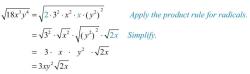How do you calculate powers?
Calculating powers is a fundamental mathematical skill that finds applications across various fields, from science to finance. Understanding the rules and methods for calculating powers can enhance your problem-solving abilities and boost your confidence in mathematical tasks. This article breaks down the concept of calculating powers and provides easy-to-follow methods for simplifying these calculations.
Introduction to Calculating Powers
A power, also known as an exponent or index, indicates the number of times a base number is multiplied by itself. In the expression "a^n," "a" is the base, and "n" is the exponent or power.
The Significance of Power Calculations
Power calculations are essential in various scenarios:
1. Scientific Notation
Scientific notation uses powers to express very large or small numbers in a concise form, making them easier to work with.
2. Compound Interest
In finance, powers are used to calculate compound interest, where the initial amount is raised to the power of the interest rate and the number of compounding periods.
3. Growth and Decay
Powers are used to model growth and decay in fields such as biology, economics, and population studies.
Simple Methods for Calculating Powers
1. Multiplying the Base
When the exponent is 2 or higher, you can calculate the power by multiplying the base by itself the specified number of times.
2. Using a Calculator
Modern calculators often have a dedicated key for exponentiation, allowing you to input the base and exponent directly.
3. Negative Exponents
A negative exponent indicates taking the reciprocal of the base raised to the positive exponent. For example, "a^-n = 1 / a^n."
Real-World Applications of Power Calculations
1. Population Growth
In biology, power calculations model population growth where the exponent represents the number of generations.
2. Interest Compounding
In finance, calculating powers is crucial for determining the growth of investments with compound interest over time.
3. Scientific Notation
Using powers in scientific notation simplifies working with extremely large or small numbers encountered in scientific research.
Benefits of Proficient Power Calculations
Mastering power calculations offers several advantages:
1. Efficient Problem Solving
Quickly calculating powers enhances your problem-solving efficiency in various mathematical scenarios.
2. Confidence in Mathematics
Understanding power calculations boosts your confidence in handling mathematical tasks and calculations.
3. Practical Application
Proficiency in power calculations empowers you to apply mathematical concepts to real-world situations.
Conclusion
Calculating powers is a foundational skill with widespread applications in diverse fields. By mastering power calculations, you equip yourself with a valuable tool for problem-solving, data analysis, and decision-making across various disciplines. Empower your math skills by embracing the simplicity and significance of power calculations.